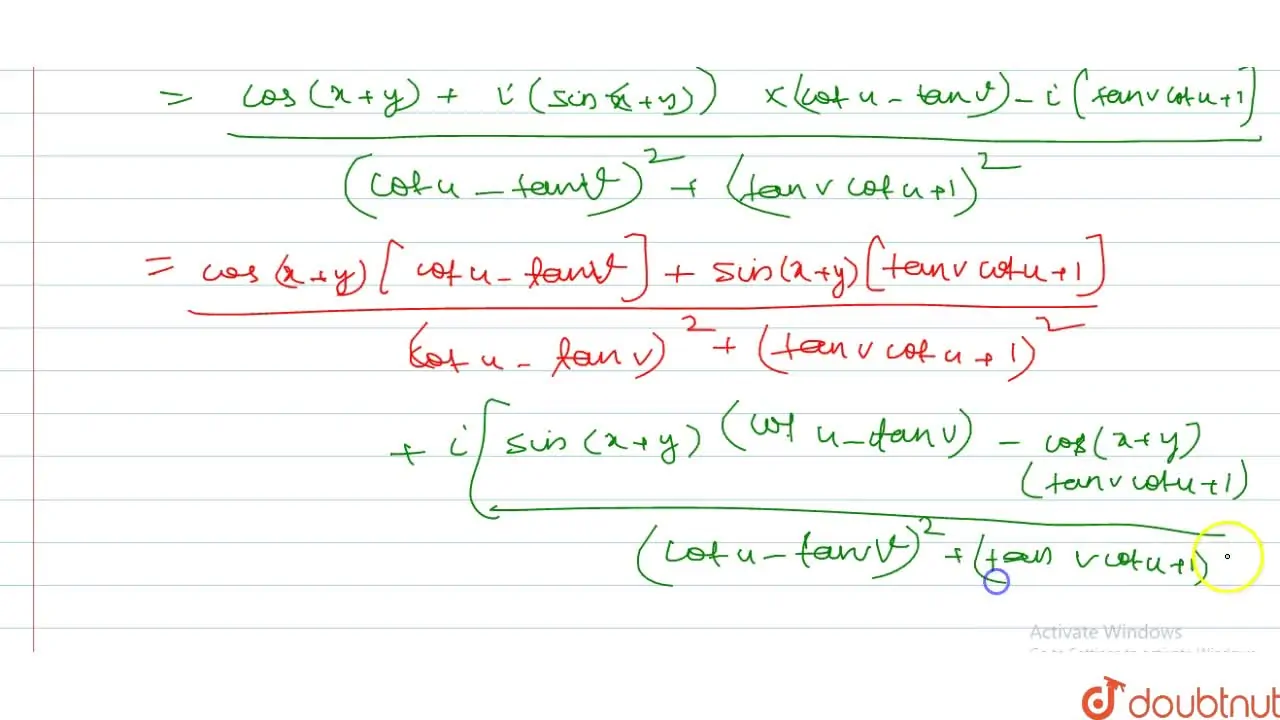
Put the following in the form A+iB:(cosx+isinx)(cosy+isiny)(cotu+i)(1+itanv)
Video Solution
|
Answer
Step by step video & image solution for Put the following in the form A+iB: ((cosx+isinx)(cosy+isiny))/((cotu+i)(1+itanv)) by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023
Similar Questions
Express the following in the form a+ib: (i−2)2
View SolutionPut the following numbers in the polar form: i
View SolutionExpress the following in the form a+ib: (5−3i)3
View SolutionExpress the following in the form a+ib: (1−i)4
View SolutionExpress the following in the form a+ib: (1+i)4
View SolutionExpress the following in the form a+ib:(1−i)4
View SolutionExpress the following in the form of a+ib:
(5−3i)3View SolutionExpress the following in the form of a+ib:
i−35View SolutionPut the following numbers in the polar form: −1+i
View SolutionExpress the following in the form a+ib:i−39
View Solution