Let and qhere , then
x(y+1)=1
y(1-x) = 1
y(x-1)=1
y(1+x) = 1
The correct Answer is:B
To solve the problem, we need to evaluate the sums x and y given by:
x=∞∑n=0(−1)n(tanθ)2n
y=∞∑n=0(cosθ)2n
where θ∈(0,π4).
Step 1: Evaluate x
The series for x is an alternating geometric series. The general form of an alternating geometric series is:
∞∑n=0(−1)nan=11+afor |a|<1
In our case, a=tan2θ. Since θ∈(0,π4), we have tanθ<1, thus tan2θ<1.
Using the formula for the sum of the series, we get:
x=11+tan2θ
Step 2: Simplify x
Using the trigonometric identity 1+tan2θ=sec2θ, we can rewrite x:
x=1sec2θ=cos2θ
Step 3: Evaluate y
The series for y is a standard geometric series:
y=∞∑n=0(cosθ)2n
The sum of a geometric series is given by:
∞∑n=0rn=11−rfor |r|<1
Here, r=cos2θ, and since θ∈(0,π4), we have cosθ<1 and thus cos2θ<1. Therefore:
y=11−cos2θ
Step 4: Simplify y
Using the identity 1−cos2θ=sin2θ, we can rewrite y:
y=1sin2θ
Step 5: Find x+1y
Now we need to compute x+1y:
1y=sin2θ
Thus,
x+1y=cos2θ+sin2θ
Using the Pythagorean identity:
cos2θ+sin2θ=1
Conclusion
We have found that:
x+1y=1
This leads us to the final result:
xy+1=y⟹y(1−x)=1
Thus, the answer is:
1
y=∞∑n=0(cosθ)2n
Similar Questions
Knowledge Check
If 0<θ,ϕ<π2,x=∞∑n=0cos2nθ,y=∞∑n=0sin2nϕ and z=∞∑n=0cos2nθ⋅sin2nϕ then :
Axy−z=(x+y)zBxy+yz+zx=zCxyz=4Dxy+z=(x+y)zIf x=∞∑n=0an,y=∞∑n=0bn,z=∞∑n=0(ab)n, where a,b<1, then
Axyz=x+y+zBxz+yz=xy+zCxy+yz=xz+yDxy+xz=yz+x
∞∑n=0(−1)nxn+1=
View SolutionIf 0<θ,ϕ<π2 and x=∞∑n=0sin2nθ,y=∞∑n=0cos2nϕ and z=∞∑n=0cosn(θ+ϕ)cosn(θ−ϕ), then
View SolutionIf x=∞∑n=0(Cosθ)2n,y=∞∑n=0(Sinϕ)2n,z=∞∑n=0(Cosϕ)2n.(Sinθ)2n Then which of the following is true ??
View SolutionFor 0<ϕ≤π2, if x=∞∑n=0cos2nϕ,y=∞∑n=0sin2nϕ,z=∞∑n=0cos2nϕsin2nϕ, then
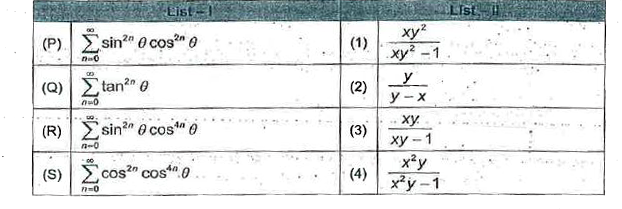
View SolutionFor 0<θ<π4, let x=∞∑n=0sin2nθ,y=∞∑n=0cos2nθ, then match the entries of column-I with column-II
Column-IColumn-II(a)∑∞0sin2nθcos2nθ(p)xy2xy2−1(b)∑∞0tan2nθ(q)yy−x(c)∑∞0sin2nθcos4nθ(r)xyxy−1(d)∑∞0cos2nθsin4nθ(s)x2yx2y−1
View Solution∞∑n=0(2i3)n=
View Solution