Electric field in space is given by −−→E(t)=E0i+j√2cos(ωt+Kz). A positively charged particle at (0,0,πK) is given velocity v0ˆk at t = 0. Direction of force acting on particle is
f = 0
antiparallel to ˆi+ˆj√2
parallel to ˆi+ˆj√2
ˆk
The correct Answer is:B
To solve the problem, we need to determine the direction of the force acting on a positively charged particle in an electric field given by:
→E(t)=E0(i+j)√2cos(ωt+kz)
The particle is initially located at (0,0,πk) and has an initial velocity of →v=v0^k at t=0.
Step 1: Calculate the Electric Field at t=0
First, we need to find the electric field at the position of the particle when t=0:
→E(0)=E0(i+j)√2cos(0+k⋅πk)
This simplifies to:
→E(0)=E0(i+j)√2cos(π)
Since cos(π)=−1, we have:
→E(0)=−E0(i+j)√2
Step 2: Determine the Direction of the Electric Field
The direction of the electric field →E(0) is:
→E(0)=−E0√2(i+j)
This indicates that the electric field is directed in the opposite direction of the vector (i+j).
Step 3: Calculate the Magnetic Field Direction
The electromagnetic wave propagates in the −z direction (as indicated by the cos(ωt+kz) term). The direction of the magnetic field →B can be determined using the right-hand rule, where →E and →B are perpendicular to the direction of wave propagation.
Using the relation:
→E×→B∝^k
We can find the direction of →B:
1. The electric field →E points in the direction of −E0√2(i+j).
2. The wave propagates in the −z direction, so we can use the right-hand rule to find →B.
Step 4: Calculate the Magnetic Force on the Particle
The force acting on a charged particle in an electric field is given by:
→FE=q→E
The magnetic force is given by:
→FB=q→v×→B
Step 5: Determine the Total Force Direction
The total force on the particle is:
→F=→FE+→FB
1. Calculate →FE using the electric field we found.
2. Calculate →FB using the velocity →v=v0^k and the direction of →B we determined.
Conclusion: Direction of the Force
The direction of the total force →F will be the vector sum of →FE and →FB. Since both forces are vectors, we can find their resultant direction.
2. The wave propagates in the −z direction, so we can use the right-hand rule to find →B.
2. Calculate →FB using the velocity →v=v0^k and the direction of →B we determined.
Topper's Solved these Questions
Similar Questions
The space has electromagnetic field which is given as →B=−B0ˆk and →E=E0ˆk. A charged particle having mass m and positive charge q is given velocity v0ˆi at origin at t=0sec. The z coordinate of the particle when it passes through the z−axis is (neglect gravity through motion)
View SolutionElectric field and magnetic field in a region of space are given by(Ē= E_0 hat j). and (¯¯¯B=B0ˆj). A charge particle is released at origin with velocity (¯v=v0ˆk) then path of particle is
View Solution
Knowledge Check
The electric field in space is given by →E=E0sin(ωt+6y−8z)ˆn then the direction of propagation of light wave is
A−3ˆj−4ˆk5B−3ˆj+4ˆk5C−3ˆi−4ˆk5D3ˆi−4ˆj5Electric field of an electromagnetic wave →E=E0cos(ωt−kx)ˆj . The equation of corresponding magnetic field at t=0 should be
A→B=E0√μ0ε0coskxˆkB→B=(E0√μ0ε0)coskxˆkC→B=E0√μ0ε0coskx(−ˆk)D→B=E0√μ0ε0coskx(−ˆk)
A positively charged particle of mass m and charge q is projected on a rough horizontal x-y plane surface with z-axis in the vertically upward direction. Both electric and magnetic fields are acting in the region and given by →E=−E0ˆkand→B=−B0ˆk , respectively. The particle enters into the field at (a0,0,0) with velocity →v=v0ˆj. The particle starts moving in some curved path on the plane. If the coefficient of friction between the particle and the plane is μ. Then calculate the
(a) time when the particle will come to rest
(b) distance travelled by the particle when it comes to rest.

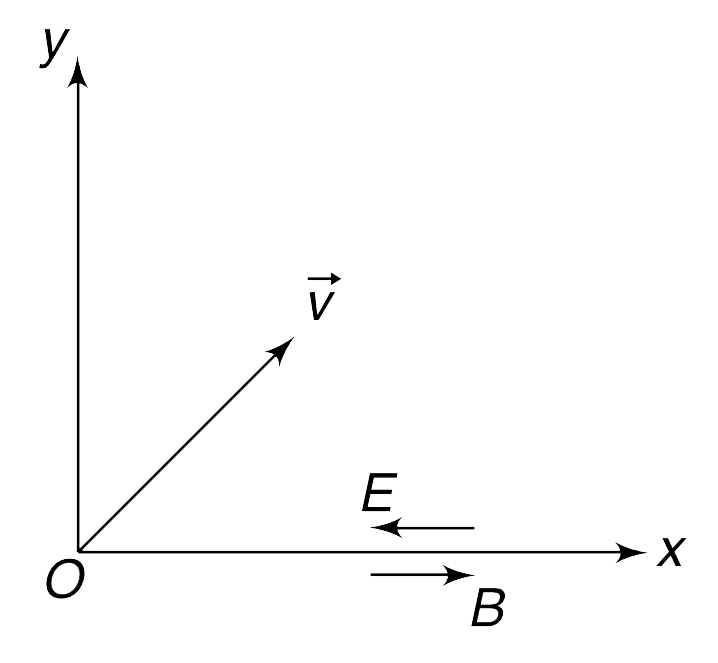
View SolutionA region has a uniform magnetic field B along positive x direction and a uniform electric field E in negative x direction. A positively charged particle is projected from origin with a velocity −−→V=V0ˆi+V0ˆj . After some time the velocity of the particle was observed to beV0ˆJ while its x co-ordinate was positive. Write all possible values of EB
View SolutionThere exists a uniform magnetic field →B=+B0ˆk for x>0and→B=0 for all x<0. A charged particle placed at (0,−a,0) is given an initial velocity →v=v0ˆi. What is the magnitude and nature of charge on the particle such that it crosses through the origin ?
Strategy: When the charge is projected perpendicular to a uniform magnetic field, it follows a circular path. In this case, the force acting on it will be directed either towards +y-axis. or y-axis. It is given that it crosses point O. Thus, the force at (0,−a,0) must be towards y-axis
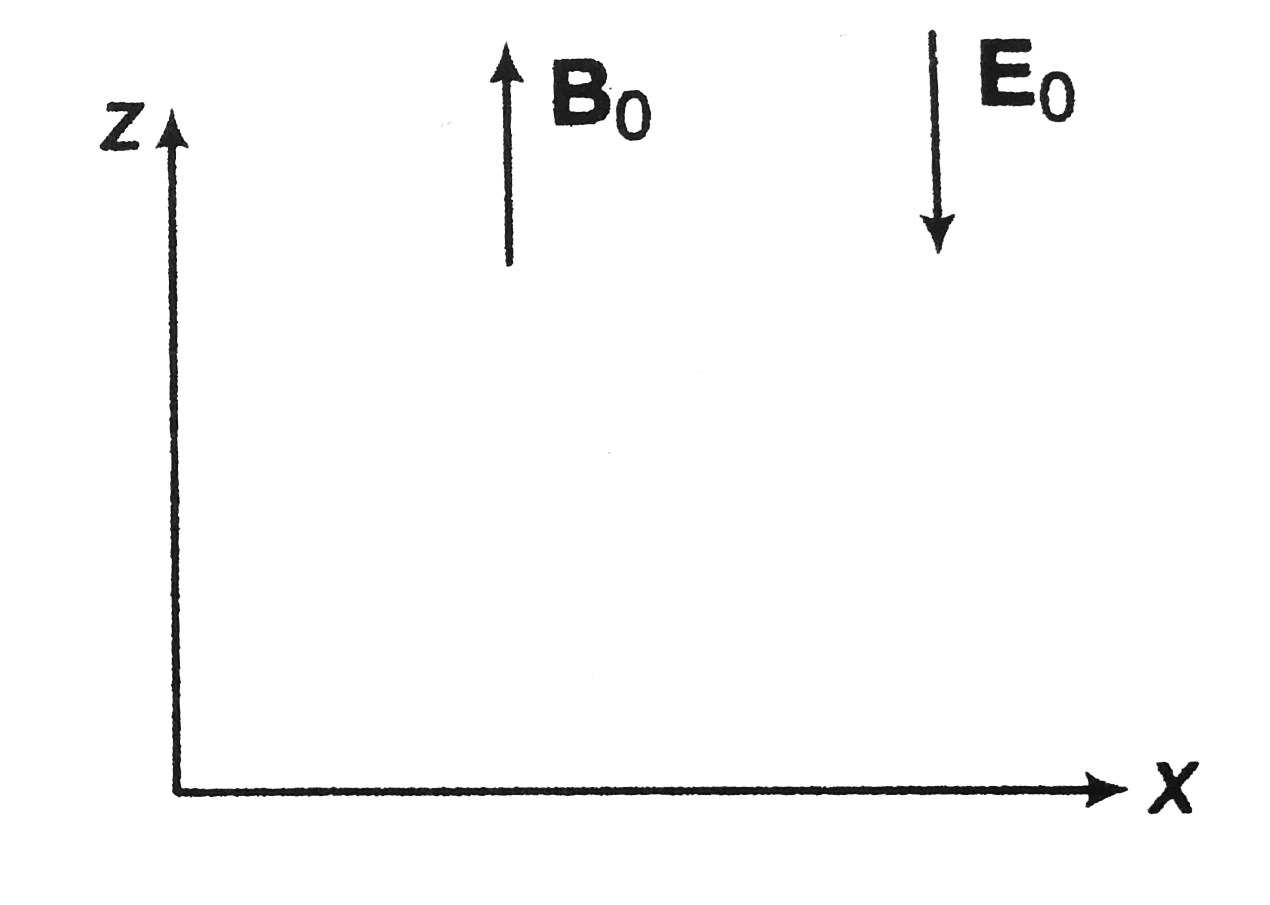
View SolutionIn a certain region, uniform electric field E=−E0ˆk and magnetic field B=B0ˆk are present. At time t=0, a particle of mass in and charge q is given a velocity v=v−0ˆj+v−0ˆk. Find the minimum speed of the particle and the time when it happens so.
View SolutionA charged particle of mass m and charge q is projected on a rough horizontally xy− plane surface with z− axis in the vertically upward direction. Both electric and magnetic fields are acting in the region and given by →E=−E0ˆk and →B=−B0ˆk respectively. The particle enters into the field at (a,0,0) with velocity →v=υ0ˆj. The particle starts moving into a circular path on the plane. If the coefficient of friction between the particle and the plane is μ. Then calculate tha :

(a) time when the particle will come to rest
(b) time when the particle will hit the centre.
(c) distance travelled by the particle when it comes to rest.
View SolutionAcceleration of a particle is given by
a=1/V
Where V is the velocity . Find
(i) Velocity of particle at time t, given that v = 10 m/s at t = 0.
(ii) Velocity of particle when its position is x, given that speed is 3 m/s at x = 0.
View Solution
