A rod of mass M and length L is placed on a smooth horizontal surface. It is hinged at one end so that it can rotate freely in a horizontal circle about this end. There exists a uniform magnetic field →B perpendicular, into the horizontal surface everywhere. The strength of magnetic field starts varying as B=B0t.
(i) Find the torque acting on the rod about its hinged end.
(ii) Find the reaction produced at hinge at t = 0.
(A charge Q is distributed uniformaly on the rod)
To solve the problem, we will break it down into two parts as stated in the question.
Part (i): Finding the Torque Acting on the Rod
1. Understanding the Setup:
- We have a rod of mass M and length L hinged at one end.
- The magnetic field →B varies with time as B=B0t.
- The magnetic field is perpendicular to the plane of rotation of the rod.
2. Charge Distribution:
- The charge Q is uniformly distributed along the length of the rod. Therefore, the linear charge density λ is given by:
λ=QL
3. Induced EMF:
- As the magnetic field changes with time, an electromotive force (EMF) is induced in the rod. The induced EMF E can be calculated using Faraday's law of electromagnetic induction:
E=−dΦdt
- The magnetic flux Φ through the area swept by the rod is given by:
Φ=B⋅A=B⋅12L⋅L=12BL2
- Substituting B=B0t:
Φ=12B0tL2
- Therefore, the induced EMF is:
E=−ddt(12B0tL2)=−12B0L2
4. Current in the Rod:
- The induced EMF causes a current I to flow through the rod, given by:
I=ER=−12B0L2R
- Here, R is the resistance of the rod.
5. Magnetic Force on the Rod:
- The magnetic force F acting on a segment dx of the rod at a distance x from the hinge is given by:
dF=I⋅B⋅dx=⎛⎝−12B0L2R⎞⎠⋅(B0t)⋅dx
- Integrating this from 0 to L gives the total force F:
F=∫L0⎛⎝−12B0L2R⎞⎠⋅(B0t)dx=−12B20L2tR⋅L=−12B20L3tR
6. Torque Calculation:
- The torque τ about the hinge is given by:
τ=F⋅L2=−12B20L3tR⋅L2=−14B20L4tR
Part (ii): Finding the Reaction Produced at Hinge at t=0
1. At t=0:
- At t=0, the magnetic field B=B0⋅0=0, hence the induced EMF and current will also be zero.
- Therefore, the torque acting on the rod will also be zero:
τ=0
2. Reaction Force at Hinge:
- The only forces acting on the rod at t=0 are the gravitational force Mg acting downwards and the normal force N at the hinge acting upwards.
- Since the rod is in equilibrium at this moment, the reaction force N at the hinge must balance the weight of the rod:
N=Mg
Final Answers:
(i) The torque acting on the rod about its hinged end is:
τ=−14B20L4tR
(ii) The reaction produced at the hinge at t=0 is:
N=Mg
- We have a rod of mass M and length L hinged at one end.
- The magnetic field →B varies with time as B=B0t.
- The magnetic field is perpendicular to the plane of rotation of the rod.
- The charge Q is uniformly distributed along the length of the rod. Therefore, the linear charge density λ is given by:
λ=QL
- As the magnetic field changes with time, an electromotive force (EMF) is induced in the rod. The induced EMF E can be calculated using Faraday's law of electromagnetic induction:
E=−dΦdt
- The magnetic flux Φ through the area swept by the rod is given by:
Φ=B⋅A=B⋅12L⋅L=12BL2
- Substituting B=B0t:
Φ=12B0tL2
- Therefore, the induced EMF is:
E=−ddt(12B0tL2)=−12B0L2
- The induced EMF causes a current I to flow through the rod, given by:
I=ER=−12B0L2R
- Here, R is the resistance of the rod.
- The magnetic force F acting on a segment dx of the rod at a distance x from the hinge is given by:
dF=I⋅B⋅dx=⎛⎝−12B0L2R⎞⎠⋅(B0t)⋅dx
- Integrating this from 0 to L gives the total force F:
F=∫L0⎛⎝−12B0L2R⎞⎠⋅(B0t)dx=−12B20L2tR⋅L=−12B20L3tR
- The torque τ about the hinge is given by:
τ=F⋅L2=−12B20L3tR⋅L2=−14B20L4tR
- At t=0, the magnetic field B=B0⋅0=0, hence the induced EMF and current will also be zero.
- Therefore, the torque acting on the rod will also be zero:
τ=0
- The only forces acting on the rod at t=0 are the gravitational force Mg acting downwards and the normal force N at the hinge acting upwards.
- Since the rod is in equilibrium at this moment, the reaction force N at the hinge must balance the weight of the rod:
N=Mg
(i) The torque acting on the rod about its hinged end is:
τ=−14B20L4tR
N=Mg
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH INSTITUTECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Assignment (SECTION - J)
4 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH INSTITUTECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EXERCISE
17 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH INSTITUTECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Assignment (SECTION - H)
3 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - AAKASH INSTITUTECHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - SECTION -J(Aakash Challengers Questions)
5 Videos
- ELECTROMAGNETIC WAVESBOOK - AAKASH INSTITUTECHAPTER - ELECTROMAGNETIC WAVESEXERCISE - ASSIGNMENT SECTION - D Assertion-Reason Type Questions
25 Videos
Similar Questions
A uniform rod AB of mass m and length l is at rest on a smooth horizontal surface. An impulse J is applied to the end B perpendicular to the rod in horizontal direction. Speed of the point A of the rod after giving impulse is:
View SolutionA straight rod AB of mass M and length L is placed on a frictionless horizontal surface. A horizontal force having contstant magnitude F and fixed direction starts acting at the end A. The rod is initially perpendicular to the force. Find the initial acceleration of end B.
View Solution
Knowledge Check
A uniform chain of mass M and length L is rotated in a horizontal circle about its one end at an angular frequency ω. The tension at its axis is
AMω2LBMω2L8CMω2L4DMω2L2A uniform rod of mass m and length l is at rest on smooth horizontal surface. An impulse P is applied to end B as shown.
The point about which rod rotates just after impulse being applied is located atAl3 from ABl6 from ACl2 from ADl4 from AA uniform rod of mass m and length l is at rest on smooth horizontal surface. An impulse P is applied to end B as shown.

Distance travelled by the centre of the rod, while rod rotates by 90∘Aπl7Bπl4Cπl12Dπl5
A rod AB of mass M and length L is shown in figure. End A of rod is hinged and end B is lying on the ground. Find the

Horizontal component of the force applied by the hinge
View SolutionA rod AB of mass M and length L is shown in figure. End A of rod is hinged and end B is lying on the ground. Find the
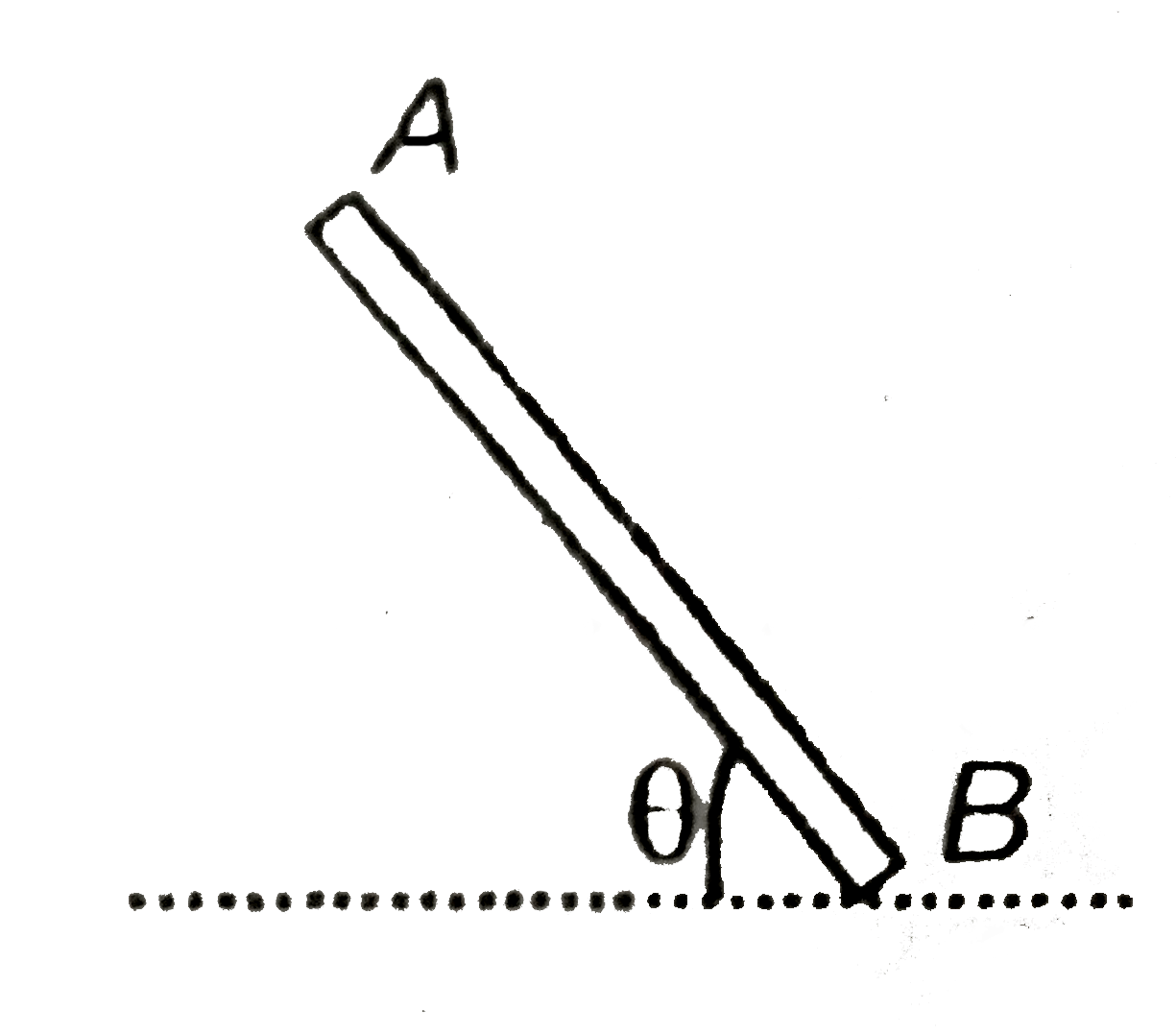
Normal reaction by ground on the rod
View SolutionA uniform rod of mass m and length l is on the smooth horizontal surface. When a constant force F is applied at one end of the rod for a small time t as shown in the figure. Find the angular velocity of the rod about its centre of mass.
View SolutionA uniform rod of length L and mass M is hinged at its upper point and is at rest at that moment in the vertical plane.A current i flows in it.A uniform magnetic field of strength B exists perpendicular to the rod and in horizontal direction is iLBN.Then find the value of N.
View SolutionA thin uniform rod AB of mass m and length L is placed on a smooth horizontal table. A constant horizontal force of magnitude F starts acting on the rod at one of the ends AB, Initially, the force is perpendicular to the length of the rod. Taking the moment at which force starts acting as t=0, find.
(a) Distance moved by centre of mass of the rod in time t0.
(b) Magnitude of initial acceleration of the end A of the rod.
View Solution
