A balloon starts rising from the earth's surface. The ascension rate is constant and equal to $v_{0}$ . Due to the wind. The balloon gathers the horizontal velocity component $v_{x} = k y$ , where k is a constnat and y is the height of ascent. Find how the following quantities depednd on the height of ascent.
(a) the horizontal drift of the balloon x (y)
(b) the total tangential and normal accelrations of the balloon.

Video Solution

Text Solution

Verified by Experts

The correct Answer is:(i) $x = \frac{a y^{2}}{2 v_{0}}$ (ii) $a_{total} = a v_{0} a_{tangential} = \frac{v_{0} a^{2} y}{\sqrt{v_{0}^{2} + a^{2} y^{2}}}, a_{normal} = \frac{a v_{0}^{2}}{{\sqrt{v}}_{0}^{2} + a^{2} y^{2}}$

Updated on:21/07/2023

Answer

Step by step video, text & image solution for A balloon starts rising from the earth's surface. The ascension rate is constant and equal to v_(0). Due to the wind. The balloon gathers the horizontal velocity component v_(x)=ky, where k is a constnat and y is the height of ascent. Find how the following quantities depednd on the height of ascent. (a) the horizontal drift of the balloon x (y) (b) the total tangential and normal accelrations of the balloon. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 PHYSICS MOTION IN A PLANE

Topper's Solved these Questions

Knowledge Check

A balloon starts rising from the ground with an acceleration of $1.25 m s^{- 2}$ . After 8 seconds, a stone is released from the balloon. After releasing, the stone will:
Acover a distance of 50 m till it strikes the ground.
Bhave a displacement of 50 till it reaches the ground.
Creach the ground in 4 seconds.
Dbegin to move down instantaneously.
Two ballons are simultaneously released from two buildings A and B. Balloon from A rises with constant velocity $10 m s^{- 1}$ While the other one rises with constant velocity of $20 m s^{- 1}$ Due to wind the balloons gather horizontal velocity $V_{x} = 0.5$ y, where 'y' is the height from the point of release. The buildings are at a distance of $250$ m & after some time 't' the balloons collide.

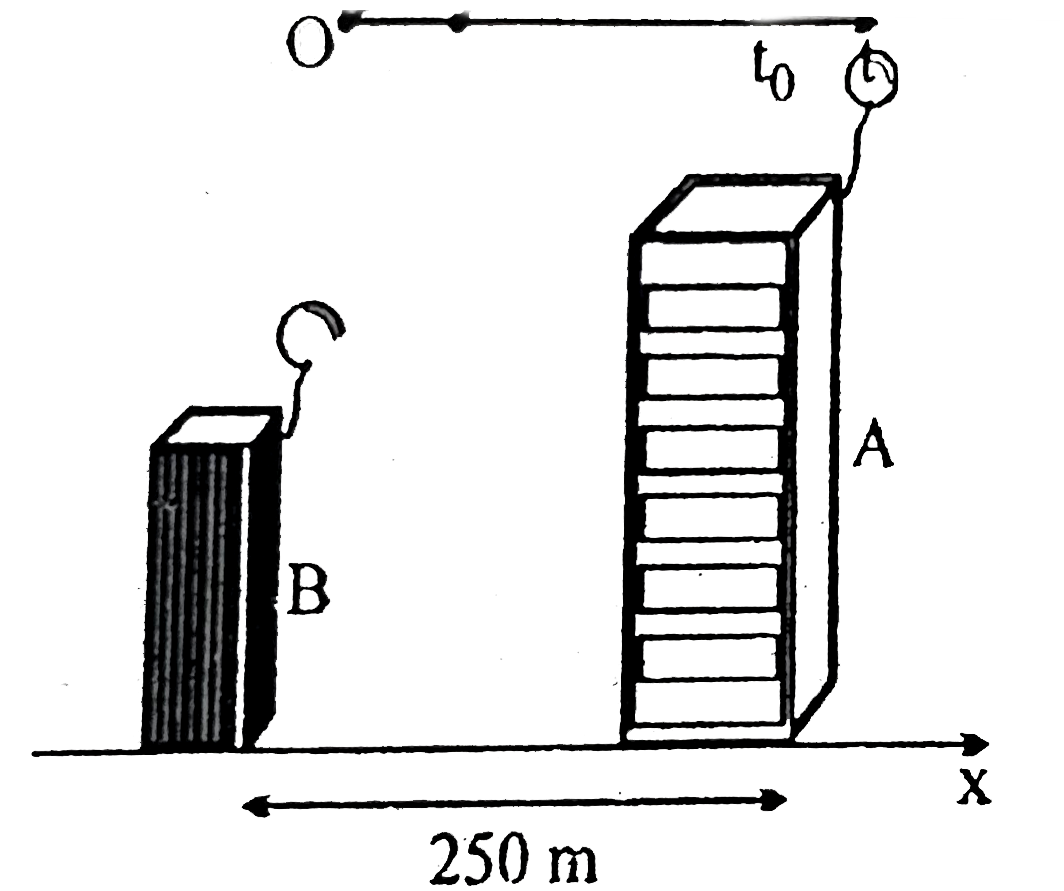A $t = 5$ sec
Bdifference in height of buildings in 100m
Cdifference in height of buildings is 500
D $t = 0$ sec
A ball is thrown vertically upward with a velocity u from balloon descending with a constant velocity v. The ball will pass by the balloon after time
A $\frac{u + v}{2 g}$
B $\frac{2 u + v}{g}$
C $\frac{2 u + 2 v}{g}$
D $\frac{(u + v)}{g}$

AAKASH INSTITUTE-MOTION IN A PLANE-Assignement section -J (Aakash Challengers Questions)

Home
Ask Doubt
Profile

The correct Answer is:(i) x=ay22v0 (ii) atotal=av0atangential=v0a2y√v20+a2y2,anormal=av20√v20+a2y2

Topper's Solved these Questions

Similar Questions

Knowledge Check

A balloon starts rising from the ground with an acceleration of 1.25ms−2. After 8 seconds, a stone is released from the balloon. After releasing, the stone will:

A ball is thrown vertically upward with a velocity u from balloon descending with a constant velocity v. The ball will pass by the balloon after time

AAKASH INSTITUTE-MOTION IN A PLANE-Assignement section -J (Aakash Challengers Questions)

The correct Answer is:(i) $x = \frac{a y^{2}}{2 v_{0}}$ (ii) $a_{total} = a v_{0} a_{tangential} = \frac{v_{0} a^{2} y}{\sqrt{v_{0}^{2} + a^{2} y^{2}}}, a_{normal} = \frac{a v_{0}^{2}}{{\sqrt{v}}_{0}^{2} + a^{2} y^{2}}$

A balloon starts rising from the ground with an acceleration of $1.25 m s^{- 2}$ . After 8 seconds, a stone is released from the balloon. After releasing, the stone will: