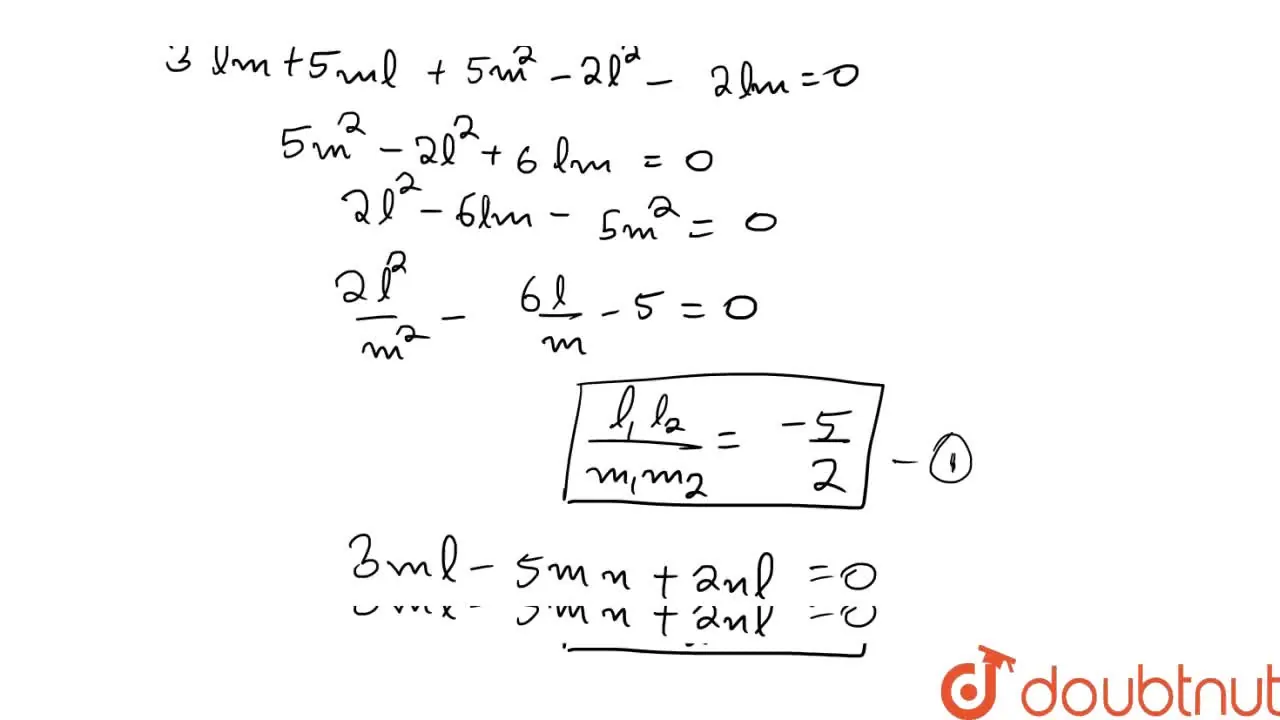
The direction cosines of two lines satisfying the conditions l + m +

The direction cosines of two lines satisfying the
conditions $l + m + n = 0 and 3 l m - 5 m n + 2 n l = 0$
where l, m, n are the direction cosines.
The value of $l m + m n + n l$ is
A0
B $- 1$
C $- \frac{1}{2}$
D1
l = m = n = 1 are the direction Cosines of
AX-axis
BY-axis
CZ-axis
Dno line
If l,m,n are direction cosines of a line , then vector $l i + m j + n k$ is a
Anull vector
Bunit vector
Cnon-unit vector
Dbound vector

The direction cosines of two lines satisfying the
conditions $l + m + n = 0 and 3 l m - 5 m n + 2 n l = 0$
where l, m, n are the direction cosines.
Angle between the lines is