Find the extrema points of $f (x) = 3 x^{4} - 4 x^{3} - 36 x^{2} + 28$

Video Solution

Text Solution

Verified by Experts

$f' (x) = 12 x^{3} - 12 x^{2} - 72 x$
$= 12 x (x + 2) (x - 3)$
Using first order derivative test ,
We determine the sign of $f' (x)$ using a number line .

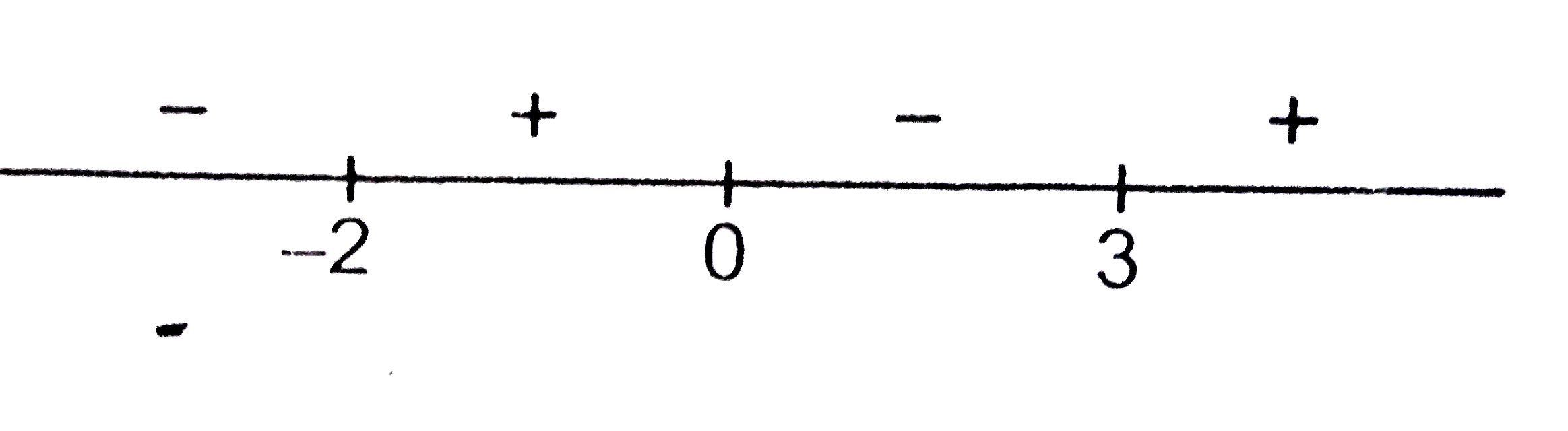
We observe that
$x = - 2, 3$ (Points of local minima )
x=0 (Points of local maxima )
Using seond order derivative test
$f' (x) = 12 x (x + 2) (x - 3)$
$f'' (x) = 36 x^{2} - 24 x - 72$
$= 12 (3 x^{2} - 2 x - 6)$
$f'' (0) < 0 \Rightarrow$ x=0 is a local maximum
$f'' (- 2) > 0 \Rightarrow$ x=-2 is a local minimum
$f'' (3) > 0 \Rightarrow$ x=3 is a local minimum

Updated on:21/07/2023

|

Class 12 MATHS APPLICATION OF DERIVATIVES

Topper's Solved these Questions

Similar Questions

Knowledge Check

If $f (x) = 3 x^{4} + 4 x^{3} - 12 x^{2} + 12$ , then f(x) is
Aincreasing in $(- \infty, 2) and$ in (0,1)
Bincreasing in (-2,0) and in $(1, \infty)$
Cdecreasing in $(- 2, 0) and$ in (0,1)
Ddecreasing in $(- \infty, - 2) and$ in (1, $\infty$ )

AAKASH INSTITUTE-APPLICATION OF DERIVATIVES-Assignment SECTION-J (Aakash Challengers Questions )

Home
Ask Doubt
Profile