Find the intervals in which f(x)=4x3−45x2+168x−16 is (i) Strictly increasing (ii) Strictly decreasing
f(x)=4x3−45x2+168x−16 ltbr? f'(x)=12x2−90x+168
=6(2x2−15x+28)
f'(x)=6(x−4)(2x−7)
(i) For strictly increasing
f'(x)>0
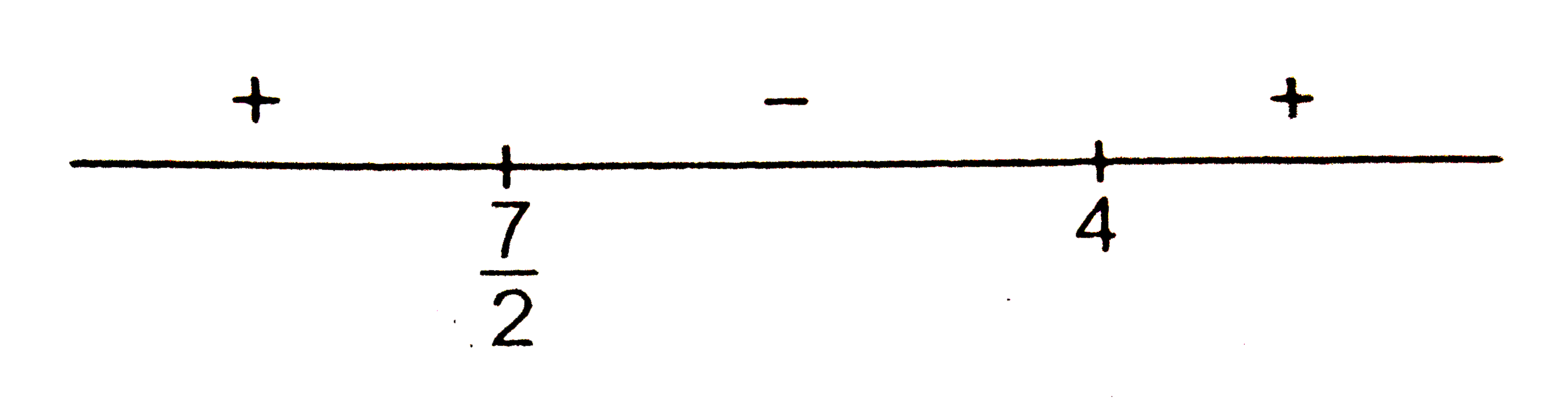
⇒(x−4)(2x−7)>0
⇒x∈(−∞,72)∪(4,∞)

(ii) For strictly decreasing
f'(x)<0
⇒(x−4)(2x−7)<0
⇒x∈(72,4)
Topper's Solved these Questions
- APPLICATION OF DERIVATIVESBOOK - AAKASH INSTITUTECHAPTER - APPLICATION OF DERIVATIVESEXERCISE - TRY YOURSELF
39 Videos
- APPLICATION OF DERIVATIVESBOOK - AAKASH INSTITUTECHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Assignment SECTION-A (Competition Level Questions)
50 Videos
- APPLICATION OF INTEGRALS BOOK - AAKASH INSTITUTECHAPTER - APPLICATION OF INTEGRALS EXERCISE - Assignment Section - I Aakash Challengers Questions
2 Videos
Similar Questions
Find the values of x for which f(x)=2x3−15x2−144x−7 is
(a) Strictly increasing (b) strictly decreasing
View SolutionFind the intervals in which the function f(x)=3x4−4x3−12x2+5 is (a) strictly increasing (b) strictly decreasing
View SolutionFind the intervals in which f(x)=32x4−4x3−45x2+51
View SolutionFind the intervals in which the function f(x)=32x4−4x−45x+51 is (a) strictly increasing. (b) strictly decreasing.
View SolutionFind the intervals in which the function f given by f(x)=x2−4x+6 is (a) strictly increasing (b) strictly decreasing
View SolutionFind the interval in which the function f given by f(x)=x2−2x+3 is
(a) Strictly increasing
(b) Strictly decreasing
View SolutionFind the intervals on which the function f(x)=6−9x−x2 is (a) strictly increasing (b) strictly decreasing
View SolutionFind the intervals in which the function f given by f(x)=2x2−3xis
(a) strictly increasing (b) strictly decreasing
View SolutionFind the intervals on which the function f(x)=10−6x−2x2 is (a) strictly increasing (b) strictly decreasing.
View SolutionFind the intervals in which the function f given by f(x)=2x3−3x2−36x+7is (a) strictly increasing (b) strictly decreasing
View Solution
