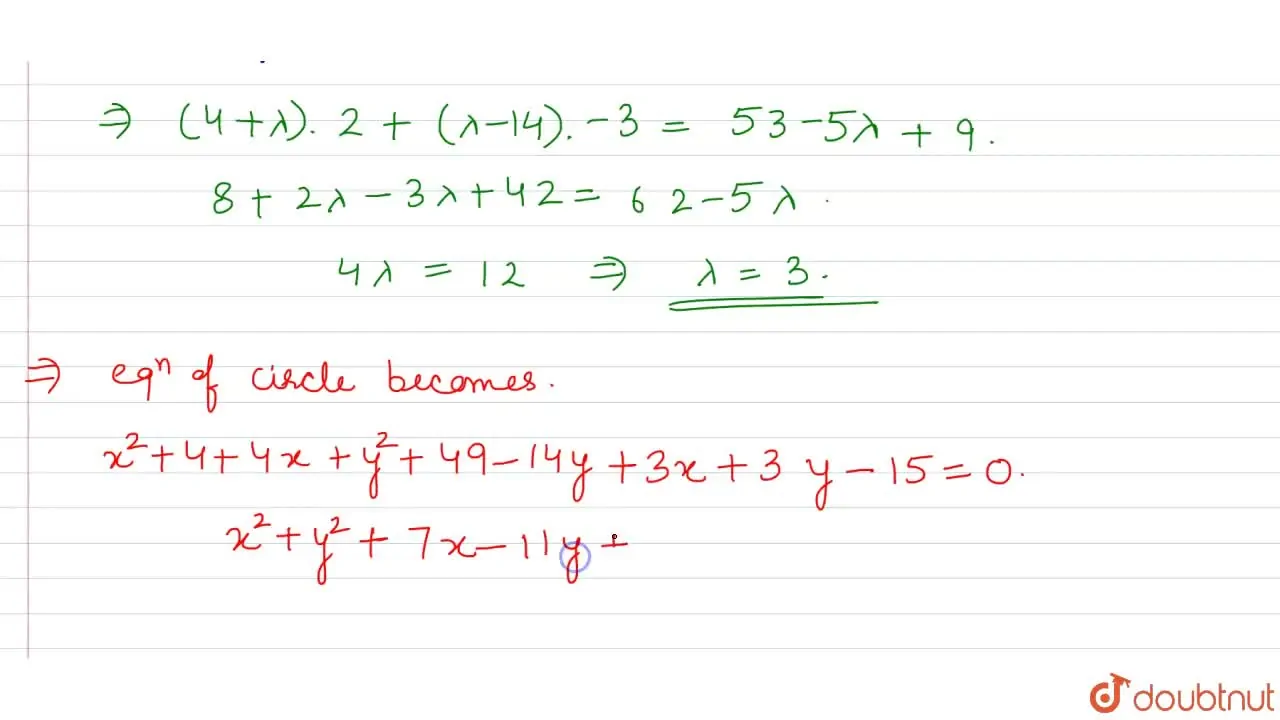The equation of a circle which touches the line x+y=5 at N(−2,7) and cuts the circle x2+y2+4x−6y+9=0 orthogonally, is
x2+y2+7x−12y+m38=0
x2+y2+7x+11y+38=0
x2+y2+7x−11y−38=0
x2+y2−7x−11y+39=0
The correct Answer is:A
Step by step video, text & image solution for The equation of a circle which touches the line x +y= 5 at N(-2,7) and cuts the circle x^2 + y^2 + 4x-6y + 9 = 0 orthogonally, is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - AAKASH INSTITUTECHAPTER - CONIC SECTIONSEXERCISE - SECTION-C ( Objective Type Questions ( More than one answer))1videos
CONIC SECTIONS
- BOOK - AAKASH INSTITUTECHAPTER - CONIC SECTIONSEXERCISE - SECTION-C45videos
CONIC SECTIONS
- BOOK - AAKASH INSTITUTECHAPTER - CONIC SECTIONSEXERCISE - Assignment (SECTION - A)55videos
CONIC SECTIONS
- BOOK - AAKASH INSTITUTECHAPTER - COMPLEX NUMBERS AND QUADRATIC EQUATIONSEXERCISE - section-J (Aakash Challengers Qestions)16videos
COMPLEX NUMBERS AND QUADRATIC EQUATIONS
- BOOK - AAKASH INSTITUTECHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - section - J6videos
CONTINUITY AND DIFFERENTIABILITY
Similar Questions
The equation of a circle which touches the line x+y=5 at N(−2,7) and cuts the circle x2+y2+4x−6y+9=0 orthogonally, is
View SolutionThe equation of a circle touches the line x+y=5 at N(−27) and cuts the circle x2+y2+4x−6y+9=0 orthogonally, is
View SolutionThe radius of the circle which touches the line x+y=0 at M (−1,1) and cuts the circle x2+y2+6x−4y+18=0 orthogonally, is
View SolutionA circle touching the line x+y−2=0 at (1,1) and cuts the circle x2+y2+4x+5y−6=0 at P and Q. Then
View SolutionThe equation of circle which pass through the points (2.0),(0.2)and orthogonal to the circle 2x2+2y2+5x−6y+4=0
View SolutionThe locus of the centre of a circle which touches the line x−2=0 and cuts orthogonally the circle x2+y2−20x+4=0 is
View SolutionThe equation of the circle which passes through the origin, center lies on the line x+y = 4 and cuts the circle x2+y2−4x+2y+4=0 orthogonally :
View SolutionIf centre of a circle lies on the line 2x−6y+9=0 and it cuts the circle x2+y2=2 orthogonally then the circle passes through two fixed points
View SolutionEquation of the circle which passes through origin and whose centre lies on the line x+y=4 and cuts the circle x2+y2−4x+2y+4=0 orthogonally is
View SolutionFind the equation of the circle , centred at (-1,4) , which touches the circle x2+y2−6x−2y+1=0 externally
View Solution