Prove that the points A(4,0),B(6,1),C(4,3) and D(3, 2) are concyclic.
Our strategy would be to show that D(3,2) lies on the circumcircle of △ABC.
Note that the points A(4, 0) and C(4, 3) have the same x-coordinates, viz x = 4.
Thus AC is parallel to y-axis.
∴ The perpendicular bisector of AC through M1(4,32) is perpendicular to y-axis.
hence y-coordinates S of triangle ABC is 32.
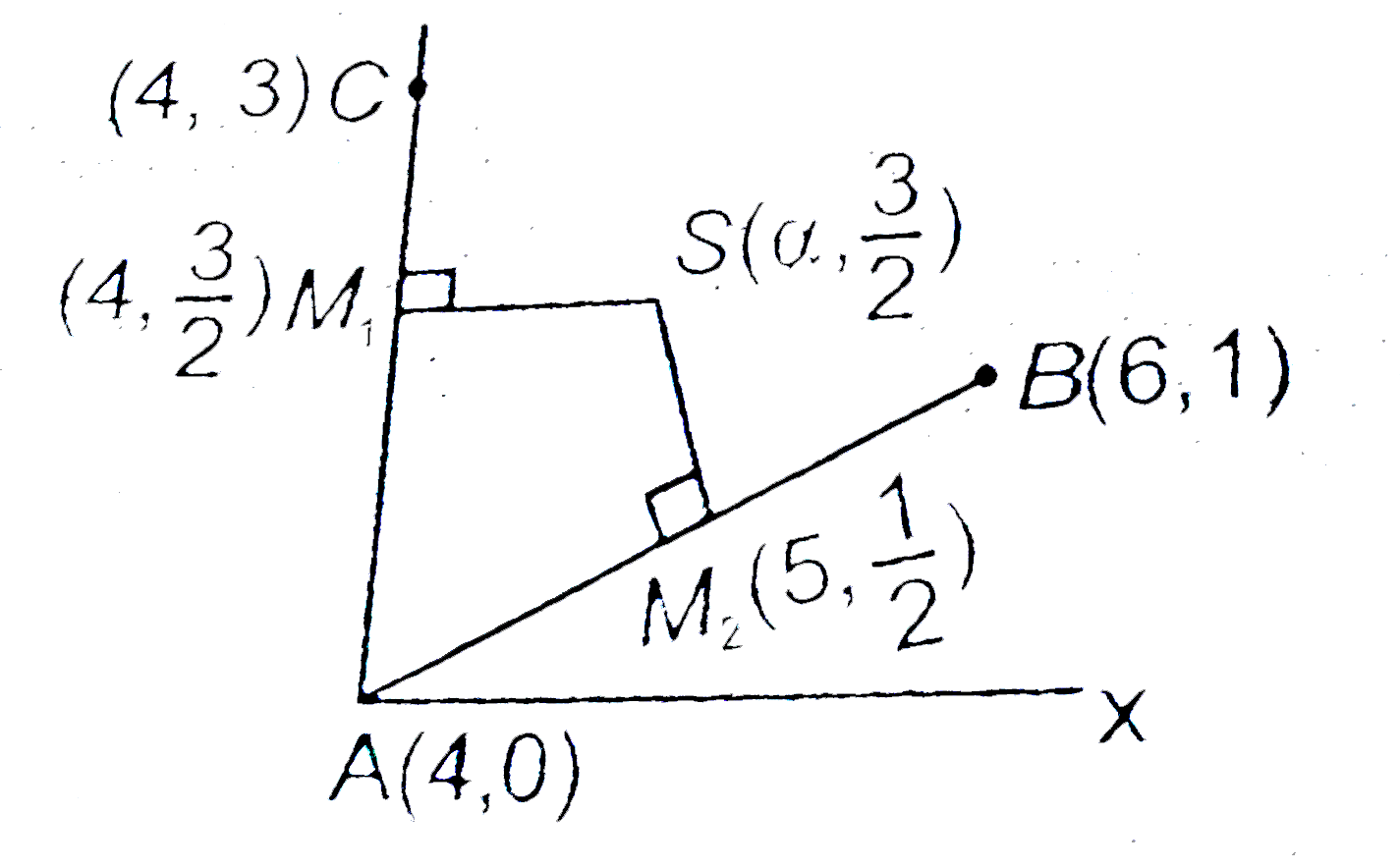
Let S be (α,32) now M2 is mid-point of AB and M2S perpendicular ot AB. (The centre of a circle is the point of intersection of two perpendicular bisectors of the non-parallel chords).
⇒32−12α−5⋅1−06−4=−1 ⇒1α−5⋅12=−1
⇒α−5=−12
⇒α=5−12 ∴α=92
Thus S is (92,32).
SA=√(α−4)2+(32)2=√(92−4)2+(32)2=√(12)2+(32)2
=√104=√52
SA=√52, therefore the circumcircle of △ABC has the centre (93,32) and radius √52. Its equation then is
(x−92)2+(y−32)2=52......(A)
The point D( 3, 2) lies on (A) - one can check it easily. Thus points A, B, C, D are concyclic
Topper's Solved these Questions
- CONIC SECTIONSBOOK - AAKASH INSTITUTECHAPTER - CONIC SECTIONSEXERCISE - Try ypurself
42 Videos
- CONIC SECTIONSBOOK - AAKASH INSTITUTECHAPTER - CONIC SECTIONSEXERCISE - Assignment (SECTION - A)
55 Videos
- COMPLEX NUMBERS AND QUADRATIC EQUATIONSBOOK - AAKASH INSTITUTECHAPTER - COMPLEX NUMBERS AND QUADRATIC EQUATIONSEXERCISE - section-J (Aakash Challengers Qestions)
16 Videos
- CONTINUITY AND DIFFERENTIABILITYBOOK - AAKASH INSTITUTECHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - section - J
6 Videos
Similar Questions
Prove that the points A(0, 4, 1), B(2, 3, -1), C(4, 5, 0) and D(2, 6, 2) are vertices of a square.
View SolutionProve that the points (1,-6), (5,2) , (7,0) and (-1,-4) are concyclic. Find the radius of the circle .
View SolutionUsing slopes, prove that the points A(−2,−1), B(1,0), C(4,3) and D(1,2) are the vertices of a parallelogram.
View SolutionShow that the points A(2, 1), B(5, 2), C(6, 4) and D(3, 3) are the angular points of a parallelogram. Is this figure a rectangle?
View SolutionThe distinct points A(0,0),B(0,1),C(1,0).andD(2a,3a) are concyclic than
View SolutionProve that the points A(3,−2,4),B(3,−2,4),B(1,1,1)andC(−1,4,−2) are collinear.
View SolutionIf the points (2,0),(0,1),(4,0) and (0,a) are concyclic then a=
View SolutionShow that the points A(3, 1), B(0, -2), C(1, 1) and D(4, 4) are the vertices of a parallelogram ABCD.
View SolutionProve that the points A (1,2,3) , B (4,0,4), C (-2,4,2) and (7,-2,5) and collinear.
View SolutionShow that the point A(4,−1,2),B(−3,5,1),C(2,3,4) and D(1,6,6) are coplanar. Also find the equation of the plane passing through these points.
View Solution
