If the angle between two lines is and the slope of one line is 2, then the product of possible slopes of other line is
1
2
6
The correct Answer is:A
To find the product of the possible slopes of the other line when the angle between two lines is 45∘ and the slope of one line is m1=2, we can use the formula for the tangent of the angle between two lines.
1. Understand the Given Information:
- The angle between the two lines, θ=45∘.
- The slope of one line, m1=2.
2. Use the Tangent Formula:
The formula for the tangent of the angle between two lines is given by:
tan(θ)=m1−m21+m1m2
For θ=45∘, we have:
tan(45∘)=1
Thus, we can set up the equation:
1=2−m21+2m2
3. Cross-Multiply to Solve for m2:
Cross-multiplying gives:
1+2m2=2−m2
4. Rearrange the Equation:
Rearranging the equation:
2m2+m2=2−1
This simplifies to:
3m2=1
Therefore:
m2=13
5. Consider the Negative Case:
The angle can also be negative, so we consider:
−1=2−m21+2m2
Cross-multiplying gives:
−1−2m2=2−m2
6. Rearrange the Equation:
Rearranging this equation:
−2m2+m2=2+1
This simplifies to:
−m2=3
Therefore:
m2=−3
7. Find the Product of the Slopes:
Now we have two possible values for m2:
m2=13andm2=−3
The product of the possible slopes is:
Product=(13)×(−3)=−1
Final Answer:
The product of the possible slopes of the other line is −1.
---
- The angle between the two lines, θ=45∘.
- The slope of one line, m1=2.
The formula for the tangent of the angle between two lines is given by:
tan(θ)=m1−m21+m1m2
For θ=45∘, we have:
tan(45∘)=1
Thus, we can set up the equation:
1=2−m21+2m2
Cross-multiplying gives:
1+2m2=2−m2
Rearranging the equation:
2m2+m2=2−1
This simplifies to:
3m2=1
Therefore:
m2=13
The angle can also be negative, so we consider:
−1=2−m21+2m2
Cross-multiplying gives:
−1−2m2=2−m2
Rearranging this equation:
−2m2+m2=2+1
This simplifies to:
−m2=3
Therefore:
m2=−3
Now we have two possible values for m2:
m2=13andm2=−3
The product of the possible slopes is:
Product=(13)×(−3)=−1
The product of the possible slopes of the other line is −1.
Topper's Solved these Questions
- STRAIGHT LINESBook:AAKASH INSTITUTEChapter:STRAIGHT LINESExercise:ASSIGNMENT (SECTION C) (OBJECTIVE TYPE QUESTIONS) (MORE THAN ONE ANSWER)
Explore 14 Videos
- STRAIGHT LINESBook:AAKASH INSTITUTEChapter:STRAIGHT LINESExercise:ASSIGNMENT (SECTION D) (LINKED COMPREHENSION TYPE QUESTIONS)
Explore 12 Videos
- STRAIGHT LINESBook:AAKASH INSTITUTEChapter:STRAIGHT LINESExercise:ASSIGNMENT (SECTION A) (OBJECTIVE TYPE QUESTIONS) (ONLY ONE ANSWER)
Explore 50 Videos
- STATISTICSBook:AAKASH INSTITUTEChapter:STATISTICSExercise:Section-C Assertion-Reason
Explore 15 Videos
- THREE DIMENSIONAL GEOMETRY Book:AAKASH INSTITUTEChapter:THREE DIMENSIONAL GEOMETRY Exercise:ASSIGNMENT SECTION - J
Explore 10 Videos
Similar Questions
Knowledge Check
Slope of budget line is
Amarginal opportunity costBmarginal rate of substitutionCmarket rate of exchangeDall of theseThe slope of the given line is ,
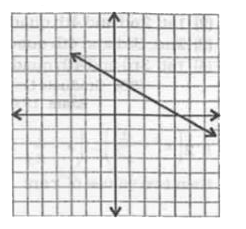 APositiveBNegativeCUndefinedDZero
APositiveBNegativeCUndefinedDZeroCalculate slope of shown line
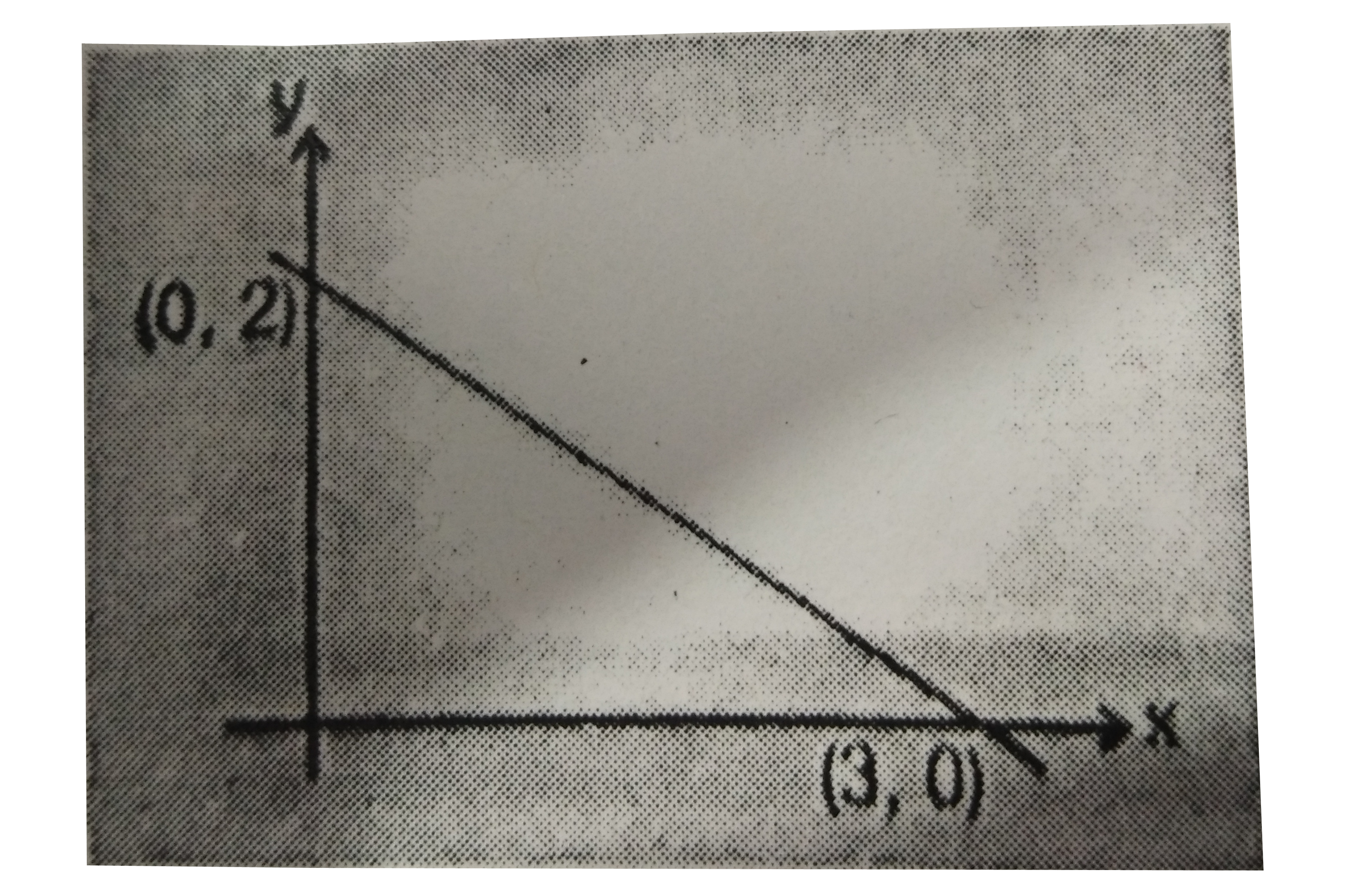 A1B-2C0D-2/3
A1B-2C0D-2/3