If n things are arranged in a row, the number of way in which they can be arranged so that non occupies its original position is n!(1−11!+12!−13!+⋅⋅⋅+(−1)n1n!)
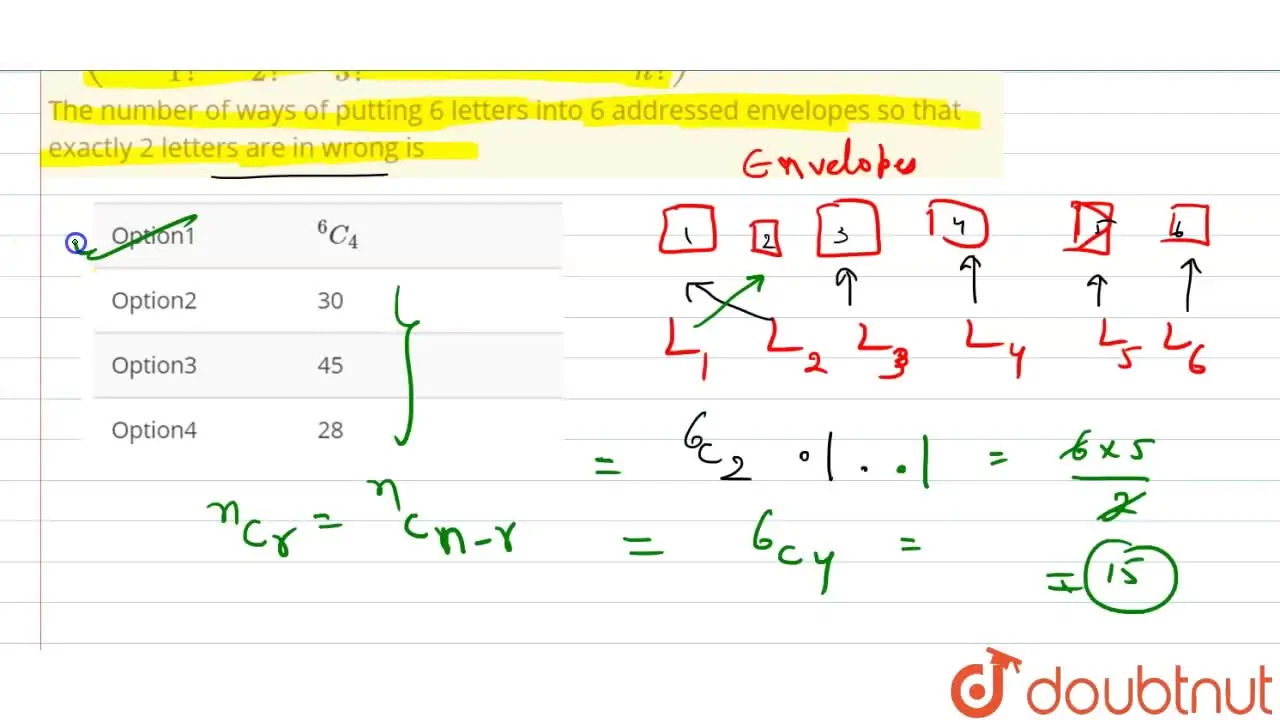
The number of ways of putting 6 letters into 6 addressed envelopes so that exactly 2 letters are in wrong is
6C4
30
45
28
The correct Answer is:A
Step by step video, text & image solution for If n things are arranged in a row, the number of way in which they can be arranged so that non occupies its original position is n!(1-(1)/(1!)+(1)/(2!)-(1)/(3!)+* * * + (-1)^(n)(1)/(n!)) The number of ways of putting 6 letters into 6 addressed envelopes so that exactly 2 letters are in wrong is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - AAKASH INSTITUTECHAPTER - PERMUTATIONS AND COMBINATIONS EXERCISE - Assignment Section E (Assertion-Reason Type Questions)5videos
PERMUTATIONS AND COMBINATIONS
- BOOK - AAKASH INSTITUTECHAPTER - PERMUTATIONS AND COMBINATIONS EXERCISE - Assignment Section F (Matrix-Match Type Questions)6videos
PERMUTATIONS AND COMBINATIONS
- BOOK - AAKASH INSTITUTECHAPTER - PERMUTATIONS AND COMBINATIONS EXERCISE - Assignment Section C Objective type questions (One option is correct )17videos
PERMUTATIONS AND COMBINATIONS
- BOOK - AAKASH INSTITUTECHAPTER - MATRICESEXERCISE - Assignment (Section - J) Aakash Challengers Questions3videos
MATRICES
- BOOK - AAKASH INSTITUTECHAPTER - PRINCIPLE OF MATHEMATICAL EXERCISE - Section-D:(Assertion-Reason Type Questions)12videos
PRINCIPLE OF MATHEMATICAL
Similar Questions
If n things are arranged in a row, the number of way in which they can be arranged so that non occupies its original position is n!(1−11!+12!−13!+⋅⋅⋅+(−1)n1n!)
The number of ways of putting at least 3 out of 6 letters in wrong envelops isView SolutionIf n things are arranged in a row, the number of way in which they can be arranged so that non occupies its original position is n!(1−11!+12!−13!+⋅⋅⋅+(−1)n1n!)
Five boys are to be seated in a row. The number of ways in which 3 boys are not seated in the place specfied to them isView SolutionIf the number of ways in which 7 letters can be put in 7 envelopes such that exactly four letters are in wrong envelopes is N, then
View SolutionBy selling an object for Rs 900, a man earns a profit that is double the loss incurred when the object is sold for Rs 450. If he sells it at a profit of 20%, find the selling price of this object.
View SolutionThe number of ways that all the letters of the word SWORD can be arranged such that no letter is in its original position is
View SolutionFind the number of ways in which the letters of word KUTKUT Can be arranged So that no two alike letters are together.
View SolutionThe number of ways in which the letters of the word 'ARRANGE' can be arranged so that two A's are together is
View SolutionThe number of ways in which four different letters can be put in their four addressed envelopes such that atleast two of them are in the wrong envelopes are
View SolutionIf n,(n>5) things are arranged in a circular order, the number of ways of selecting three of the things so that no two of which are next to each other is
View SolutionA person buys a radio for 1030 and he spent 50 on its repairs. If he sold it for 1200, find the profit percent
View Solution