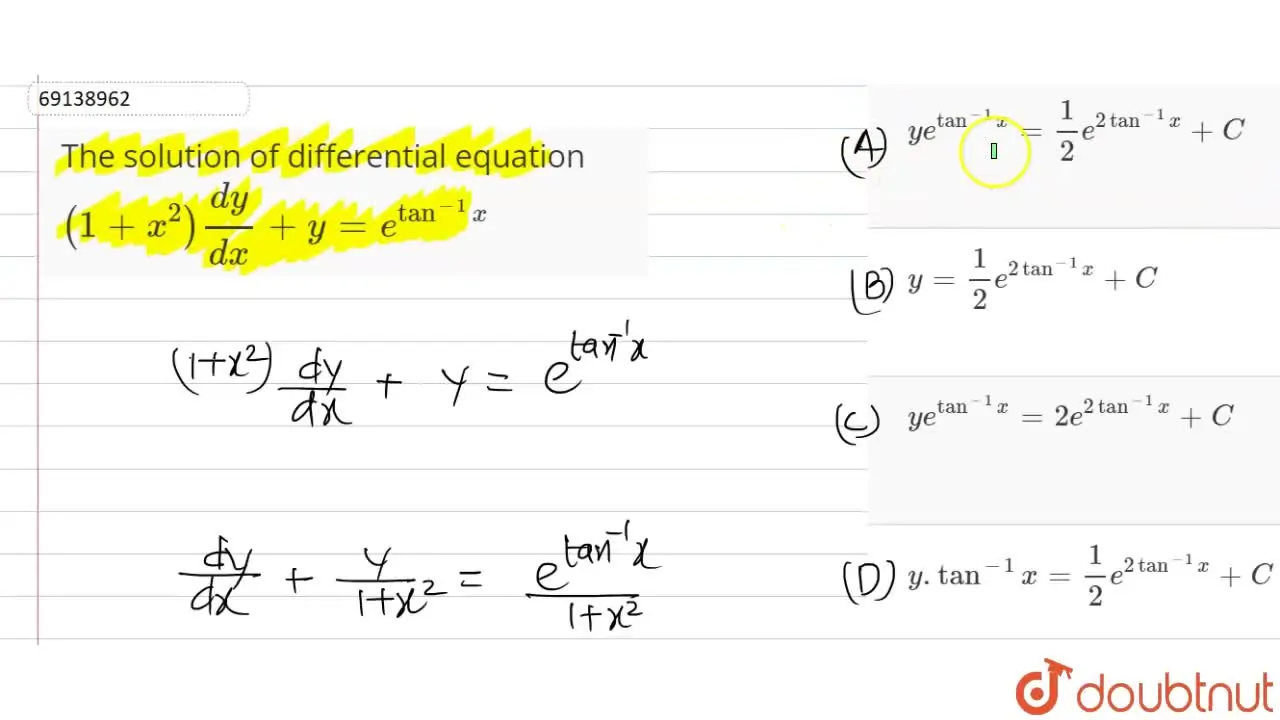
The solution of differential equation
$(1 + x^{2}) \frac{d y}{d x} + y = e^{{tan}^{- 1} x}$

A

$y e^{{tan}^{- 1} x} = \frac{1}{2} e^{2 {tan}^{- 1} x} + C$

B

$y = \frac{1}{2} e^{2 {tan}^{- 1} x} + C$

C

$y e^{{tan}^{- 1} x} = 2 e^{2 {tan}^{- 1} x} + C$

D

$y . {tan}^{- 1} x = \frac{1}{2} e^{2 {tan}^{- 1} x} + C$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

|

Answer

Step by step video, text & image solution for The solution of differential equation (1+x^(2)) (dy)/(dx) + y = e^(tan^(-1)x) by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 MATHS DIFFERENTIAL EQUATIONS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One or More
Solution of the differential equation $(1 + x^{2}) \frac{d y}{d x} + y = {tan}^{- 1} x$ is
A $y e^{{tan}^{- 1} x} = (1 - {tan}^{- 1} x) e^{{tan}^{- 1} y} + c$
B $y e^{{tan}^{- 1} x} = ({tan}^{- 1} x - 1) e^{{tan}^{- 1} x} + c$
C $y e^{{tan}^{- 1} x} = ({tan}^{- 1} x - 1) e^{{tan}^{- 1} x} + c$
DNone of these
Question 2 - Select One
The solution of the differential equation $(1 + y^{2}) d x + (x - e^{- {tan}^{- 1} y}) d y = 0$ is
A $y e^{{tan}^{- 1} x} = {tan}^{- 1} x + C$
B $x e^{{tan}^{- 1} y} = {tan}^{- 1} y + C$
C $y = {tan}^{- 1} x e^{{tan}^{- 1} x} + C$
D $y = x e^{{tan}^{- 1} x} + C$
Question 3 - Select One
The integrating factor of the differential equation $(1 + x^{2}) \frac{d y}{d x} + y = e^{{tan}^{- 1} x}$ is
A ${tan}^{- 1} x$
B $1 + x^{2}$
C $e^{{tan}^{- 1} x}$
D ${log}_{e} (1 + x^{2})$

AAKASH INSTITUTE-DIFFERENTIAL EQUATIONS-Assignment Section - B (Objective Type Questions (One option is correct))

Home
Ask Doubt
Profile