Prove that:
Video Solution
Text Solution
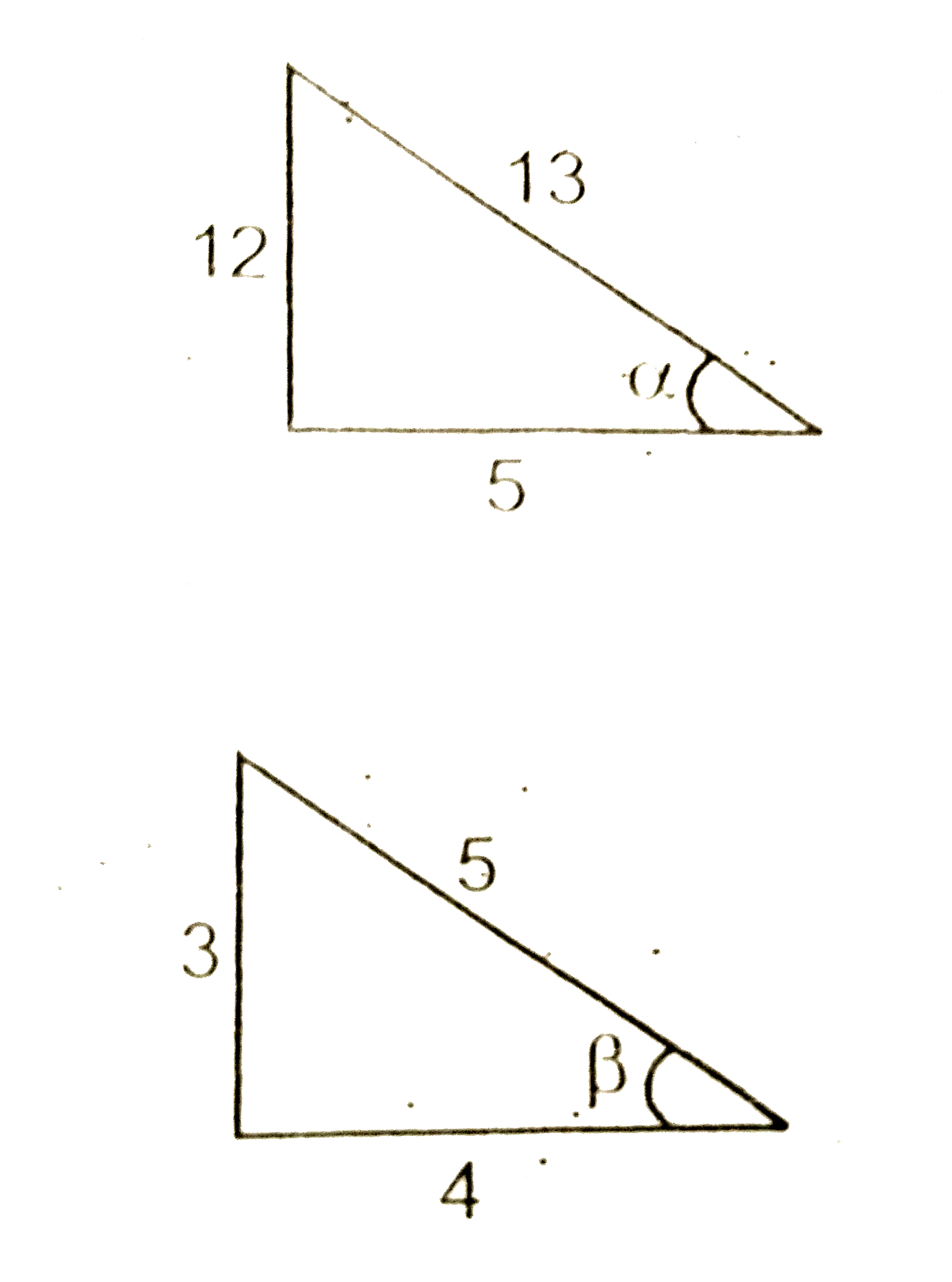
Let
Now,
Here,
We cannot use
Consider,
|
Topper's Solved these Questions
- INVERSE TRIGONOMETRIC FUNCTIONSBook:AAKASH INSTITUTEChapter:INVERSE TRIGONOMETRIC FUNCTIONSExercise:TRY YOURSELF
Explore 21 Videos
- INVERSE TRIGONOMETRIC FUNCTIONSBook:AAKASH INSTITUTEChapter:INVERSE TRIGONOMETRIC FUNCTIONSExercise:ASSIGNMENT (SECTION - A)(OBJECTIVE TYPE QUESTIONS (ONE OPTION IS CORRECT))
Explore 45 Videos
- INTEGRALSBook:AAKASH INSTITUTEChapter:INTEGRALSExercise:Try yourself
Explore 50 Videos
- LIMITS AND DERIVATIVES Book:AAKASH INSTITUTEChapter:LIMITS AND DERIVATIVES Exercise:Section - j
Explore 3 Videos
Similar Questions
Prove that: sin−1(12)13+cos−145+tan−1(63)16=π
View SolutionShow that sin−1'513+cos−1'35=tan−1'6316.
View SolutionProve that: sin−1(513)+cos−1(45)=12sin−1(36964225)
View SolutionProve that sin−1(513)+sin−1(1665)=cos−1(45)
View SolutionProve that
cos−1(513)+cos−1(−725)+sin−1(36)325=π
View SolutionProve that:cos−1(1213)+sin−1(35)=sin−1(5665)
View SolutionLet α=2tan−1(12)+sin−135andβ=sin−1(1213)+cos−1(45)+cos−1(1663) be such that2sinαandcosβ are roots of the equation x2−px+q=0, then (p−q) is
View SolutionProve that: sin−1(35)+cos−1(1213)=sin−1(5665)
View Solutionsin−1(513)+tan−1(125)=
View SolutionProve that:tan−1(6316)=sin−1(513)+cos−1(35)
View Solution