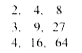Determine the maximum that hydrogen in its ground state can absorb. What would be the next smaller wavelength that would work ?

Video Solution

Text Solution

Verified by Experts

Strategy : Maximum wavelength corresponds to minimum energy, and this would be the jump from the ground state up to the first excited state. The next smaller wavelength occurs for the jump from the ground state to the second excited state. In each case, the energy difference can be used to find the wavelength .
The energy needed to jump from the ground state to the first excited state is 13.6 eV-3.4 eV=10.2 eV , the required wavelength, as we saw in one of the previous example, is 122 nm. The energy to jump from the ground state to the second excited state is 13.6 eV-1.5 eV=12.1 eV, which corresponds to a wavelength
$λ = \frac{c}{f} = \frac{hc}{hf} = \frac{hc}{E_{3} - E_{1}}$
$= \frac{(6.63 \times 10^{- 34} J . s) (3.00 \times 10^{8} m / s)}{(12.1 e V) (1.60 \times 10^{- 19} J / e V)}$
=103 nm

Updated on:21/07/2023

Determine the minimum wavelength that hydrogen in its ground state can obsorb. What would be the next smaller wavelength that would work?
A $133 n m$
B $13.3 n m$
C $10.3 n m$
D $103 n m$
The radius of hydrogen atom, in its ground state, is of the order of
A $10^{- 8}$ cm
B $10^{- 6}$ cm
C $10^{- 5}$ cm
D $10^{- 4}$ cm
The maximum wavelength that a sample of hydrogen atoms can absorb is
A912 A
B1216 A
C1028 A
DInfinite