A straight solenoid of length 50 cm has 1000 turns per metre and mean cross-sectional area of 2×10−4m2 . It is placed with its axis of 30∘ with uniform magnetic field of 0.32 T . Find the torque acting on the solenoid when a current of 2 ampere is passed through it .
To find the torque acting on a solenoid placed in a magnetic field, we can use the formula for torque (τ) on a current-carrying loop in a magnetic field:
τ=n⋅I⋅A⋅B⋅sin(θ)
Where:
- n = number of turns in the solenoid
- I = current through the solenoid
- A = cross-sectional area of the solenoid
- B = magnetic field strength
- θ = angle between the magnetic field and the axis of the solenoid
Step 1: Calculate the total number of turns in the solenoid
Given:
- Length of the solenoid (L) = 50 cm = 0.5 m
- Number of turns per meter = 1000 turns/m
Total number of turns (N) can be calculated as:
N=n⋅L=1000turns/m⋅0.5m=500turns
Step 2: Identify the values for current, area, magnetic field, and angle
Given:
- Current (I) = 2 A
- Cross-sectional area (A) = 2×10−4m2
- Magnetic field (B) = 0.32 T
- Angle (θ) = 30∘
Step 3: Calculate sin(θ)
We know:
sin(30∘)=12
Step 4: Substitute all values into the torque formula
Now substitute N, I, A, B, and sin(θ) into the torque formula:
τ=N⋅I⋅A⋅B⋅sin(θ)
Substituting the values:
τ=500⋅2⋅(2×10−4)⋅0.32⋅12
Step 5: Simplify the expression
Calculating step by step:
τ=500⋅2=1000
τ=1000⋅(2×10−4)=0.2
τ=0.2⋅0.32=0.064
τ=0.064⋅12=0.032N m
Final Answer
The torque acting on the solenoid is:
τ=0.032N m
---
- n = number of turns in the solenoid
- I = current through the solenoid
- A = cross-sectional area of the solenoid
- B = magnetic field strength
- θ = angle between the magnetic field and the axis of the solenoid
Given:
- Length of the solenoid (L) = 50 cm = 0.5 m
- Number of turns per meter = 1000 turns/m
N=n⋅L=1000turns/m⋅0.5m=500turns
Given:
- Current (I) = 2 A
- Cross-sectional area (A) = 2×10−4m2
- Magnetic field (B) = 0.32 T
- Angle (θ) = 30∘
We know:
sin(30∘)=12
Now substitute N, I, A, B, and sin(θ) into the torque formula:
τ=N⋅I⋅A⋅B⋅sin(θ)
τ=500⋅2⋅(2×10−4)⋅0.32⋅12
Calculating step by step:
τ=500⋅2=1000
τ=1000⋅(2×10−4)=0.2
τ=0.2⋅0.32=0.064
τ=0.064⋅12=0.032N m
The torque acting on the solenoid is:
τ=0.032N m
Topper's Solved these Questions
- MAGNETISM AND MATTER BOOK - AAKASH INSTITUTECHAPTER - MAGNETISM AND MATTER EXERCISE - Try Yourself
53 Videos
- MAGNETISM AND MATTER BOOK - AAKASH INSTITUTECHAPTER - MAGNETISM AND MATTER EXERCISE - Assignment Section - A Objective Type Questions (One option is correct)
58 Videos
- LAWS OF MOTIONBOOK - AAKASH INSTITUTECHAPTER - LAWS OF MOTIONEXERCISE - Assignment (SECTION-D) (Assertion-Reason Type Questions)
15 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - AAKASH INSTITUTECHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - SECTION - J
9 Videos
Similar Questions
A straight solenoid of length 50 cm has 1000 turns per metre and mean cross-sectional area of 2×10−4m2 . It is placed with its axis of 30∘ with uniform magnetic field of 0.32 T . Find the torque acting on the solenoid when a current of 2 ampere is passed through it .
View SolutionA straight solenoid of length 50cm has 1000 turns and a mean cross sectional area of 2×10−4m2. It is placed with its axis at 30∘ with a uniform magnetic field of 0⋅31T. Find the torque acting on the solenoid when a current of 2A is passed through it.
View Solution
Knowledge Check
An air core solenoid has 1000turns and is one metre long. Its cross-sectional area is 10cm2. Its self-inductance is
A0.1256mHB12.56mHC1.256mHD125.6mHA closely wound solenoid of 1000 turns and area of cross-section 2×10−4m2 carries a current of 1A.It is placed in horizontal axis at30∘ with the diretion of uniform magnetic field of 0.16 T.Magnetic moment of solenoid and torque experienced by the solenoid due to the field are
A0.1Am2,0.016 NmB0.2Am2,0.026 NmC0.2Am2,0.016NmD0.4Am2,0.116NmA closely wound solenoid of 100 turns and area of cross section 5×10−4m2 carries current of 2A it is placed in such a way that its horizontal axis is at 30∘ with the direction of a uniform magnetic field of intensity 0.2 T what is the torque experienced by the solenoid in the magnetic field ?
A0.1 NmB0.2 NmC0.01 NmD0.02 Nm
A closely wound solenoid of 1000 turns and area of cross-section 2×10−4m2 carries a current of 1A. It is placed in a horizontal plane with its axis making an angle of 30∘ with the direction of uniform magnetic field of 0.16T. Calculate the torque acting on the solenoid.
View SolutionA straight solenoid of length 1m has 5000 turns in the primary and 200 turns in the secondary.If the area of cross-section is 4cm^(2) ,the mutual inductance will be
View SolutionA closely wound solenoid of 1000 turns and area of cross-section 2.0×10−4m2, carrying a current of 2.0 A. It is placed with its horizontal axis at 30∘ with the direction of a uniform horizontal magenitic field of 0.16 T, as shown in Fig. 11.3.
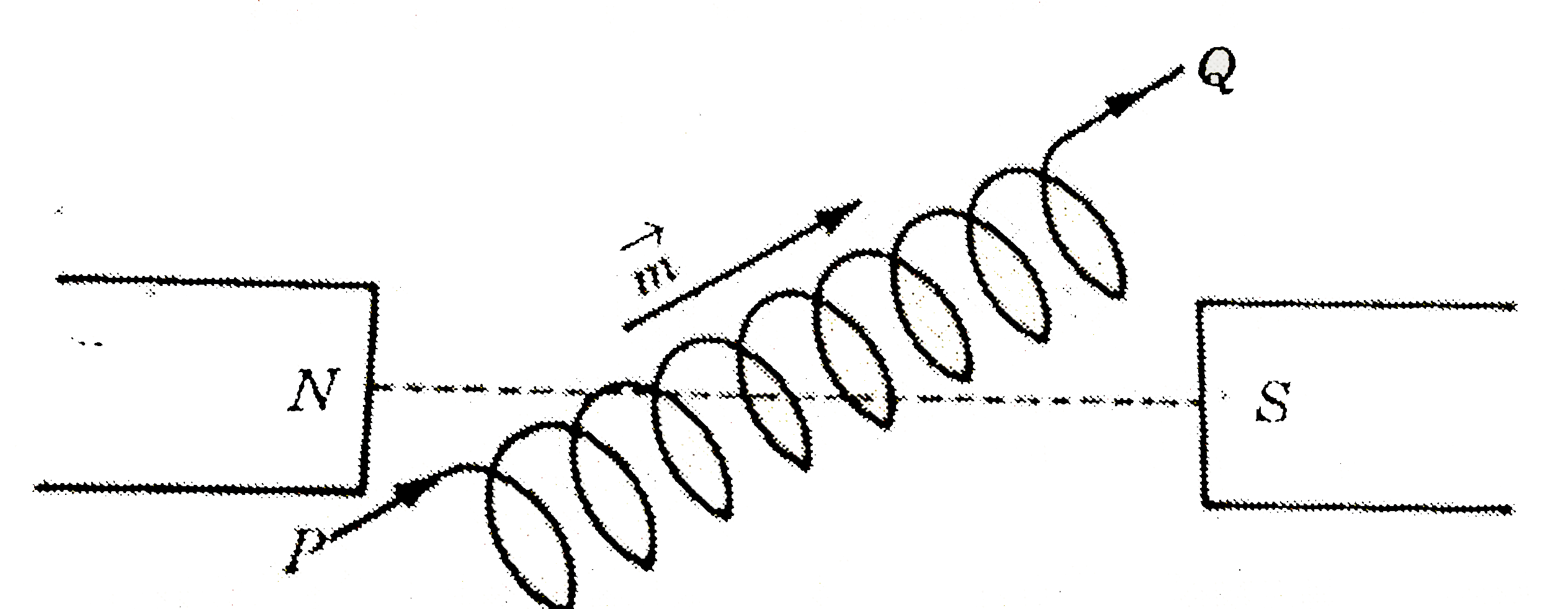
(a) What is the torque experienced by the solenoid due to the field ?
(b) If the solenoid is free to turn about the verticle direction, specify its orientations of stabvle and unstable equilibriums. What is the amount of work needed to displace the solenoid from its stable orientation to its unstable orientations ?
View SolutionA closely wound solenoid of 1000 tuins and area of Cross-section 2.0×10−4 in- carries a current of 2.0 A. It is placed with its horizontal axis al 30∘ with the direction of a uniform horizontal magnetie field of 0.16 T as shown in the figure. What is the magnitude of the restoring torque produced by the lield on the solenoid!

View SolutionA straight solenoid of length 1m has 5000 turns in the primary and 200 turns in the secondary. If the area of cross section is 4cm2, the mutual inductance will be
View Solution