$x (x, t) = \frac{0.8}{[{(4 x + 5 t)}^{2} + 5]}$ , represents a moving pulse where x and y are in metre and t is in second. Find
(i) The direction of wave propagation.
(ii) The wave speed.
(iii) The maximum displacement from the mean position (i.e., the aplitude of the wave).
(iv). Whether the wave pulse is symmetric or not.

Video Solution

Text Solution

Verified by Experts

(i) The equation represents a travelling wave because the argument of this function is 4x+5t, which is of the form $a x + b t$ . Because there is a positive sign between a and b, the wave is moving in the negative a axis direction.
(ii) The wave speed equals $\frac{b}{a}$ and here $a = 4 and b = 5$ . Hence the wave speed v is given by $v = \frac{5}{4} = 1.25$ unit.
(iii) Clearly y is maximum when (4x+50) is zero. hence the amplitude A is given by
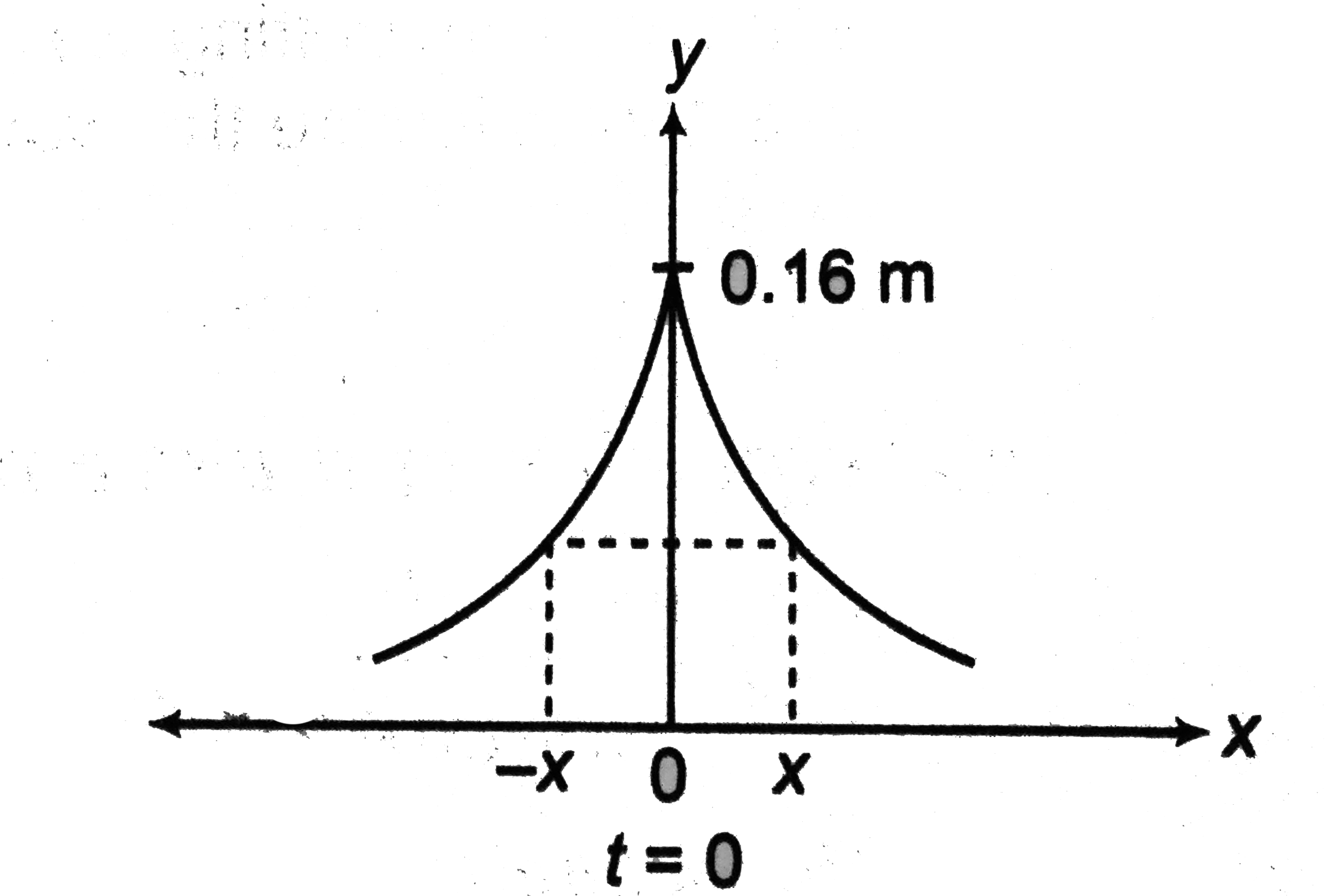
$A = \frac{0.8}{[{(0)}^{2} + 5]} = 0.16$ unit
(iv) At t=0, the wave pulse is represented by $y = \frac{0.8}{[16 x^{2} + 5]}$
As changing x to -x does not affect the value of y, wave pulse is symmeric.
the shape of this pulse is shown in the figure.
.

Updated on:21/07/2023

$x (x, t) = \frac{0.8}{[{(4 x + 5 t)}^{2} + 5]}$ , represents a moving pulse where x and y are in metre and t is in second. Find
(i) The direction of wave propagation.
(ii) The wave speed.
(iii) The maximum displacement from the mean position (i.e., the aplitude of the wave).
(iv). Whether the wave pulse is symmetric or not.
APulse is moving in +x direction.
BIn two seconds it will travel a distance of 2.5 m
CIt's maximum displacement is 0.16 m
DIt's a symmetric pulse.
$y (x, t) = 0.8 / {[4 x + 5 t)}^{2} + 5]$ represents a moving pulse, where $x$ and $y$ are in meter and $t$ in second. Then
A(a) pulse is moving in + x direction
B(b) in $2 s$ it will travel a distance of $2.5 m$
C( c ) its maximum displacement is $0.16 m$
D(d) it is a sysmmetric pulse
$y (x, t) = \frac{1.0}{[{(a x + b t)}^{2} + {(c x + d t)}^{2} + 5]}$ represents a moving pulse where x and y are in metres and t in seconds. It has speed of pulse $b / a$ . Find the correct option.
A $b c = a d$
B $b d = a c$
C $a b = c d$
Dnone of these