The angle which the free surface of a liquid filled in a container will make with horizontal if the container is accelerated horizontally with acceleration g√3 is
30∘
45∘
60∘
15∘
The correct Answer is:A
To solve the problem of finding the angle that the free surface of a liquid makes with the horizontal when a container is accelerated horizontally with an acceleration of g√3, we can follow these steps:
1. Understand the Situation:
- We have a container filled with liquid, which is being accelerated horizontally with an acceleration a=g√3.
- We need to determine the angle θ that the free surface of the liquid makes with the horizontal.
2. Identify Forces Acting on the Liquid:
- The liquid experiences two accelerations:
- The gravitational acceleration g acting downwards.
- The horizontal acceleration a=g√3.
3. Use Pressure Difference:
- Consider two points in the liquid: point B (at height h) and point C (at height 0).
- The pressure difference between these two points can be expressed as:
PB−PA=ρ⋅a⋅x
where x is the horizontal distance between points A and B.
4. Vertical Pressure Difference:
- The vertical pressure difference can be expressed as:
PB−PC=ρ⋅g⋅h
5. Set Up the Equations:
- From the first equation:
PB−PA=ρ⋅g√3⋅x
- From the second equation:
PB−PC=ρ⋅g⋅h
6. Relate the Pressures:
- Since PA and PC are both atmospheric pressure, we can set the two expressions for PB equal to each other:
PA+ρ⋅g√3⋅x=PA+ρ⋅g⋅h
- Cancel PA from both sides:
ρ⋅g√3⋅x=ρ⋅g⋅h
7. Simplify:
- Cancel ρ and g from both sides:
x√3=h
- Rearranging gives:
hx=1√3
8. Use Trigonometry:
- From the triangle formed by points A, B, and C, we have:
tan(θ)=hx
- Substitute the value we found:
tan(θ)=1√3
9. Find the Angle:
- The angle θ whose tangent is 1√3 is:
θ=30∘
Final Answer:
The angle which the free surface of the liquid makes with the horizontal is 30∘.
- We have a container filled with liquid, which is being accelerated horizontally with an acceleration a=g√3.
- We need to determine the angle θ that the free surface of the liquid makes with the horizontal.
- The liquid experiences two accelerations:
- The gravitational acceleration g acting downwards.
- The horizontal acceleration a=g√3.
- Consider two points in the liquid: point B (at height h) and point C (at height 0).
- The pressure difference between these two points can be expressed as:
PB−PA=ρ⋅a⋅x
where x is the horizontal distance between points A and B.
- The vertical pressure difference can be expressed as:
PB−PC=ρ⋅g⋅h
- From the first equation:
PB−PA=ρ⋅g√3⋅x
- From the second equation:
PB−PC=ρ⋅g⋅h
- Since PA and PC are both atmospheric pressure, we can set the two expressions for PB equal to each other:
PA+ρ⋅g√3⋅x=PA+ρ⋅g⋅h
- Cancel PA from both sides:
ρ⋅g√3⋅x=ρ⋅g⋅h
- Cancel ρ and g from both sides:
x√3=h
- Rearranging gives:
hx=1√3
- From the triangle formed by points A, B, and C, we have:
tan(θ)=hx
- Substitute the value we found:
tan(θ)=1√3
- The angle θ whose tangent is 1√3 is:
θ=30∘
The angle which the free surface of the liquid makes with the horizontal is 30∘.
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF FLUIDSBOOK - AAKASH INSTITUTECHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - SECTION - C
15 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - AAKASH INSTITUTECHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - SECTION - D
2 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - AAKASH INSTITUTECHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - SECTION - A
50 Videos
- MAGNETISM AND MATTER BOOK - AAKASH INSTITUTECHAPTER - MAGNETISM AND MATTER EXERCISE - ASSIGNMENT (SECTION D)
26 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - AAKASH INSTITUTECHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Assignment(Section-D)
12 Videos
Similar Questions
The angle which the free surface of a liquid filled in a container will make with horizontal if the container is accelerated horizontally with acceleration g√3 is
View SolutionWhat angle will the free surface of a liquid subtend with the horizontal when the liquid in a container is moving horizontally with constat ac celeration a as shown in fig.
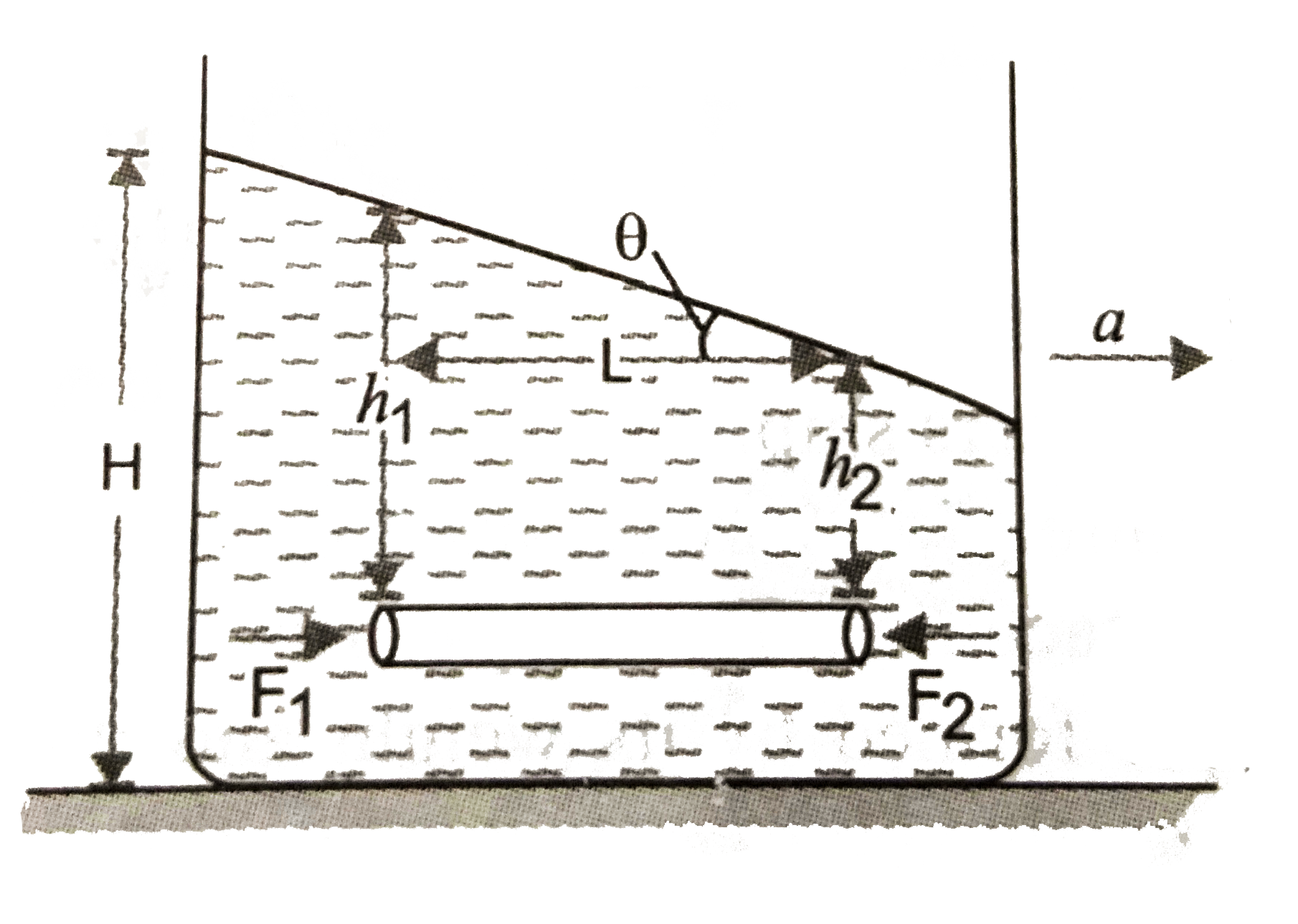
View Solution
Knowledge Check
If the surface of a liquid is plane, then the angle of contact of the liquid with the walls of container is
Aacute angleBobtuse angleC90∘D0∘If the surface of a liquid is plane, then the angle of contact of the liquid with the walls of container is
AAcute angleBobtuse angleC90∘D0∘A cork suspended from the bottom of a container filled with water with a string as shown in figure. If the container accelerates in a horizontal direction towards right, which one is correct?
 AInclination of string with vertical is tan−1 (a/g) towards leftBInclination of the string with is tan−1 (a/g) towards rightCInclination of the string with vertical is [π/2−tan−1(a/g)] towards leftDInclination of the string with vertical is [π/2−tan−1(a/g)] towards right
AInclination of string with vertical is tan−1 (a/g) towards leftBInclination of the string with is tan−1 (a/g) towards rightCInclination of the string with vertical is [π/2−tan−1(a/g)] towards leftDInclination of the string with vertical is [π/2−tan−1(a/g)] towards right
Pendulum Is Accelerating Horizontally
View SolutionWater is filled in a container having square base (2m×2m).Height of water in the container is h=2m . Now the container is tilted so that its base makes an angle of 30∘ with the horizontal.The tilted container is accelerated to right with a horizontal acceleration of a=2ms2 .Now the water surface makes an angle a with horizontal. If the value of α is tan−1(1x) ,then what is the value of x(g=10ms2)?
View SolutionPressure variation in liquid in accelerated container
View SolutionLiquid in accelerated container Problems
View SolutionA vessel filled with water is moving horizontally with constant acceleration w. What shape will the surface of the liquid have?
View Solution