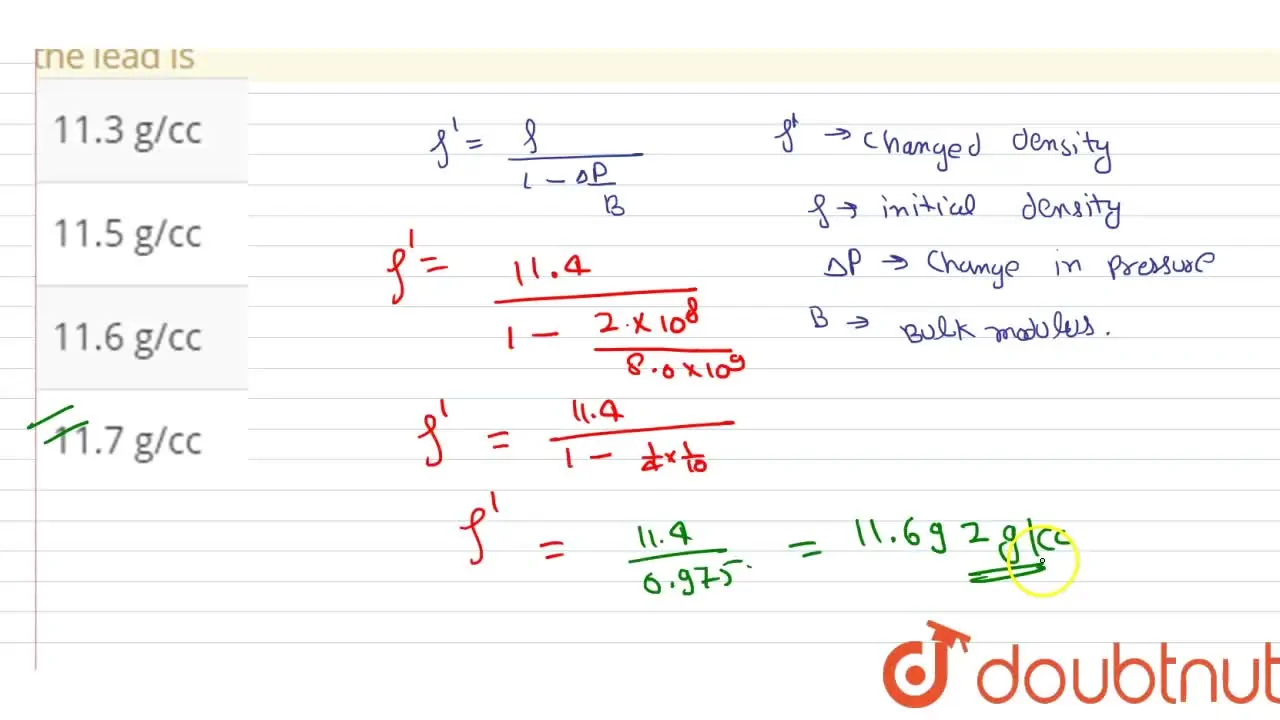
If the Bulk modulus of lead is 8.0×109N/m2 and the initial density of the lead is 11.4g/cc, then under the pressure of 2.0×108N/m2, the density of the lead is
11.3 g/cc
11.5 g/cc
11.6 g/cc
11.7 g/cc
The correct Answer is:D
Step by step video, text & image solution for If the Bulk modulus of lead is 8.0xx10^(9)N//m^(2) and the initial density of the lead is 11.4g//"cc", then under the pressure of 2.0xx10^(8)N//m^(2), the density of the lead is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - AAKASH INSTITUTECHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Assignment (SECTION - B)15videos
MECHANICAL PROPERTIES OF SOLIDS
- BOOK - AAKASH INSTITUTECHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Assignment (SECTION - C)2videos
MECHANICAL PROPERTIES OF SOLIDS
- BOOK - AAKASH INSTITUTECHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Try Yourself32videos
MECHANICAL PROPERTIES OF SOLIDS
- BOOK - AAKASH INSTITUTECHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - SECTION - J9videos
MECHANICAL PROPERTIES OF FLUIDS
- BOOK - AAKASH INSTITUTECHAPTER - Mock test 03EXERCISE - EXAMPLE37videos
Mock test 03
Similar Questions
The bulk modulus of water is 2.3×109N/m2 its compressibility is
View SolutionBulk modulus of metal is 1010N/m2 and its density is 11g/cm3. The density of this metal under a pressure of 20000N/cm2 will be g/cm3
View SolutionThe bulk modulus of a metal is 8×109Nm−2 and its density is 11 g cm−2. The density of this metal under a pressure of 20000 N cm−2 will be (in g cm−3)
View SolutionThe bulk modulus of water is 2.0×109N/m2. The pressure required to increase the density of water by 0.1% is
View SolutionThe bulk modulus of a gas is 6×103N/m2. The additional pressure needed to reduce the volume of the gas by 10% is
View SolutionAssertion : A given mass of a gas is subjected to an external pressure of 0.5×1010N/m2 , the bulk modulus of the gas is 0.5×1010N/m2 . The ratio of the density of the gas before and after applying the external pressure is 1:1
Reason : Pressure is inversely proportional to density of gas and bulk modulus is inversely proportional to change in volumeView SolutionThe bulk modulus of water is 2.1×109Nm−2. The pressure required ot increase the density of water by 0.1% is
View SolutionThe bulk modulus of a gas is 6×103N/m2. The additional pressure needed to reduce the volume of the liquid by 10% is
View SolutionThe bulk modulus of a liquid is 6×103N/m2. What is the additional pressure required to reduce the volume of the liquid by 10% ?
View SolutionFor a steel rod, the Young's modulus of elasticity is 2.9×1011N/m2, and the density of steel is 8×103kg/m3.What is the velocity of longitudinal waves in steel?
View Solution