A cubical block of side 'a' is held at rest, against a rough vertical wall by applying a force F acting along the centre. The mass of the block is m. Taking acceleration due to gravity as 'g' , determine
(i) The minimum coefficient of friction between the block and wall
(ii) The torque by normal reaction about the centre of mass
The situation is shown in the figure.
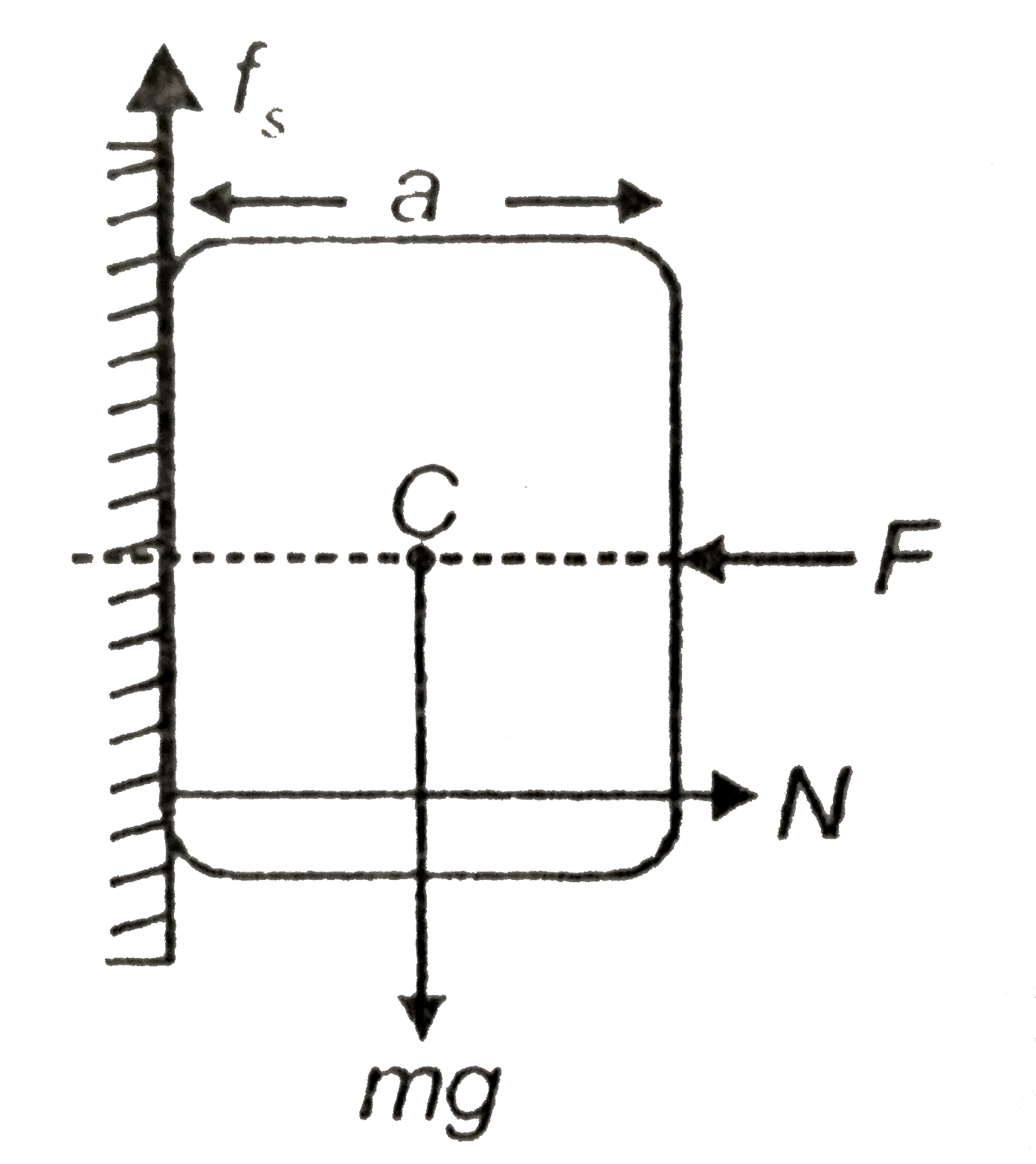
Let C be the cenre. For equilirbium of the block
∑Fx=0, ∑Fy=0, ∑τ=0
⇒F−N=0, fs=mg, fs×a2+τN=0
Here, clockwise torque has been taken as negative.
Note: Normal reaction due to the wall N, does not pass through the centre C.
Now, N=F
fs=mg
(i) As fs≤μN
⇒mg≤μF
or μ≥mgF
(ii) −fs(a)2+τN=0
τN=fs×a2=mg.a2 (in counter clockwise sense)
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - AAKASH INSTITUTECHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Illustration
4 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - AAKASH INSTITUTECHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Assignment (Section - A) Objective Type Questions (One option is correct)
62 Videos
- SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITSBOOK - AAKASH INSTITUTECHAPTER - SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITSEXERCISE - Assignment (Section-D (Assertion and reason))
5 Videos
- TEST 1BOOK - AAKASH INSTITUTECHAPTER - TEST 1EXERCISE - EXERCISE
9 Videos
Similar Questions
A block of mass M is held against a rough vertical wall by pressing it with a finger . If the coefficient of friction between the block and the wall is μ and the acceleration due to gravity is g , calculate the minimum force required to be applied by the finger to hold the block against the wall.
View SolutionA block of mass M is held against a rough vertical wall by pressing it with a finger . If the coefficient of friction between the block and the wall is μ and the acceleration due to gravity is g , calculate the minimum force required to be applied by the finger to hold the block against the wall.
View Solution
Knowledge Check
A block of mass 1 kg is held at rest against a rough vertical surface by pushing by a force F horizontally. The coefficient of friction is 0.5. When
 AF = 40 N, friction on the block is 20 N.BF = 30 N, friction on the block is 10 N.CC = 20 N, friction on the block is 10 N.DMinimum value of force F to keep block at rest is 20 N.
AF = 40 N, friction on the block is 20 N.BF = 30 N, friction on the block is 10 N.CC = 20 N, friction on the block is 10 N.DMinimum value of force F to keep block at rest is 20 N.A block of mass 'm' is held stationary against a rough wall by applying a force F as shown. Which one of the following statement is incorrect ?
 AFriction force f=mgBNormal reaction N=FCF will not produce a torqueDN will not produce any torque
AFriction force f=mgBNormal reaction N=FCF will not produce a torqueDN will not produce any torqueA block weighs W is held against a vertical wall by applying a horizontal force F. The minimum value of F needed to hold the block is
Aless tha WBEqual to WCGreater than WDData is insufficient
A block of mass M is held against a rough vertical wall by pressing it with a finger . If the coefficient of friction between the block and the wall is μ and the acceleration due to gravity is g , calculate the minimum force required to be applied by the finger to hold the block against the wall.
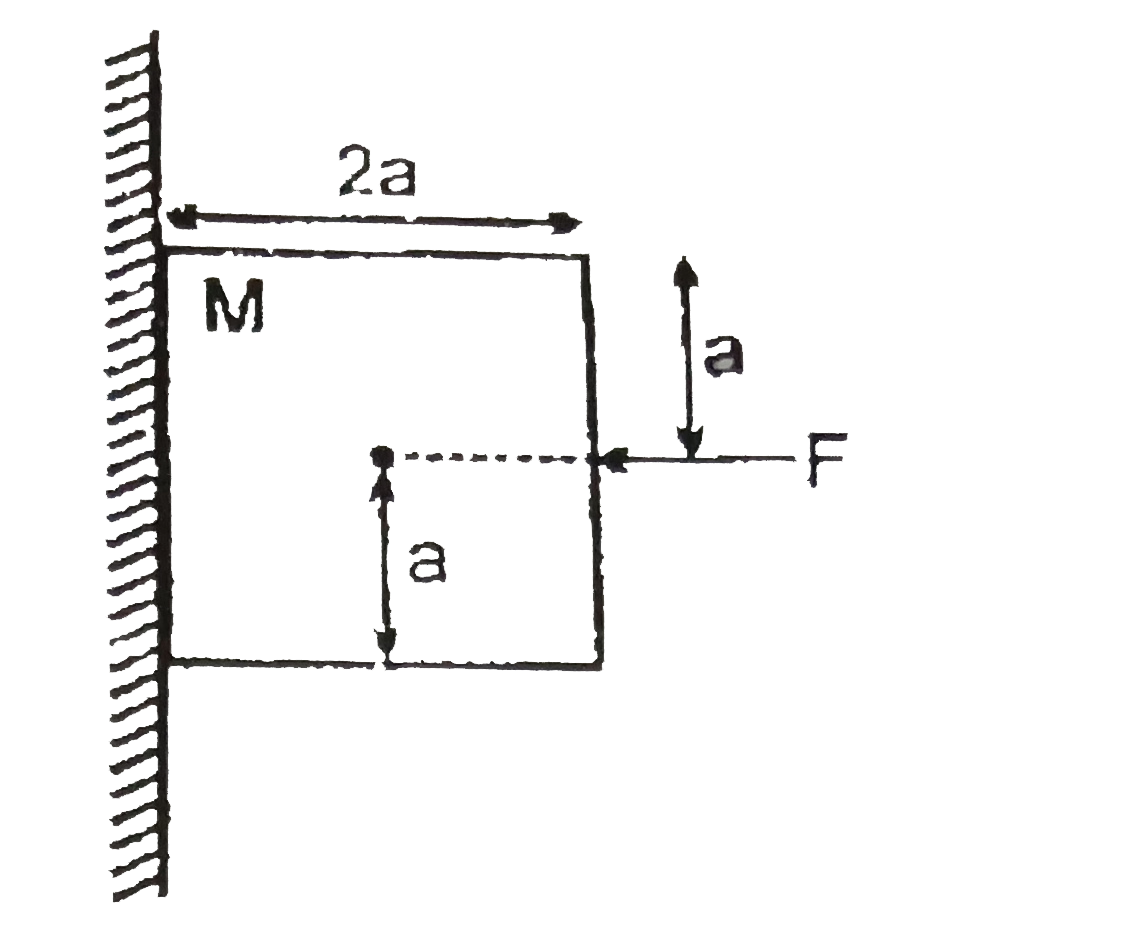
View SolutionIn the figure shown, a cubical block is held stationary against a rough wall by applying a force F, then. Incorrect statement among the following is :
View SolutionA rectangular block is held against a vertical wall by applying a force of 200N normal to the wall. If the frictional froce just prevents the block from sliding down the wall, what is the mass of the block? (The coefficient of static friction between the block and the wall is 0.49)
View SolutionA block of weight W is held against a vertical wall by applying a horizontal force of 75N . The surface3 of the wall is rough. Now (conside μ<1)
View SolutionA block of mass M is held against a rough vertical wall by pressing it with a finger. If the coefficient of friction between the block and the wall is μ and the acceleration due to gravity is g, what is the minimum force required to be applied by the finger to hold the block against the wall?
View Solution