A particle is suspended vertically from a point O by an inextensible mass less string of length L. A vertical line AB is at a distance of from O as shown in figure. The particle is given a horizontal velocity u. At some point, its motion ceases to be circular and eventually the object passes through the line AB. At the instant of crossing AB, its velocity is horizontal. Find u.
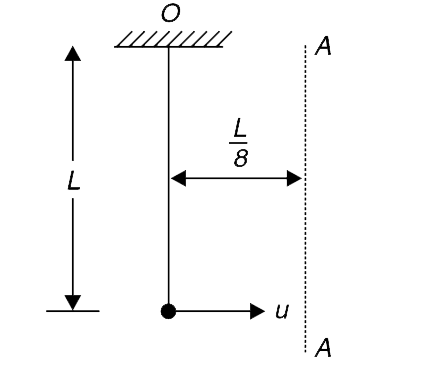
Let its circular motion cases at upper half circle at an angle with horzontal as shown in figure. Also it cannot cases in lower half circle, because in lower half circle, its can velocity cannot be horzontal while passing through AB, F.B.D at 'P' w.r.t. non-inertial from
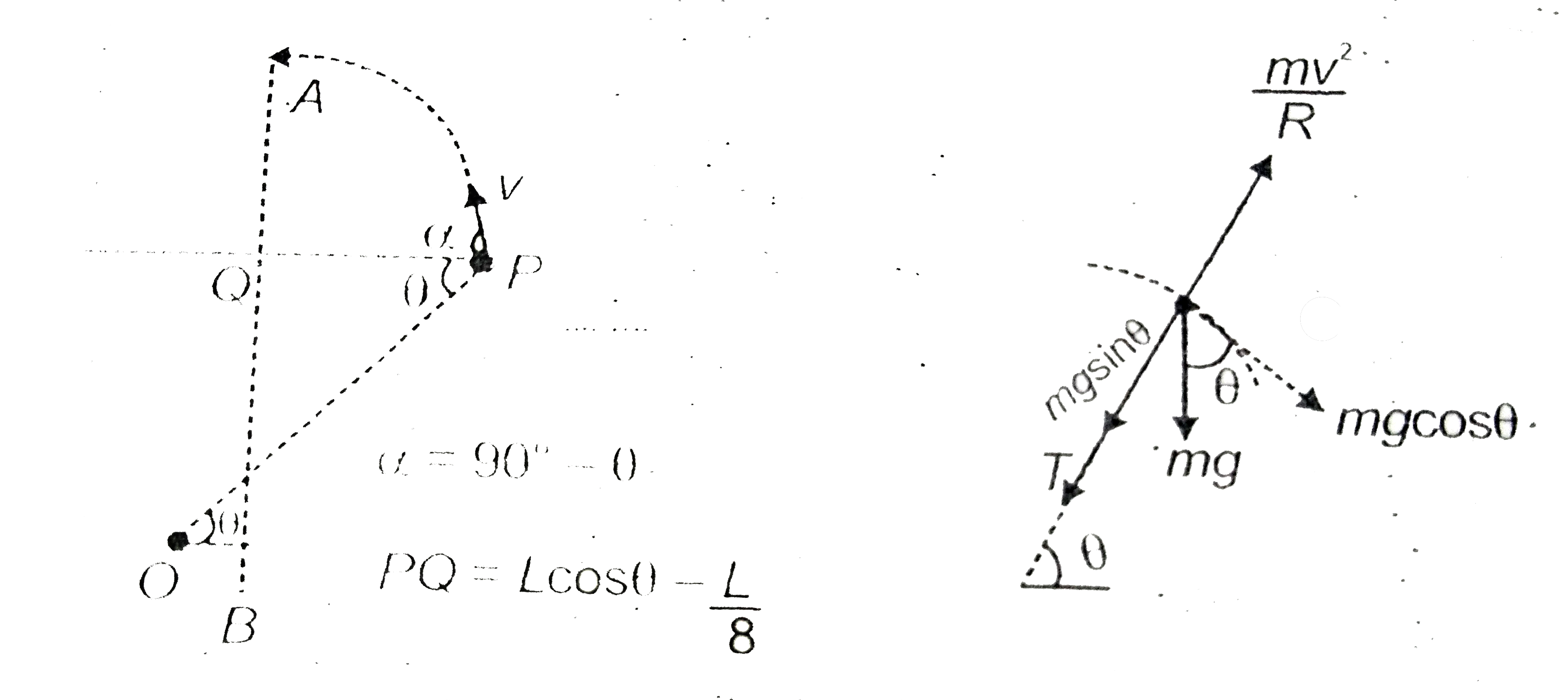
circuilar motion cases, its tension becomes zero.
Now, applying coservation of mechanical energy between bottom most point and at P. [Assumign bottom most point as fererence]
From equation (1),
The motion of particle after P is projectile and when it passes through line AB, then its becomes horzontal, it means at AB line it will be at its maximum height, from the given diagram.

From equation (2),
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTECHAPTER - WORK, ENERGY AND POWEREXERCISE - IILUSTRATION
2 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTECHAPTER - WORK, ENERGY AND POWEREXERCISE - TRY YOURSELF
95 Videos
- WAVESBOOK - AAKASH INSTITUTECHAPTER - WAVESEXERCISE - ASSIGNMENT ( SECTION-D ( Assertion - Reason Type Questions ))
12 Videos
Similar Questions
Two point masses m are connected the light rod of length l and it is free to rotate in vertical plane as shown in figure. Calculate the minimum horizontal velocity is given to mass so that it completes the circular motion in vertical lane.
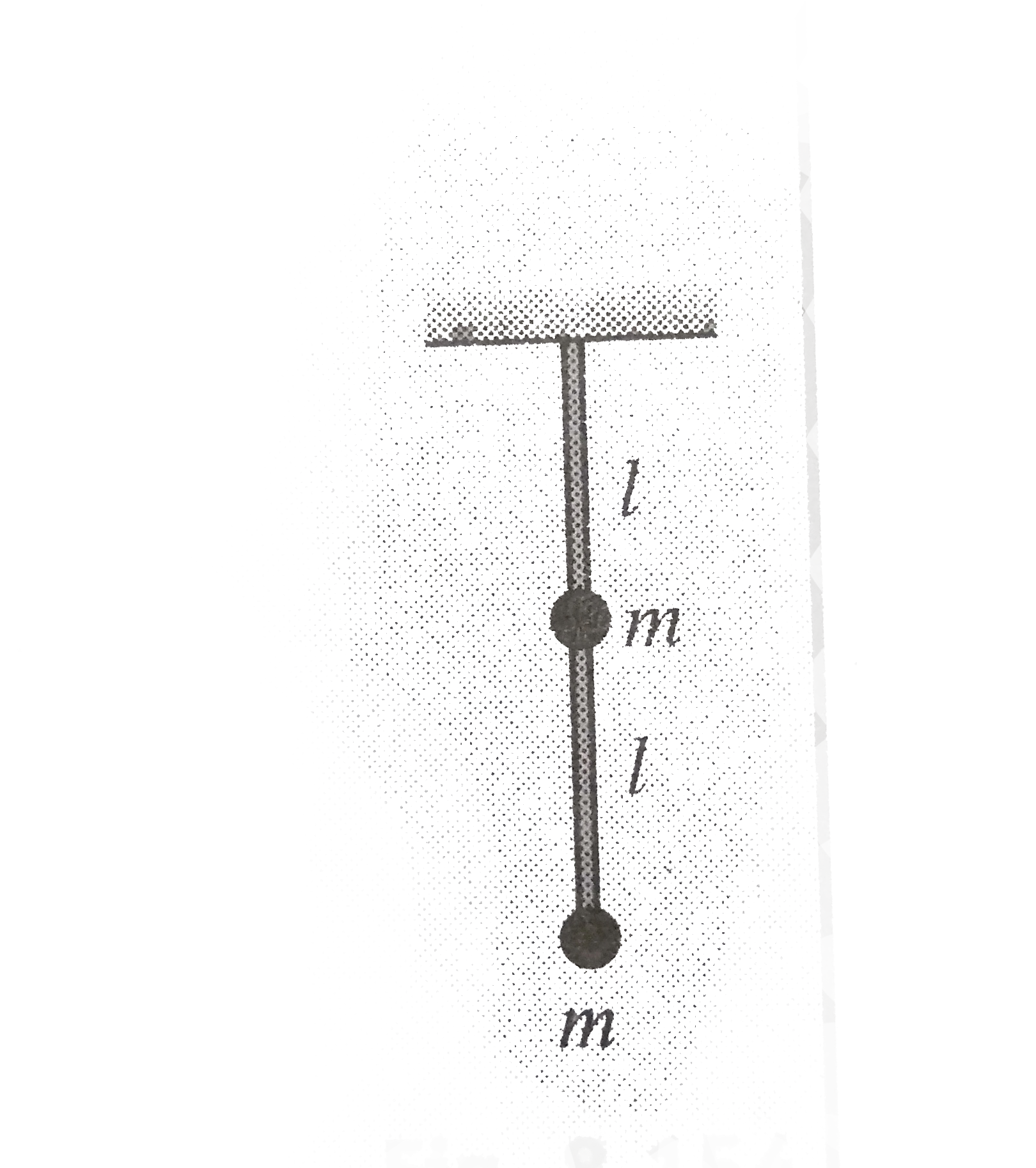
View SolutionA particle of mass m is suspended from a ceiling through a string of length L. The particle moves in a horizontal circle of radius r such that r =L√2. The speed of particle will be :
View Solution
Knowledge Check
As shown in figure, a particle of mass m is performing vertical circular motion. The velocity of the particle is increased, then at which point will the string break?
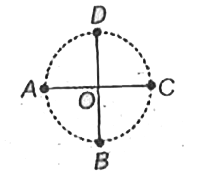 AABBCCDD
AABBCCDDA particle of mass m is suspended from the ceiling through a string of length L. The particle moves in a horizontal circle of radius r. The speed of the particle is
Arg√L2−r2Br√g(L2−r2)14Cr√g(L2−r2)12DmgL(L2−r2)12A particle of mass m is suspended from point O and undergoes circular motion in horizontal plane as conical pendulum as shown in figure.
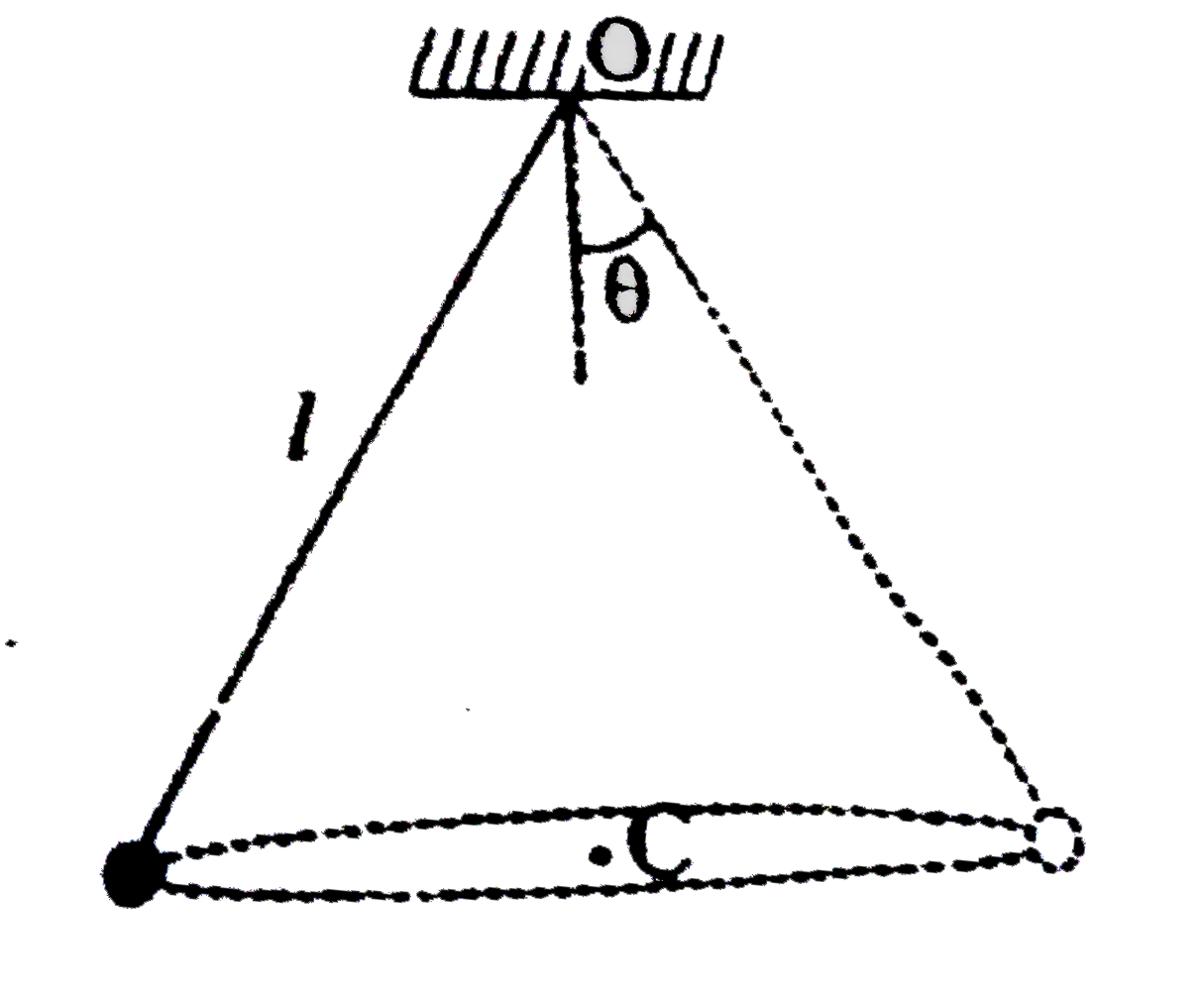 AAngular momentum of particle about point of suspension does not remains constant.BAngular momentum of particle about centre of circle remains constant.CAverage force during half rotation is 2mgtanthethaπDAverage torque about axis OC during half rotation is zero
AAngular momentum of particle about point of suspension does not remains constant.BAngular momentum of particle about centre of circle remains constant.CAverage force during half rotation is 2mgtanthethaπDAverage torque about axis OC during half rotation is zero
A particle of mass m is suspended from a fixed point O by a string length l.At t=0, it is displaced from its equilibrium position and released.If T is the tension in the string with time t, then the correct graph is
View SolutionA particle suspended from a fixed point, by a light inextensible thread of length L is projected horizontally from its lowest position with velocity 7gL . The thread will slack after swinging through an angle , such that equal
View SolutionA particle of mass m is attached to one end of a light inextensible string and the other end of the string is fixed in vertical plane as shown. Particle is given a horizontal velocity u=√52gl
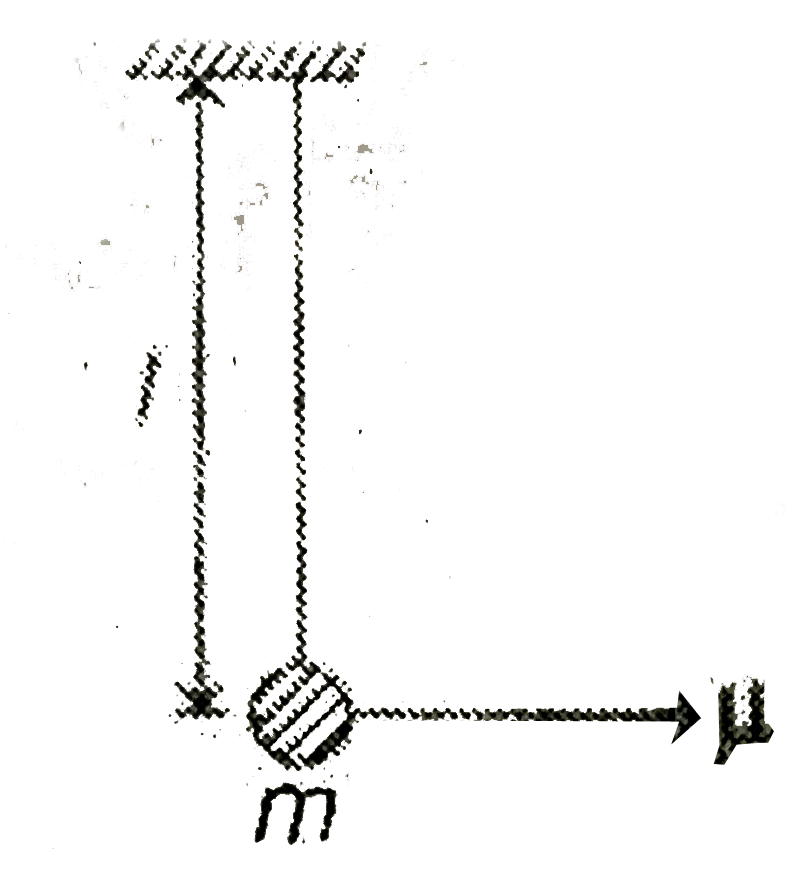
The tension in the string at an instant when acceleration of the particle is horizontal and √3g is
View SolutionA particle of mass m is attached to one end of a light inextensible string and the other end of the string is fixed in vertical plane as shown. Particle is given a horizontal velocity u=√52gl

The maximum angle made by the string with downward vertical is
View SolutionA point mass m is connected with ideal inextensible string of length l and other end of string is tied at point O as shown in the figure. The string is made horizontal and mass is released from point A to perform vertical circular motion. There is a nail at point N such that ON =r . Find the value of r so that mass m is able to just perform complete vertical circular motion about point N.
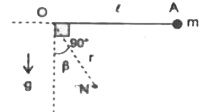
View Solution
