A dparticle of mas m moving with speed u collides elastically with a sphere of radius R and same mass at rest, at an impact parameter d. Find
(a) Angle between their final velocities and
(b) Magpitude of their final velocities.
As the collision is perfectly elastic and masses of both the particles are same thus their velocity vectors should b e exchanged along the line impact.
Thus v2=ucosθandv3=0
Now id we focus on the particle along there is no force on it parpendicular to the line of impact.
Thus its velocity does not change in this directin and hence.
v1=usinθ
Now, from geometry,
sinθ=dR
cosθ=√1−d2R2
⇒v2=uR√R2−d2
v1=udR
v3=0
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTECHAPTER - WORK, ENERGY AND POWEREXERCISE - IILUSTRATION
2 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTECHAPTER - WORK, ENERGY AND POWEREXERCISE - TRY YOURSELF
95 Videos
- WAVESBOOK - AAKASH INSTITUTECHAPTER - WAVESEXERCISE - ASSIGNMENT ( SECTION-D ( Assertion - Reason Type Questions ))
12 Videos
Similar Questions
A particle of mass m moving with velocity u collides elastically head on with a particle of mass 2m at rest. After collision the heavier particle moves with velocity?
View SolutionA sphere of mass m moving with velocity v collides head-on with another sphere of the same mass at rest. If the coefficient of resistitution e=1/2, then what is the ratio of final velocity of the second sphere to the intial velocity of the first sphere ?
View Solution
Knowledge Check
An object of mass M1 moving horizontally with speed u collides elastically with another object of mass M2 at rest. Select correct statement.
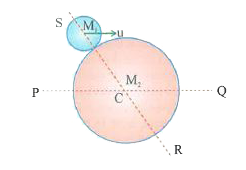 AThe momentum of system is conserved only in direction PQBMomentum of M1 is conserved in direction perpendicular to SRCMomentum of M2 will change in direction normal to CRDAll of these
AThe momentum of system is conserved only in direction PQBMomentum of M1 is conserved in direction perpendicular to SRCMomentum of M2 will change in direction normal to CRDAll of theseA body of mass m2 moving with velocity v0 collides elastically with another mass of m3 . Find % change in KE of first body
A32B96C34D80A smooth sphere of mass M moving with velocity u directly collides elastically with another sphere of mass m at rest. After collision their final velocities are V and v respectively. The value of v is
A2uMmB2μMMC2u1+mMD2u1+Mm
A particle of mass m moving with velocity v0 collides with sphere of same mass at rest , as shown. If the surface of contact is smooth and the collision is elastic, find the velocities of particle and sphere after the collision.
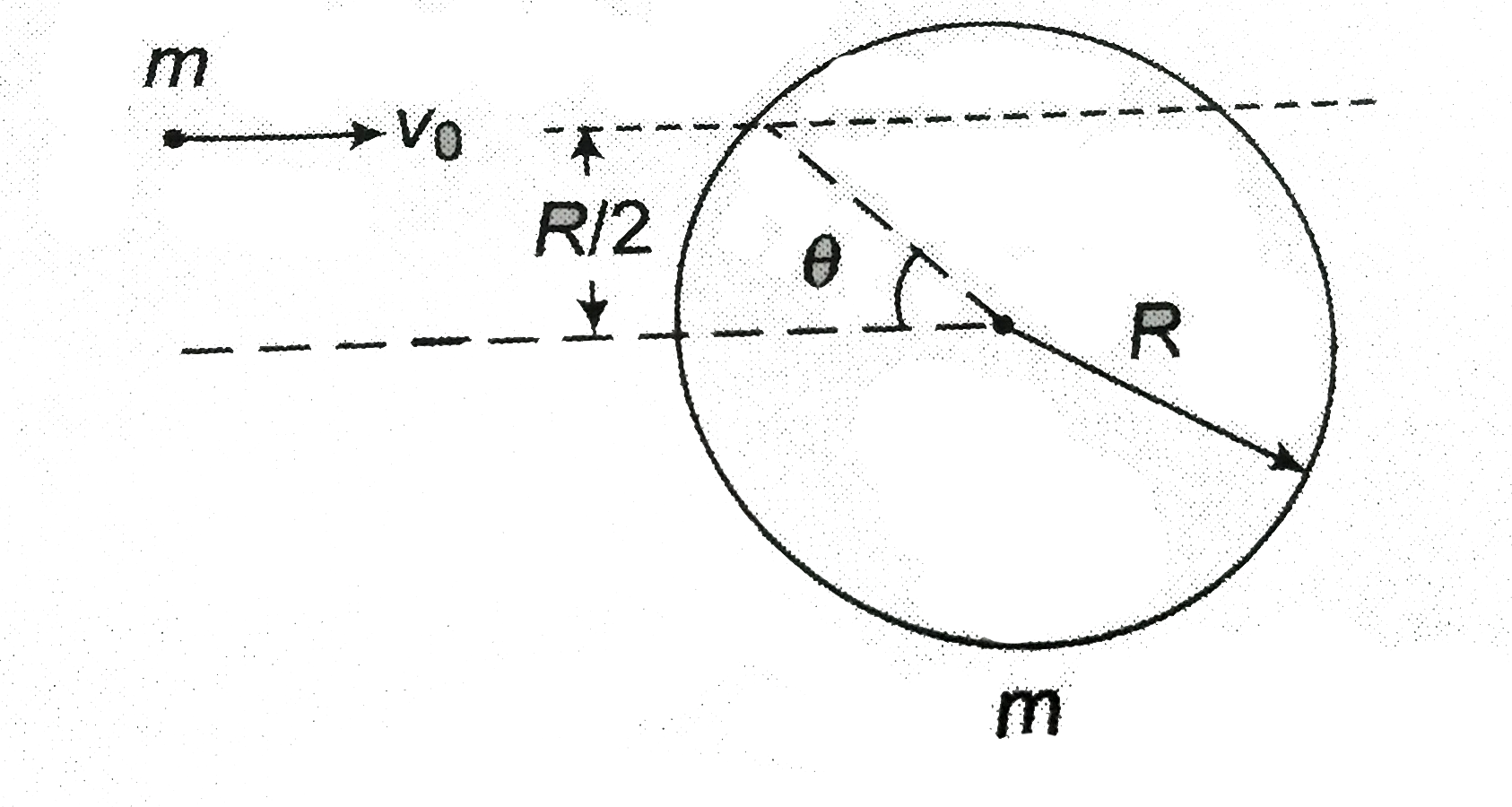
View SolutionA smooth sphere of mass M moving with velocity u directly collides elastically with another sphere of mass m at rest. After collision their final velocities are V and v respectively. The value of v is
View SolutionA body of mass m moving with velocity uˆi collides elastically with a body at rest of mass 3m and then the final velocity of body mass m is v ˆj. Find the relation between v and u
View SolutionA body of mass M moves with velocity v and collides elasticity with another body of mass m(M>>m) at rest , then the velocity of the body of mass m is
View SolutionA ball of mass m1 moving with a certain speed collides elastically with another ball of mass m2 kept at rest . If the first ball starts moving in reversed direction after the impact then
View Solution