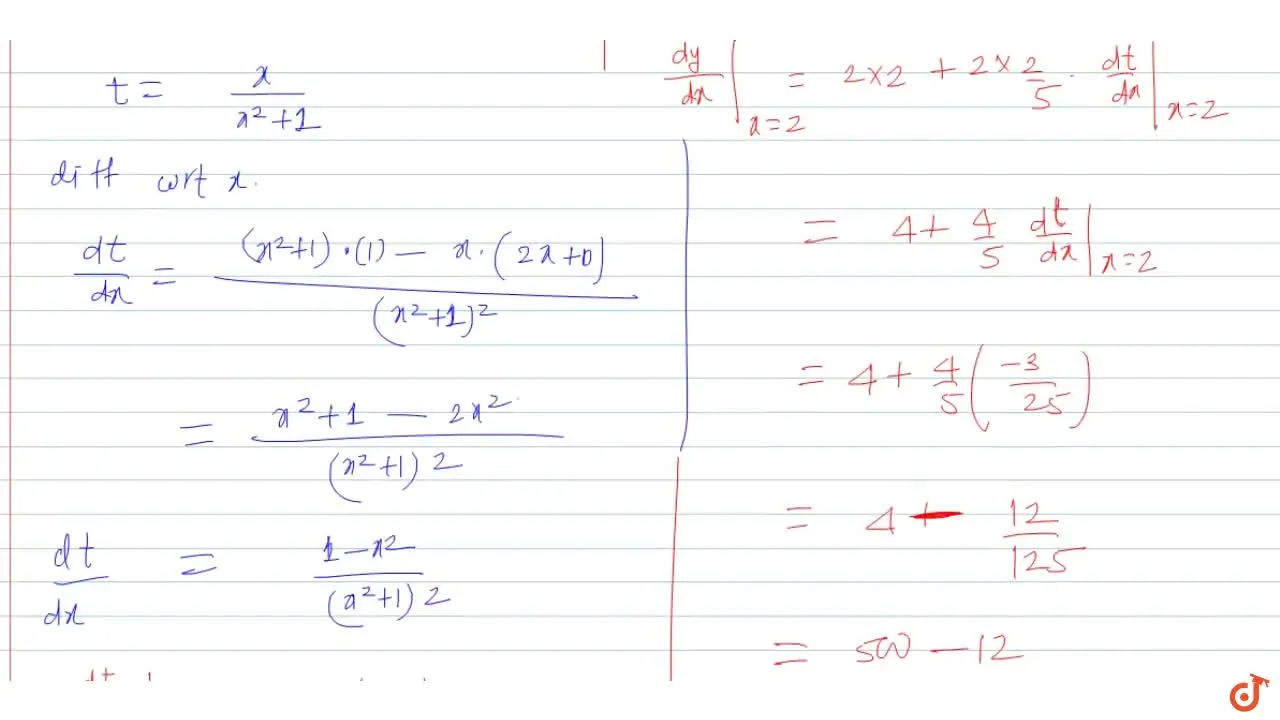
If $t (1 + x^{2}) = x and x^{2} + t^{2} = y,$ then at $x = 2$ the value of $\frac{d y}{d x}$ is equal to

$\frac{24}{5}$

$\frac{101}{125}$

$\frac{488}{155}$

$\frac{358}{125}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

Updated on:21/07/2023

If $t (1 + x^{2}) = x and x^{2} + t^{2} = y$ then at $x = 2$ the value of $\frac{d y}{d x}$ is
A $\frac{488}{125}$
B $\frac{88}{125}$
C $\frac{101}{125}$
DNone
If $x = t^{2}$ and $y = t^{3}$ , then what is the value of $\frac{d^{2} y}{{d x}^{2}}$ ?
A1
B $\frac{3}{2 t}$
C $\frac{3}{4 t}$
D $\frac{3}{2}$
If x=cos 2t and $y = {sin}^{2} t$ , then what is the value of $\frac{d^{2} y}{{d x}^{2}}$ ?
A0
Bsin(2t)
C $- cos (2 t)$
D $- \frac{1}{2}$