Solve: y4dx+2xy3dy=ydx−xdyx3y3
Video Solution
Text Solution
We can rewrite the given equation as
y4dx+2xy3dy+1xy3xdy−ydxx2=0
⇒xy7dx+2x2y6dy+d(yx)=0
⇒x2y6dx+2x3y5dy+xyd(yx)=0
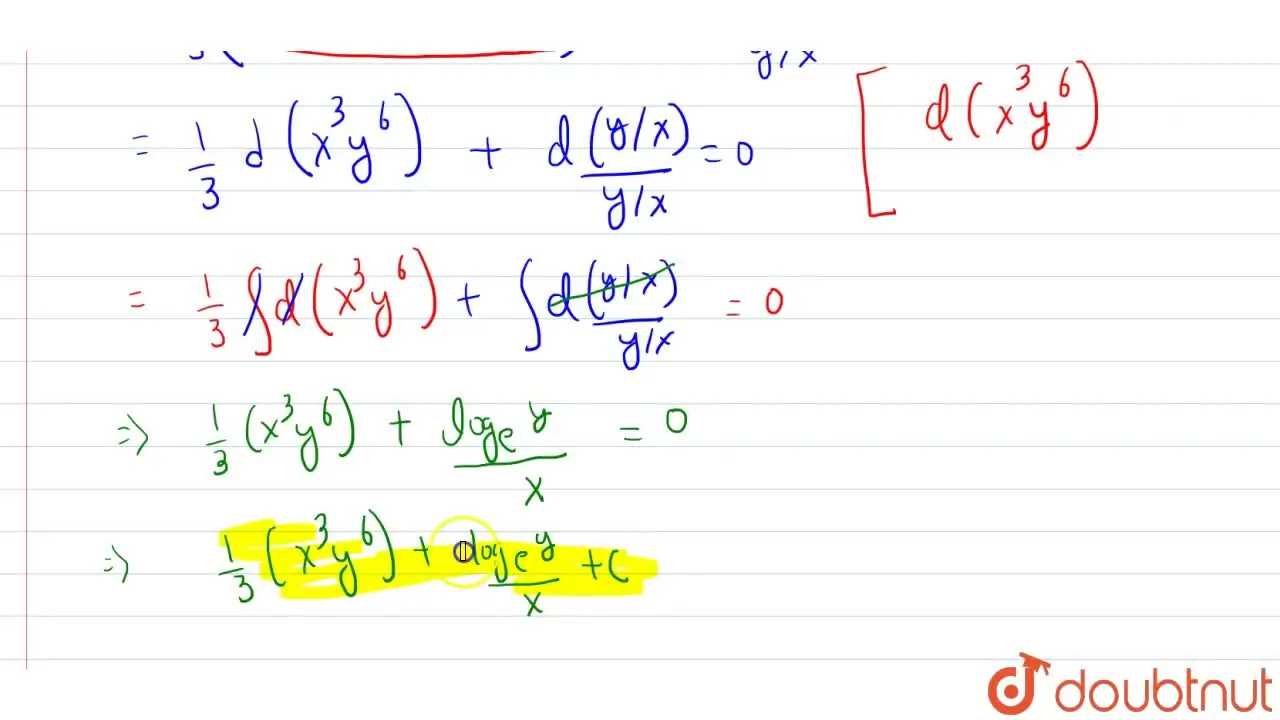
⇒13(3x2y6+6x3y5dy)+d(y/x)y/x=0
⇒13∫d(x3y6)+∫(dx/yy/x)=0
⇒x3y63+logeyx=c
|
Updated on:21/07/2023
Related Playlists
- BOOK - CENGAGECHAPTER - DIFFERENTIAL EQUATIONSEXERCISE - Exercise 10.16videos
DIFFERENTIAL EQUATIONS
- BOOK - CENGAGECHAPTER - DIFFERENTIAL EQUATIONSEXERCISE - Exercise 10.26videos
DIFFERENTIAL EQUATIONS
- BOOK - CENGAGECHAPTER - DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS EXERCISE - Exercise337videos
DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS
- BOOK - CENGAGECHAPTER - DIFFERENTIATIONEXERCISE - Numerical Value Type3videos
DIFFERENTIATION
Similar Questions
Solve: ydx+(x−y3)dy=0
View Solutiondydx=x3y3−xy
View Solution(x2y3+xy)dy=dx
View SolutionSolve: (x+2y3)dy=ydx.
View SolutionSolve: x2ydx=(x3+y3)dy=0
View SolutionSolve: y(1+xy)dx−xdy=0
View SolutionSolve : dydx=y3+2x2yx3+2xy2.
View SolutionSolve: dydx=x3y3−xy
View SolutionSolve (i) xdx+ydy+xdy−ydxx2+y2=0
(ii) y(1+xy)dx−xdy=0View SolutionSolve each of the following initial value problem: (y4−2x3y)dx+(x4−2xy3)dy=0, y(1)=1
View Solution