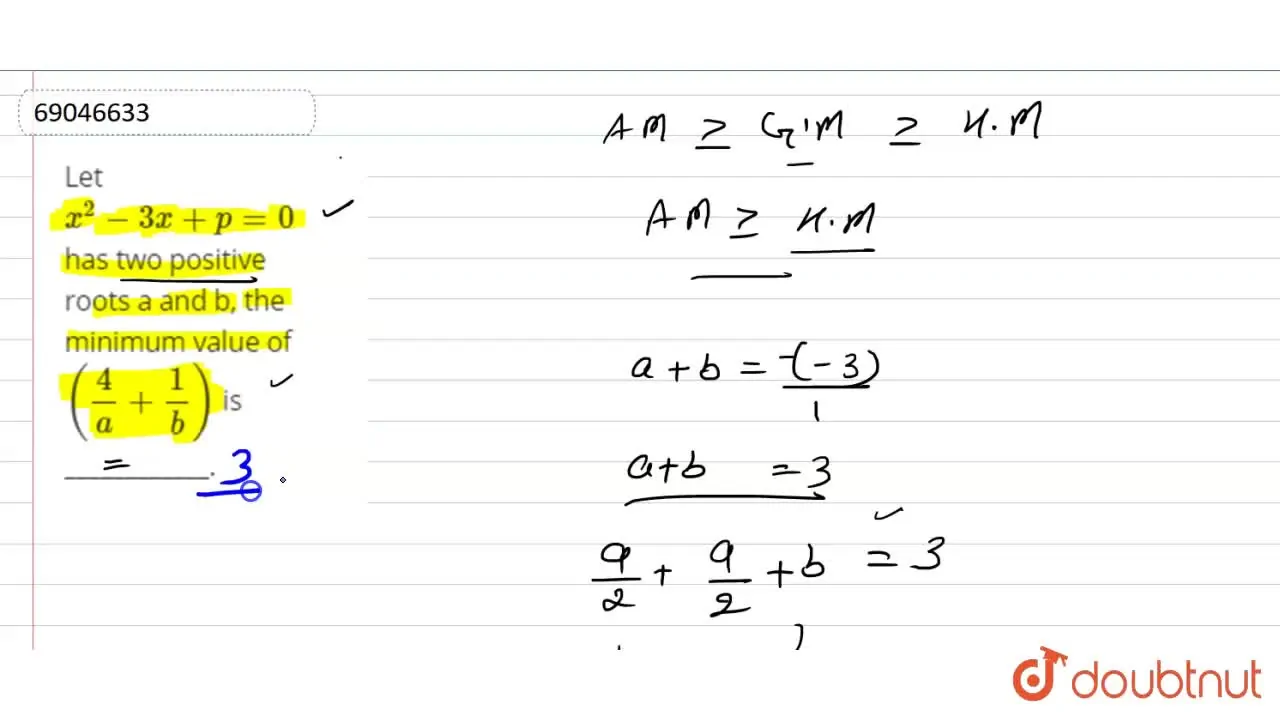
Let x2−3x+p=0 has two positive roots a and b, the minimum value of (4a+1b) is ____________.
The correct Answer is:3
a + b = 3
H.M ≥ A.M for numbe a2,a2, b. We have
32a+2a+1b≤a2+a2+63=1
∴1≥32a+2a+1bor 2a+2a+1b≥3
∴4a+1b≥3
Related Playlists
- BOOK - CENGAGECHAPTER - INEQUALITIES INVOLVING MEANS EXERCISE - Exercise (Comprehension)6videos
INEQUALITIES INVOLVING MEANS
- BOOK - CENGAGECHAPTER - INEQUALITIES AND MODULUSEXERCISE - Single correct Answer21videos
INEQUALITIES AND MODULUS
- BOOK - CENGAGECHAPTER - INTEGRALSEXERCISE - Solved Examples And Exercises324videos
INTEGRALS
Similar Questions
Let x2−3x+p=0 has two positive roots aandb , then minimum value if (4a+1b) is,
View SolutionLet x2−3x+p=0 has two positive roots a and b, then minimum value of 1a+1b is
View SolutionIfx4+px3+qx2+rx+5=0 has four positive roots,then the minimum value of pr is equal to
View SolutionLet x and y be two positive real numbers such that x+y=1. Then the minimum value of 1x+1y is
View SolutionIf x2+ax−3x−(a+2)=0 has real and distinct roots, then minimum value of (a2+1)/(a2+2) is
View SolutionThe equation 2x3−3x2+p=0 has three real roots. Then find the minimum value of p.
View SolutionIf the equation x4−4x3+ax2+bx+1=0has four positive roots, then the value of (a+ b) is :
View SolutionThe equation x3−6x2+px−8=0 has only positive real roots. The value of p is
View SolutionLet x and y be two positive real numbers such that x + y = 1. Then the minimum value of 1x+1y is-
View SolutionIf the equation x4−4x3+ax2+bx+1=0 has four positive roots, fond the values of a and b.
View Solution