A ball of mass 200g rests on a vertical post of height 20m.A bullet of mass 10g,travelling in horizontal direction,hits the centre of the ball.After collision both travels independently.The ball hits the ground at a distance 30m and the bullet at a distance of 120m from the foot of the post.The value of initial velocity of the bullet will be (If g=10ms2 ) :
400ms
360ms
60ms
120ms
The correct Answer is:B
To solve the problem step by step, we will analyze the motion of the ball and the bullet separately and apply the principles of physics, particularly projectile motion and conservation of momentum.
Step 1: Determine the time of flight for the ball
The ball falls from a height of 20 m. We can use the equation of motion for free fall:
h=12gt2
Where:
- h=20m (height)
- g=10m/s2 (acceleration due to gravity)
- t = time of flight
Rearranging the equation gives:
20=12×10×t2
20=5t2
t2=205=4
t=√4=2s
Step 2: Calculate the horizontal velocity of the ball after the collision
The ball travels a horizontal distance of 30 m while falling. We can find its horizontal velocity V using the formula:
Distance=Velocity×Time
Substituting the known values:
30=V×2
Solving for V:
V=302=15m/s
Step 3: Calculate the horizontal velocity of the bullet after the collision
The bullet travels a horizontal distance of 120 m in the same time of flight (2 seconds). We can find its horizontal velocity V′:
120=V′×2
Solving for V′:
V′=1202=60m/s
Step 4: Apply the conservation of momentum
Since the collision is elastic, we apply the conservation of momentum before and after the collision. Let u be the initial velocity of the bullet.
The total momentum before the collision is equal to the total momentum after the collision:
mb⋅u+mball⋅0=mb⋅V′+mball⋅V
Where:
- mb=10g=0.01kg (mass of the bullet)
- mball=200g=0.2kg (mass of the ball)
- V′=60m/s (velocity of the bullet after collision)
- V=15m/s (velocity of the ball after collision)
Substituting the values:
0.01u+0=0.01⋅60+0.2⋅15
Calculating the right side:
0.01u=0.6+3
0.01u=3.6
Solving for u:
u=3.60.01=360m/s
Final Answer
The initial velocity of the bullet is 360m/s.
---
The ball falls from a height of 20 m. We can use the equation of motion for free fall:
- h=20m (height)
- g=10m/s2 (acceleration due to gravity)
- t = time of flight
The ball travels a horizontal distance of 30 m while falling. We can find its horizontal velocity V using the formula:
The bullet travels a horizontal distance of 120 m in the same time of flight (2 seconds). We can find its horizontal velocity V′:
Since the collision is elastic, we apply the conservation of momentum before and after the collision. Let u be the initial velocity of the bullet.
- mb=10g=0.01kg (mass of the bullet)
- mball=200g=0.2kg (mass of the ball)
- V′=60m/s (velocity of the bullet after collision)
- V=15m/s (velocity of the ball after collision)
The initial velocity of the bullet is 360m/s.
Topper's Solved these Questions
Similar Questions
A ball of mass 0.2 kg rests on a vertical post of height 5m. A bullet of mass 0.01 kg, travelling with a velocity v m/s in a horizontal direction, hits the centre of the ball. After the collision, the ball and ullet travel independently. The ball hits the ground at a distance of 20 m and the bullet at a distance of 100m from the foot of the post. the initial velocity v of the bullet is
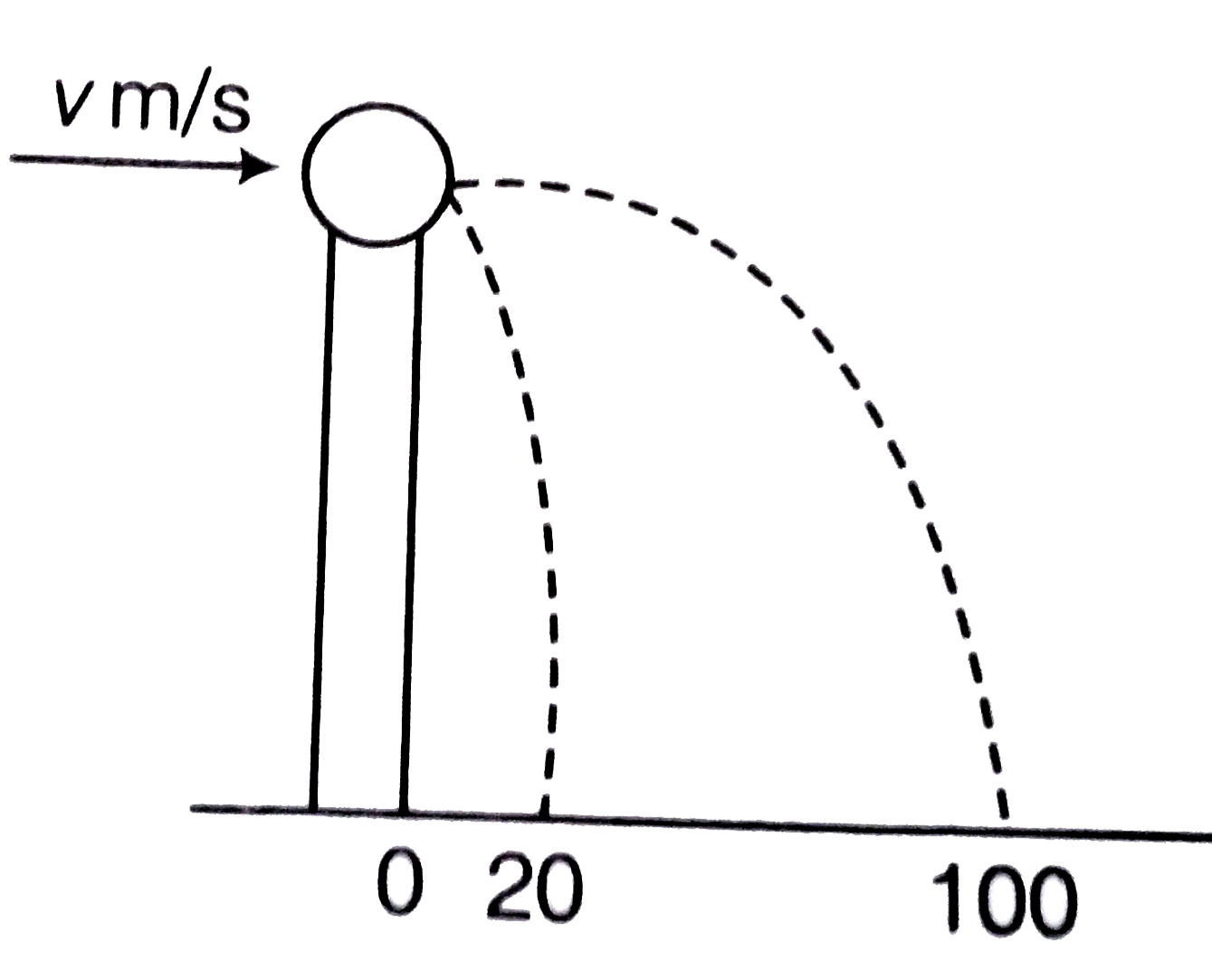
View SolutionA ball is thrown vertically downward with a velocity of 20m/s from the top of a tower,It hits the ground after some time with a velocity of 80m/s .The height of the tower is : (g=10mg2)
View Solution
Knowledge Check
A ball of mass 0.2 kg rests on a vertical post of height 5 m. A bullet of mass 0.01 kg, travelling with a velocity Vm/s in a horizontal direction, hits the centre of the ball. After the collision, the ball and bullet travel independently. The ball hits the ground at a distance of 20 m and the bullet at a distance of 100 m from the foot of the post. The velocity V of the bullet is
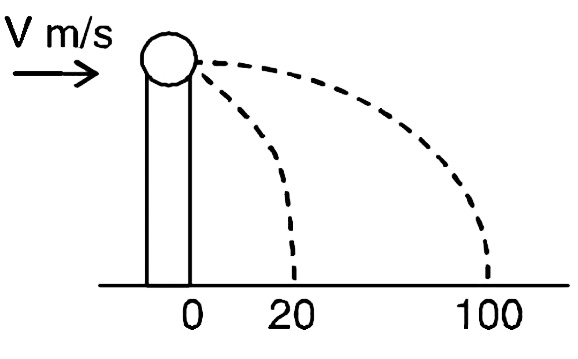 A250m/sB250√2m/sC400m/sD500m/s
A250m/sB250√2m/sC400m/sD500m/sIf a ball is thrown vertically upwards with a velocity of 40m/s, then velocity of the ball after 2s will be (g=10m/s2)
A15m/sB20m/sC25m/sD28m/sA bullet is fired from a rifle that is held 1.6 m above the ground in a horizontal position. The initial speed of the bullet is 1100 m/s. Find the time it takes for the bullet to strike the ground and the horizontal distance traveled by the bullet.
A0.24 s, 693 mB0.57 s, 630 mC0.32 s, 440 mD0.63 s, 352 m
A small ball with mass M=0.2kg rests on top of a vertical column with height h=5m. A bullet with mass m =0.01kg, moving with velocity v0=500m/s, passes horizontally through the center of the ball. The ball reaches the ground at a horizontal distance s=20m from the column. Where does the bullet reach the ground? What part of the kinetic energy of the bullet was converted into heat when the bullet passed through the ball? Neglect resistance of the air. Assume that g=10m/s2.
View SolutionA ball is thrown vertically upwards from the top of a tower with a velocity of 10m/s. If the ball falls on the ground after 5 seconds, the height of the tower will be? (use g=10m/s2)
View SolutionA ball is thrown from the top of a tower with an initial velocity of 10 m//s at an angle of 30∘above the horizontal. It hits the ground at a distance of 17.3 m from the base of the tower. The height of the tower (g=10m/s2) will be
View SolutionA ball is dropped from a height of 80 in. The distance travelled by it in the fourth second will be_____ (take g=10m/s2)
View SolutionA ball is thrown vertically downward with a velocity of 20m/s from the top of a tower,It hits the ground after some time with a velocity of 80m/s .The height of the tower is : (g=10mg2)
View Solution
