If cos40∘=xandcosθ=1−2x2, then the possible values of θ lying between 0∘ and 360∘ is
A
100∘and260∘
B
80∘and280∘
C
280∘and110∘
D
110∘and260∘
Video Solution
Text Solution
The correct Answer is:A
|
Answer
Step by step video & text solution for If cos40^(@)=xandcostheta=1-2x^(2), then the possible values of theta lying between 0^(@) and 360^(@) is by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
If K=cos20∘ and cosx=2K2−1, then the possible value of x between 0∘ and 360∘ are
A140∘B40∘,140∘C40∘,320∘D50∘,130∘The value of θ lying between 0 and π2 and satisfying the equation
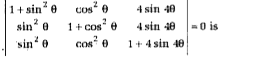 A7π24or11π24B5π24C3π24Dnone of these
A7π24or11π24B5π24C3π24Dnone of theseIf sin32∘=kandcosx=1−2k2,α,β are the values of x between 0∘ and 360∘ with α<β, then
Aα+β=180∘Bβ−α=200∘Cβ=4α+40∘Dβ=5α−20∘
If cos θ=12(x+1x), what is the value of cos 2θ ?
View Solutionlf sin(x−y)−cos(x+y)=12 then the values of x&y lying between 0andπ are given by
View SolutionIf cosθ=−12and0∘<θ<360∘, then the values of θ are
View SolutionIf cosθ=−12 and 0<θ<360∘, then the value of θ are
View SolutionIf the eccentricity of the hyperbola x2(1+sinθ)2−y2cos2θ=1 is 2√3, then the sum of all the possible values of θ is (where, θ∈(0,π))
View Solution

