Question

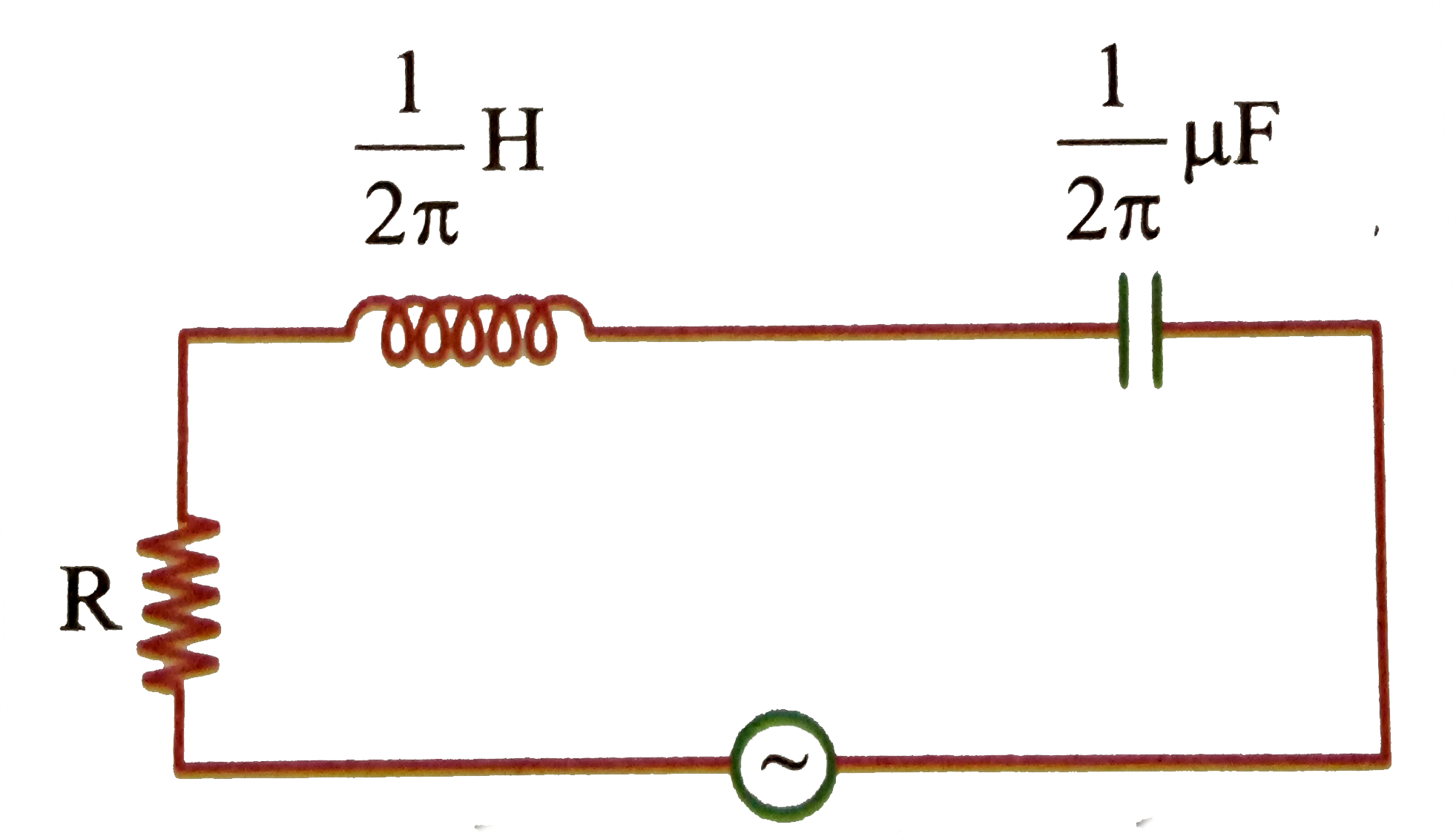
In an LCR series resonant circuit which one of the following cannot be the expression for the Q-factor

Options

A. `(omega_(r))L/(R )`
B. `(1)/(omega CR)`
C. `sqrt((L)/(C )) (1)/(R )`
D. `(R )/(LC)`

Answer

D

Explanation

To determine which expression cannot be the Q-factor in an LCR series resonant circuit, we will analyze the Q-factor and its possible expressions step by step.

1. Understanding the Q-factor:
The Q-factor (Quality factor) of a resonant circuit is a dimensionless parameter that describes how underdamped the circuit is, and it is defined as the ratio of the reactive power to the resistive power in the circuit.

2. Basic Formula for Q-factor:
The Q-factor can be expressed in terms of the inductive reactance (XL) and the resistance (R) of the circuit:
\( Q = \frac{X_L}{R} \)
where \(X_L = \omega L\) (with \(\omega\) being the angular frequency).

3. Resonance Condition:
At resonance in an LCR circuit, the inductive reactance equals the capacitive reactance:
\( X_L = X_C \quad \Rightarrow \quad \omega L = \frac{1}{\omega C} \)
This leads to the resonant frequency:
\( \omega_0 = \frac{1}{\sqrt{LC}} \)

4. Alternative Expressions for Q-factor:
Using the resonance condition, we can express the Q-factor in different forms:
- Substituting \(X_L\) into the Q-factor formula:
\( Q = \frac{\omega L}{R} \)
- Another form can be derived from the relationship between \(X_C\) and \(R\):
\( Q = \frac{X_C}{R} = \frac{1/\omega C}{R} = \frac{1}{\omega CR} \)

5. Evaluating Given Options:
Now, we will check the possible expressions for the Q-factor:
- Option 1: \( \frac{\omega L}{R} \) - This is valid.
- Option 2: \( \frac{1}{\omega CR} \) - This is also valid.
- Option 3: \( \frac{1}{\omega C} R \) - This can be rearranged to show it is valid.
- Option 4: \( \frac{R}{LC} \) - This does not fit any derived expression for the Q-factor.

6. Conclusion:
The expression that cannot be the Q-factor in an LCR series resonant circuit is:
\( \text{Option 4: } \frac{R}{LC} \)