A charged particle ( mass m and charge q) moves along X axis with velocity V0 . When it passes through the origin it enters a region having uniform electric field →E=−Eˆj which extends upto x=d . Equation of path of electron in the region . x>d is :
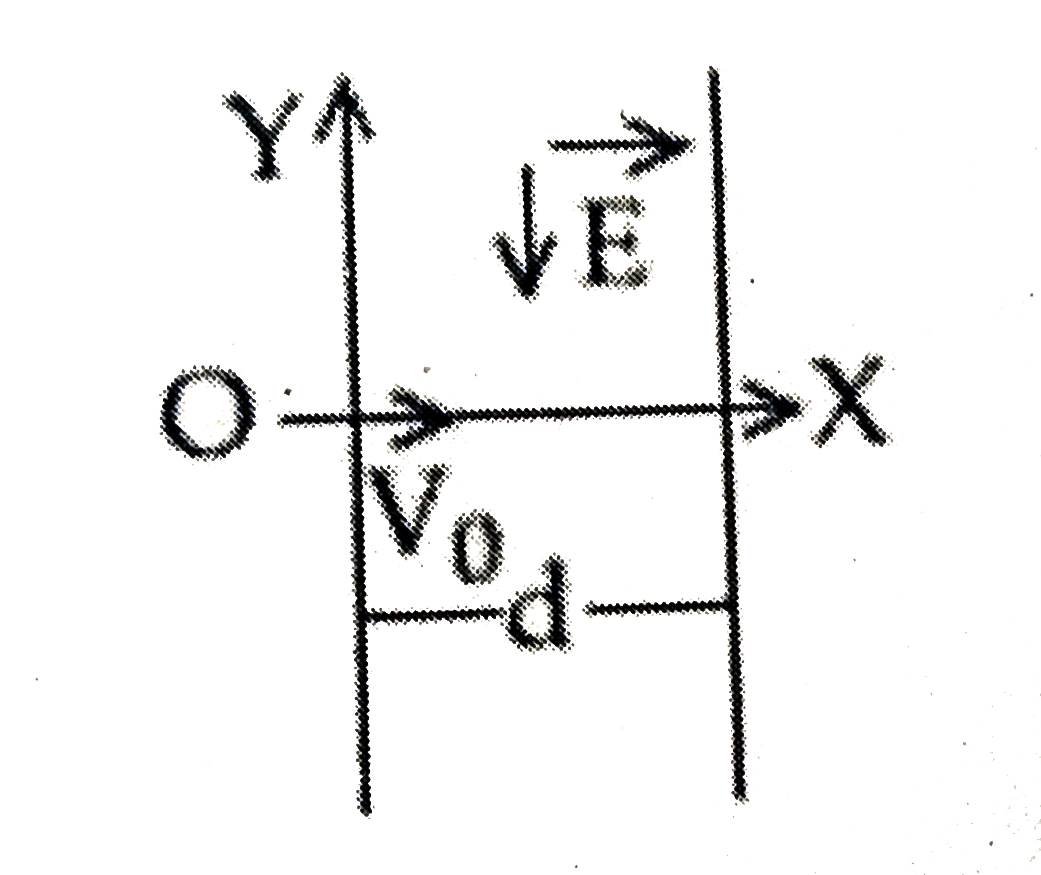
y=qEdmv20(x−d)
y=qEdmv20(d2−x)
y=qEdmv20x
y=qEd2mv20x
The correct Answer is:B
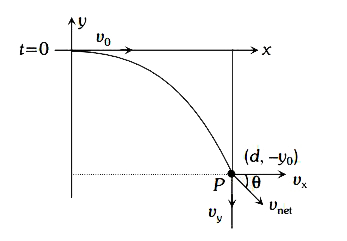
Let particle have charge q and mass .m.
Solve for (a,m) mathematically
Fx=0,ax=0,(v)x = constant
Time taken to reach at .P. = dv0=t0 (let) ...(i)
(along -y axis), y0=0+12.qEm.t20 ...(ii)
vx=v0
(along -y axis), v = u + at
tanθ=vyvx=qEt0m.v0 (t0=dv0)
tanθ=qEdm.v20
Slope =−qEdmv20
Now we have to find eqn of straight line whose slope is −qEdmv20 and it pass through point →(d,−y0)
Because after x>d
No electric field = Fnet=0,→v = const.
y=mx+c(m=qEdmv20,(d−y0))
−y0=−qEdmv20.d+c⇒c=−y0+qEd2mv20
Put the value -
y=−qEdmv20x−y0+qEd2mv20
y0=12.qEm(dv0)2=12qEd2mv20
y=−qEdmv20−12qEd2mv20+qEd2mv20
y=−qEdmv20x+12qEd2mv20
y=qEdmv20(d2−x)
Similar Questions
A particle of mass m and charge q moves with a constant velocity v along the positive x direction. It enters a region containing a uniform magnetic field B directed along the negative z direction, extending from x = a to x = b. The minimum value of v required so that the particle can just enter the region x lt b is
View SolutionA charged particle of mass m and charge q is accelerated through a potential difference of V volts. It enters a region of uniform magnetic field which is directed perpendicular to the direction of motion of the particle. Find the radius of circular path moved by the particle in magnetic field.
View Solution
Knowledge Check
A particle of mass m and charge Q moving with a velocity v enters a region on uniform field of induction B Then its path in the region is s
AAways circularBcircular, If v×B=0Ccircular,If v.B=0DNone of theseA particle of mass m and charge q moves with a constant velocity v along the positive x direction. It enters a region containing a uniform magnetic field B directed along the negative z direction, extending from x=a to x=b. The minimum value of v required so that the particle can just enter the region x>b is
AqbBmBq(b−a)BmCqaBmDq(b+a)B2mA particle of the mass m and cgharge q moves with a constant velocity v along the positive x−direction.Itentersaregionconta∈∈gauniformmag≠ticfieldBdirectedalongtheminiμmvalueofvrequiredsotˆthepartic≤canjustentertheregion xgtb is
AqbBmBq(b−A)BmCqaBmDq(b+a)B2m
A particle of mass m and charge q moving with velocity v enters the region of uniform magnetic field at right angle to the direction of its motion. How does its kinetic energy get affected?
View SolutionA charged particle, having a charge q, is moving with a speed v along the x-axis. It enters a region of space where an electric field →E(=Eˆj) and a magnetic field →B are both present. The particle, on emerging from this region, is observed to be moving along the x-axis only. Obtain an expression for the magnitude of →B in terms of v and E. Give the direction of →B.
View SolutionA particle having mass m and charge q is released from the origin in a region in which electric field and magnetic field are ginen by
B=+B0ˆj and →E=+E0ˆi.
Find the speed of the particle as a function of its X-coordinate.
View SolutionA particle of mass m and charge q moves with a constant velocity v along the positive x direction. It enters a region containing a uniform magnetic field B directed along the negative z direction, extending from x=a to x=b. The minimum value of v required so that the particle can just enter the region x>b is
View SolutionA coil of mass m and charge q moves with a constant velocity v along the positive x−direction . It enters a region containing a uniform magnetic field B directed along the negative z−direction , extending from x=a→x=b. The minimum value of v required so that the paRTICLE CAN JUST ENTER THE REGION X>B IS
View Solution
