A symmetric star shaped conducting wire loop is carrying a steady state current I as shown in the figure. The distance between the diametrically opposite vertices of the star is 4a. The magnitude of the magnetic field at the center of the loop is
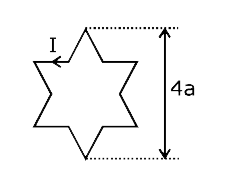
μ0I4πa6[√3−1]
μ0I4πa6[√3+1]
μ0I4πa3[2−√3]
μ0I4πa3[√3−1]
The correct Answer is:A
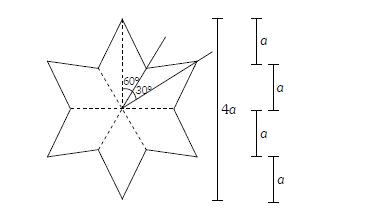
Total Magnetic Field at centre = 12 times magnetic field due to one wire
B=12μ0I4πa[sin60∘−sin30∘]=μ0I4πa×12[√32−12]
⇒B=μ0I4πa×6(√3−1)
Similar Questions
Knowledge Check
A square loop of side a carries a current I. The magnetic field at the center of the loop is
A2μ0I√2πaBμ0I√2πaC4μ0I√2πaDμ0IπaA wire bent in the shape of a regular n-polygonal loop carries a steady current I. Let l be the perpendicular distance of a given segment and R be the distance of vertex both from the centre of the loop. The magnitude of the magnetic field at the centre of the loop is given by -
Anμ0I2πlsin(π/n)Bnμ0I2πRsin(π/n)Cnμ0I2πlcos(π/n)Dnμ0I2πRcos(π/n)A square conducting loop of side length L carries a current I.The magnetic field at the centre of the loop is
Aindependent of LBproportional LCinversely proportional to LDlinearly proportional to L
A square loop is carrying a steady current I and the magnitude of its magnetic dipole moment is m.If this square loop is changed to a circular loop and it carries the same current the magnitude of the magnetic dipole moment of circular loop will be
View SolutionThe wire loop shown in figure carries a current as shown. The magnetic field at the centre O is ,

View SolutionAn equilateral triangular loop of wire of side / carries a current I. The magnetic field produced at the circumcentre of the loop is
View SolutionA rectangular loop of metallic wire is of length a and breadth b and carries a current i. The magnetic field at the centre of the loop is
View SolutionA star shaped loop ( with l= length of each section ) carries current i. Magnetic field at the centroid of the loop is
View Solution

