Two spherical black bodies of radii r1 and r2 and with surface temperature T1 and T2 respectively radiate the same power. Then the ratio of r1 and r2 will be
(T2T1)2
(T2T1)4
(T1T2)2
(T1T2)4
The correct Answer is:A
Power (P) =AεσT4
For same power
A∝1T4⇒(r1r2)2=(T2T1)4⇒r1r2=(T2T1)2
Similar Questions
Fig, shows a system of two concentric spheres of radii r1 andr2 and kept at temperature T1 and T2(T1>T2) respectively. Find the expression for radial rate of flow of heat through the substance.
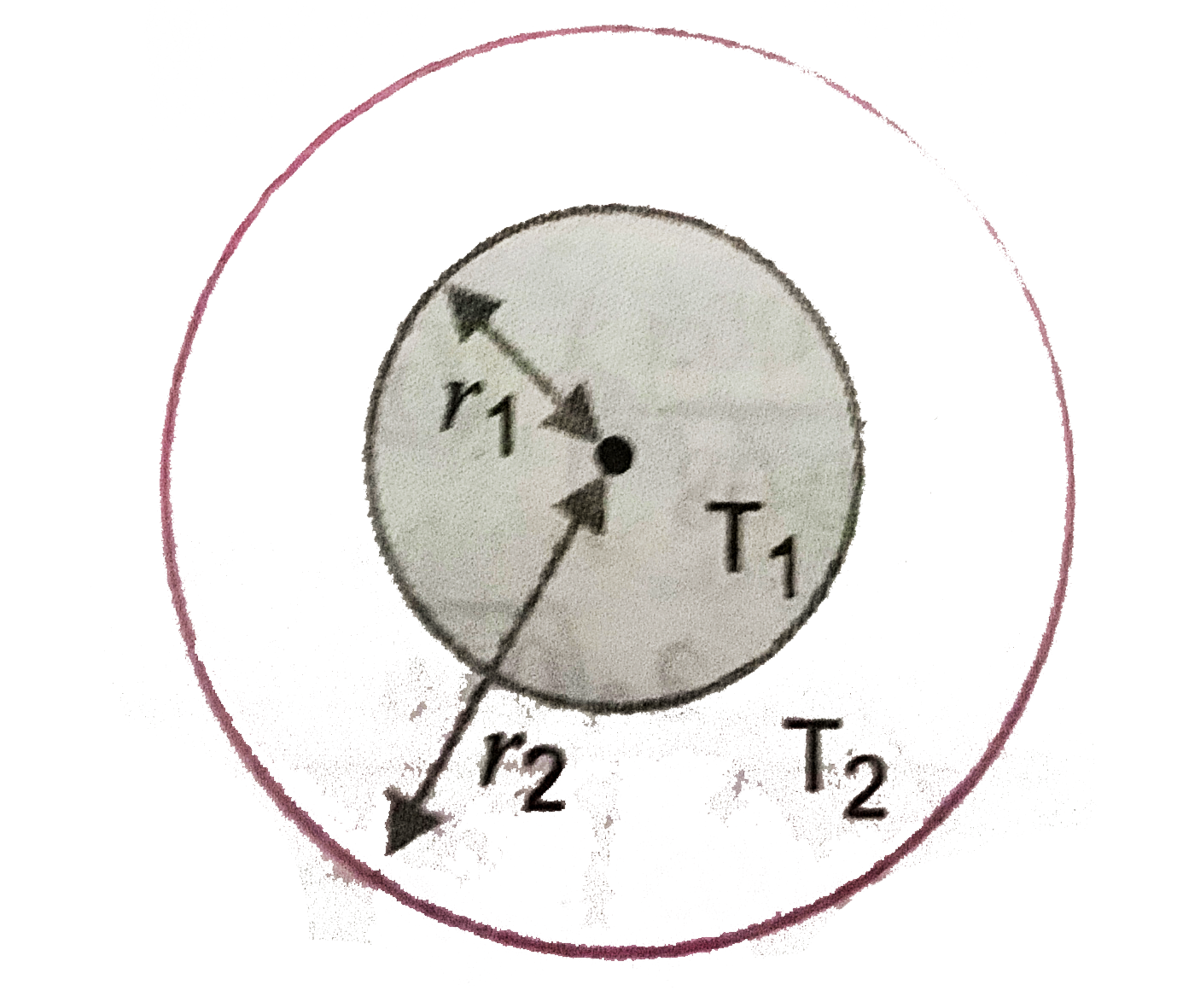
View SolutionTwo wires of same material and same length have radii r1andr2 respectively. Compare their resistances.
View Solution
Knowledge Check
Two spherical black bodies of radii R1 and R2 and with surface temperature T1 and T2 respectively radiate the same power. R1/R2 must be equal to
A(T1T2)2B(T2T1)2C(T1T2)4D(T2T1)4The figure shows a system of two concentric spheres of radii r1andr2 are kept at temperature T1andT2, respectively. The radial rate of flow of heat in a substance between the two concentric spheres is proportional to
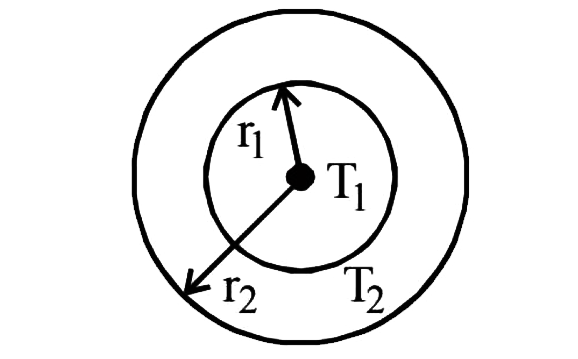 AIn(r2r1)Br2−r1r1r2C(r1−r2)Dr1r2r2−r1
AIn(r2r1)Br2−r1r1r2C(r1−r2)Dr1r2r2−r1Two spheres of the same material having radii r and 4r and temperature 2T0 and T0 respectively. The ratio of rate of radiation of energy by the sphere is
A1:1B1:2C2:1D3:1
Two charged spherical conductors of radii R1andR2 when connected by a connecting wire acquire charges q1andq2 respectively. Find the ratio of their charge densities in terms of their radil ?
View SolutionTwo planets have radii r1and2r1 and densities are ρ1and4ρ1 respectively. The ratio of their acceleration due to gravities is
View SolutionTwo planets have radii r1andr2 and densities d1andd2 respectively. Then the ratio of acceleration due to gravity on them is
View SolutionTwo spheres of radii R1 and R2 are made of the same material and are at the same temperature. The ratio of their thermal capacities is:
View SolutionA spherical black body of radius r radiated power P at temperature T when placed in surroundings at temprature T0(<<T) If R is the rate of colling .
View Solution