Two different isotherms representing the relationship between pressure p and volume V at a given temperature of the same ideal gas are shown for masses $m_{1}$ and $m_{2}$ then

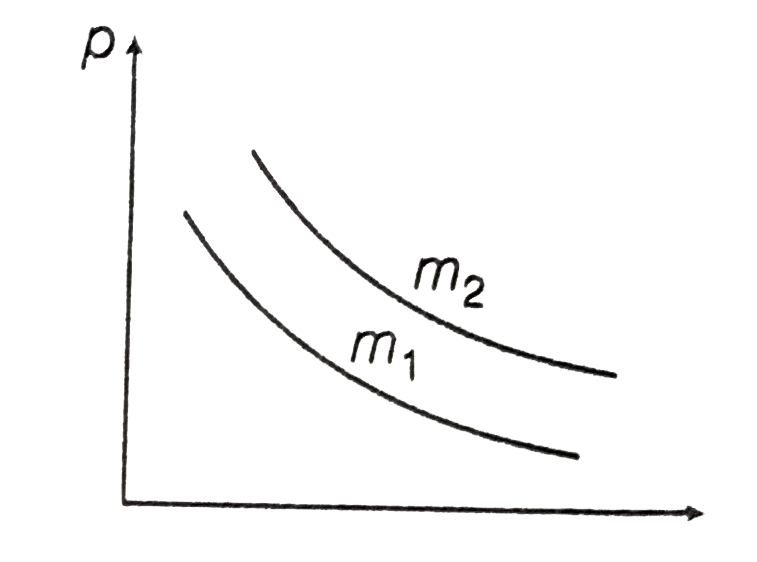
$m_{1} > m_{2}$

$m_{1} = m_{2}$

$m_{1} < m_{2}$

$m_{1} > < m_{2}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

$P V = \frac{m}{M} R T$
For 1st graph, $P = \frac{m_{1}}{M} \frac{R T}{V_{1}}$
For 2nd graph, $P = \frac{m_{2}}{M} \frac{R T}{V_{2}}$
$\frac{m_{1}}{m_{2}} = \frac{V_{1}}{V_{2}} \Rightarrow m \propto V$
As $V_{2} > V_{1} \Rightarrow m_{1} < m_{2}$

Updated on:21/07/2023

Class 11 PHYSICS KINETIC THEORY OF GASES

Similar Questions

Two different at constant. The relationship between volume V and the pressure P at a given temperature of same ideal gas are shown for masses m_1 and m_2 of the gas respectively. Then
03:33
View Solution
For same mass of two different ideal gases of molecular weight M_(1) and M_(2) plots of log V vs log P at a given constant temperature are show, Identify the correct option.
02:24
View Solution
Characters A and B with two identical and frictionless pistons have the same ideal gas at the same temperature and the same volume V. A mass of gas m_(A) And B is the mass of the gas m_(B) is. The gas in each cylinder is now circulated to 2 V isothermally the same final volume. The pressure in A and B respectively DeltaP And 1.5DeltaP is found. Then
View Solution
Characters A and B with two identical and frictionless pistons have the same ideal gas at the same temperature and the same volume V. A mass of gas m_(A) And B is the mass of the gas m_(B) is. The gas in each cylinder is now circulated to 2 V isothermally the same final volume. The pressure in A and B respectively DeltaP And 1.5DeltaP is found. Then
View Solution
The p -V diagram of two different masses m_(1) and m_(2) are drawn (as shown) at constant temperature (T) . State whether m_(1) gt m_(2) "or" m_(2) gt m_(2) . .
02:05
View Solution
The p -V diagram of two different masses m_(1) and m_(2) are drawn (as shown) at constant temperature (T) .
02:39
View Solution
Two different isotherms representing the relationship between pressure p and volume V at a given temperature of the same ideal gas are shown for masses $m_{1}$ and $m_{2}$ then

03:22
View Solution
Two monatomic ideal gas 1 and 2 of molecular masses $m_{1} and m_{2}$ respectively are enclosed in separate containers kept at same temperature. The ratio of the speed of sound in gas 1 to that in gas 2 is given by
03:27
View Solution

Two different isotherms representing the relationship between pressure p and volume V at a given temperature of the same ideal gas are shown for masses m1 and m2 then

The correct Answer is:C

PV=mMRT For 1st graph, P=m1MRTV1 For 2nd graph, P=m2MRTV2 m1m2=V1V2⇒m∝V As V2>V1⇒m1<m2

Similar Questions

Recommended Questions

Two different isotherms representing the relationship between pressure p and volume V at a given temperature of the same ideal gas are shown for masses $m_{1}$ and $m_{2}$ then

$P V = \frac{m}{M} R T$
For 1st graph, $P = \frac{m_{1}}{M} \frac{R T}{V_{1}}$
For 2nd graph, $P = \frac{m_{2}}{M} \frac{R T}{V_{2}}$
$\frac{m_{1}}{m_{2}} = \frac{V_{1}}{V_{2}} \Rightarrow m \propto V$
As $V_{2} > V_{1} \Rightarrow m_{1} < m_{2}$