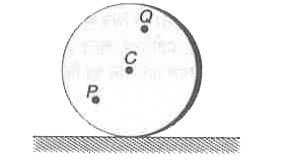
A disc is rolling (without slipping) on a horizontal surface. $C$ is its center and $Q$ and $P$ are two points equidistant from $C$ . Let $V_{P}, V_{Q}$ and $V_{C}$ be the magnitude of velocities of points $P, Q$ and $C$ respectively, then
.

$v_{Q} > v_{C} > v_{P}$

$v_{Q} < v_{C} < v_{P}$

$v_{Q} = v_{P}, v_{C} = \frac{v_{P}}{2}$

$v_{Q} < v_{C} > v_{P}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

As $O Q > O C > O P$
Hence, $v_{Q} > v_{C} > v_{P}$

Updated on:21/07/2023

Class 11 PHYSICS RIGID BODY DYNAMICS

Similar Questions

एक वृत्ताकार डिस्क एक क्षैतिज तल पर बिना फिसले एकसमान कोणीय चाल $ω$ से लुढ़क रही है। डिस्क का केंद्र C है तथा C से समदूरस्थ बिंदु Q तथा P है। यदि $P, Q,$ व C के वेगों के परिमाण क्रमशः $v_{P}, v_{Q}$ तथा $v_{C}$ हैं तब

View Solution
In a triangle $A B C$ , let P and Q be points on AB and AC respectively such that $P Q ∣ ∣ B C$ . Prove that the median $A D$ bisects $P Q$ .
View Solution
The ionization potential of hydrogenic ions P and Q are $V_{P} and V_{Q}$ respectively. If $V_{Q} < V_{P}$ then radii ......
View Solution
A disc is rolling (without slipping) on a horizontal surface. C is its centre and Q and P are two point equidistance from C. let upsilon_(p),upsilon_(Q) and upsilon_(C) be the magnitude of velocities of points P, Q, and C repsectively, (a). upsilon_(Q)gtupsilon_(C)gtupsilon_(P) (b). upsilon_(Q)ltupsilon_(C)ltupsilon_(P) (c). upsilon_(Q)=upsilon_(P),upsilon_(C)=(1)/(2)upsilon_(P) (d). upsilon_(Q)ltupsilon_(C)gtupsilon_(P)
View Solution
A disc is rolling without slipping on a horizontal surface with C, as its centre and Q and P the two points equidistant from C. Let $v_{P}, v_{Q} and v_{C}$ be the magnitudes of velocities of point P,Q and C respectively, then

View Solution
A disc rolls on ground without slipping. Velocity of centre of mass is $v$ . There is a point P on circumference of disc at angle $θ$ . Suppose, $v_{P}$ is the speed of this point. Then, match the following columns

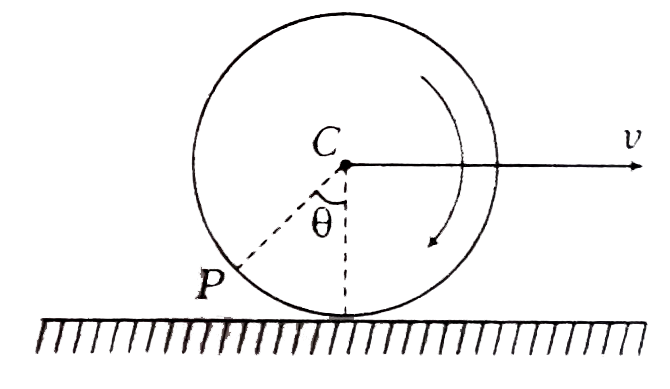
$\begin{matrix} Column-I & Column-II (A) & If θ = 60^{\circ} & (p) & v_{P} = \sqrt{2} v (B) & If θ = 90^{\circ} & (q) & v_{P} = v (C) & If θ = 120^{\circ} & (r) & v_{P} = 2 v D) & If θ = 180^{\circ} & (s) & v_{P} = \sqrt{3} v \end{matrix}$
View Solution
A cylinder rolls on the planks A and B without relative sliding. If the planks move with velocities -2vhati, vhati respectively and the plank A has acceleration veca(=ahati) , find the: (a) v_(C) and v_(Q) and (b) a_(C) and a_(Q) .
View Solution
A disc is rolling (without slipping) on a horizontal surface. C is its center and Q and P are two points equidistant from C . Let V_(P), V_(Q) and V_(C) be the magnitude of velocities of points P, Q and C respectively, then .
View Solution

A disc is rolling (without slipping) on a horizontal surface. C is its center and Q and P are two points equidistant from C. Let VP,VQ and VC be the magnitude of velocities of points P,Q and C respectively, then .

The correct Answer is:A

As OQ>OC>OP Hence, vQ>vC>vP

Similar Questions

Recommended Questions

A disc is rolling (without slipping) on a horizontal surface. $C$ is its center and $Q$ and $P$ are two points equidistant from $C$ . Let $V_{P}, V_{Q}$ and $V_{C}$ be the magnitude of velocities of points $P, Q$ and $C$ respectively, then
.

As $O Q > O C > O P$
Hence, $v_{Q} > v_{C} > v_{P}$