A circular disc of radius R is removed from a bigger circular disc of radius 2R such that the circumference of the discs coincoid . The centre of mass of the new disc is αR from the centre of the bigger disc . the value of α is
1/3
1/2
1/6
1/4
The correct Answer is:A
By super position principle
−3M4αR+M4R=0
implies α=13
Aliter : A1=π(2R)2,x1=0,y1=0
A2=π®2,x2=R,y2=0
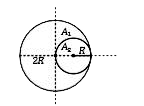
XCMA1x1−A2x2A1−A2=(4πR2×0)−[(πR2)×R]4πR2−πR2
XCM=−R3=αR
:. α=13
Similar Questions
A circular disc of radius R is removed from a bigger circular disc of radius 2R such that the circumferences of the disc coincide. The centre of mass of the new disc is αR from the centre of the bigger disc. The value of α is :
View SolutionA circular disc of radius R is removed from a bigger circular disc of radius 2R such that the circumferences of the discs touch. The centre of mass of the new disc is at a distance aR from the centre of the bigger disc. The value of a is
View SolutionA circular disc of radius R is removed from a bigger of the discs coincide. The centre of mass of the new disc is ar from the centre of the bigger disc. The value of α is
View SolutionA solid toy is in the form of a hemisphere surmounted by a right circular cone of same radius. The height of the cone is 10 Cm and the radius of the base is 7 cm. Determine the volume of the toy. Also find the area of the coloured sheet required to cover the toy. (Use π=22/7 and √149=12.2)
View SolutionFrom the circular disc of radius 4R two small discs of radius R are cut off. The centre of mass of the new structure will be at

View SolutionThe distance of center of mass from the center of a uniform disc of radius R. if a circular plate is removed from the disc as shown in figure will be (C is centre of complete disc)
View SolutionA circular disc of radius R is removed from a bigger circular disc of radius 2 R such that the circumferences of the discs coincide. The center of mass of new disc is alpha R from the center of the bigger disc. The value of alpha is
View SolutionA circular disc of radius Ris removed from a bigger circular disc of radius 2R such that the circumferences of the discs coincide. The centre of mass of the new disc is alphaR from the centre of the bigger disc. The value of
View Solution