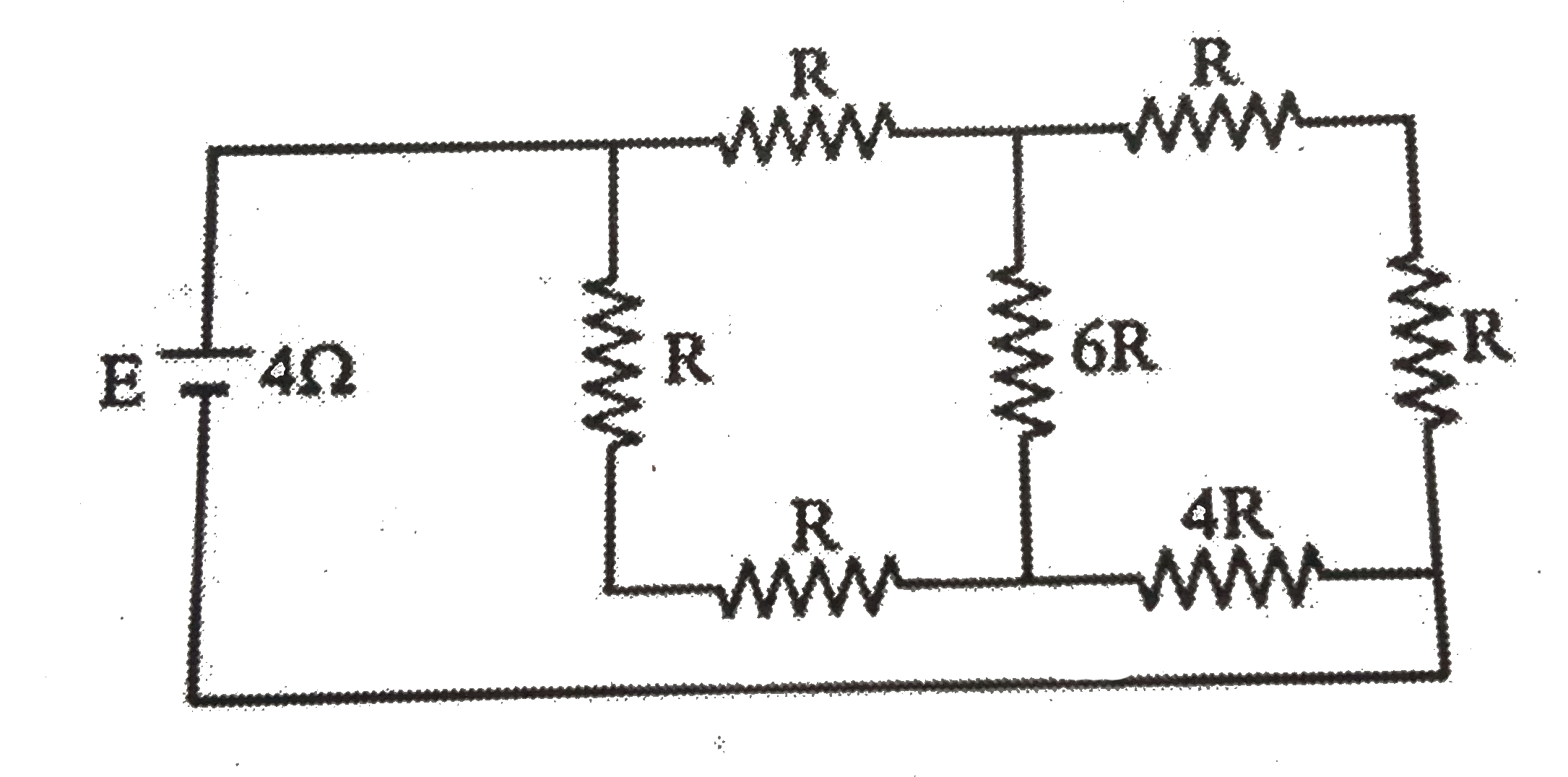
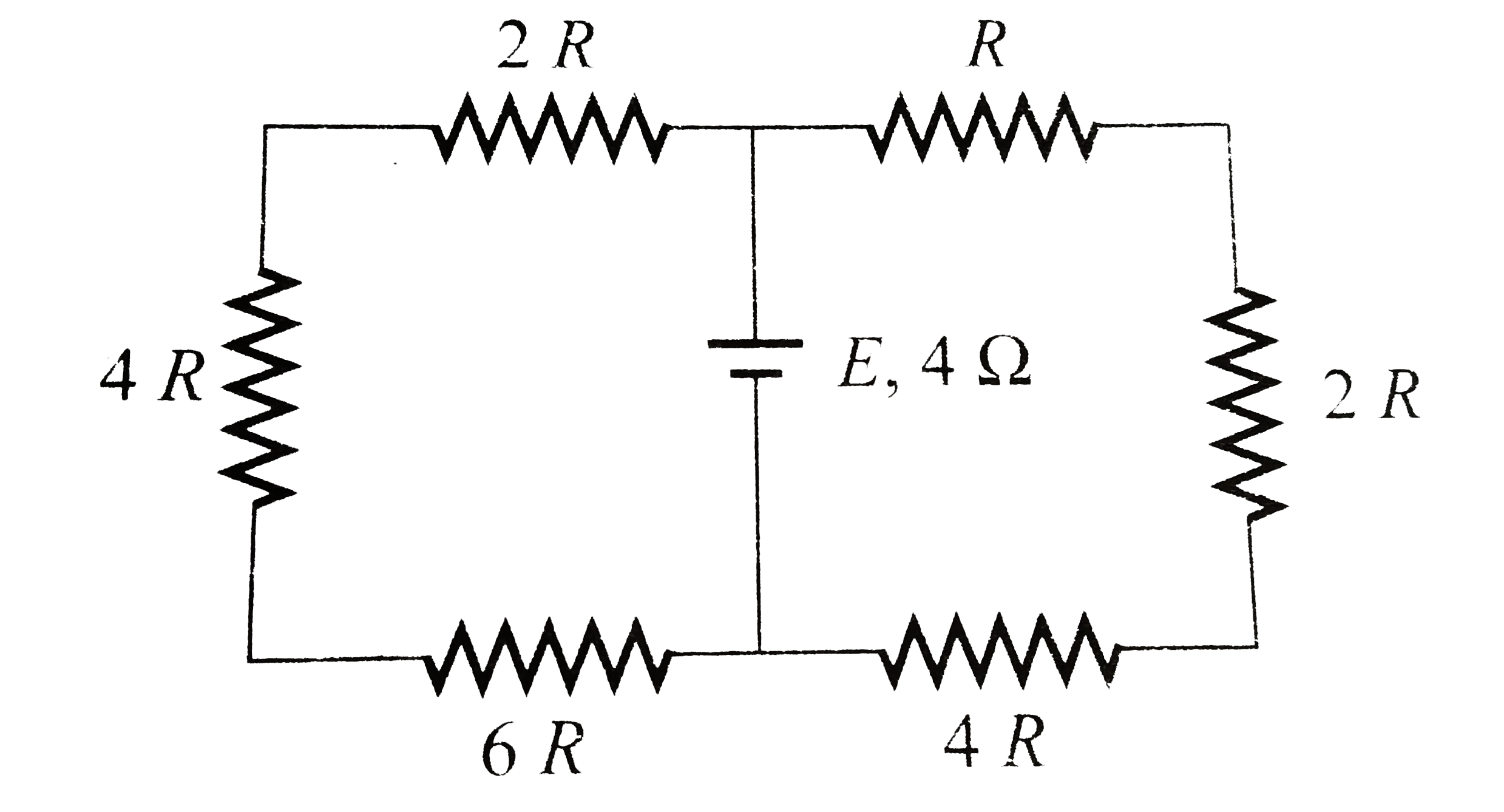
A battery of internal resistance 4Ω is connected to the network of resistance as shown. In order to give the maximum power to the network, the value of R should be-
4/9
8/9
2
18
The correct Answer is:C
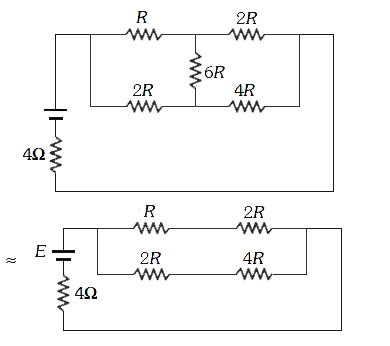
A balanced Wheatstone bridge therefore no current will pass through 6R.
External resistance.
=3R×6R9R=2R
For maximum power.
Rinternal=Rexternal
⇒4=2R⇒R=2Ω
Similar Questions
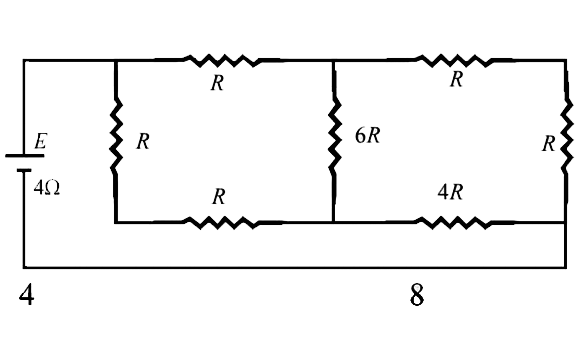
A battery of internal resistance 4Ω is connected to the network of resistance as shown . In order that the maximum power can be delivered to the network, the value of R in Ω should be
 .
.
View SolutionA battery of internal resistance 4Omega is connected to the network of resistances as shown in the fig. In order that the maximum power can be delivered to the network, the value of R in Omega should be
View SolutionA battery if internal resistance 4Omega is connected to the network of ressitances as shown. What must be the value of R so that maximum power is delivered to the network? Find the maximum power?
View SolutionA battery of internal resistance 4Ω is connected to the network of resistances as shown in the fig. In order that the maximum power can be delivered to the network, the value of R in Ω should be
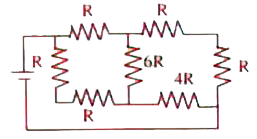
View SolutionA battery of internal resistance 4Ω is connected to the network of resistance as shown . In order that the maximum power can be delivered to the network, the value of R in Ω should be
 .
.
View SolutionA battery of internal resistance 4Ω is connected to the network of the resistance as shown in figure . If the maximum power can be delivered to the network, the magnitude of resistance in Ωshould be
View SolutionA network of resistances is connected to a 16V battery with internal resistance of 1 Omega as shown in Fig. Compute the equivalent resistance of the network
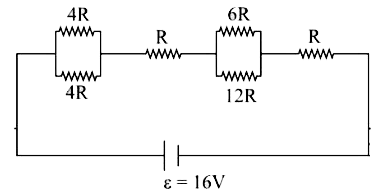
View SolutionThe resistive network shown below is connected to a D.C. source of 16V. The power consumed by the network is 4 Watt. The value of R (in Ω) is _______.
View Solution