In figure shows a rectangular block with dimensions x , 2 x and 4 x . Electrical contacts can be made to the block between opposite pairs of faces (for example, between the faces labelled A - A , B - B and C - C ). Between which two faces would the maximum electrical resistance be obtained ( A - A : Top and bottom faces, B - B : Left and right faces, C - C : Front and rear faces)
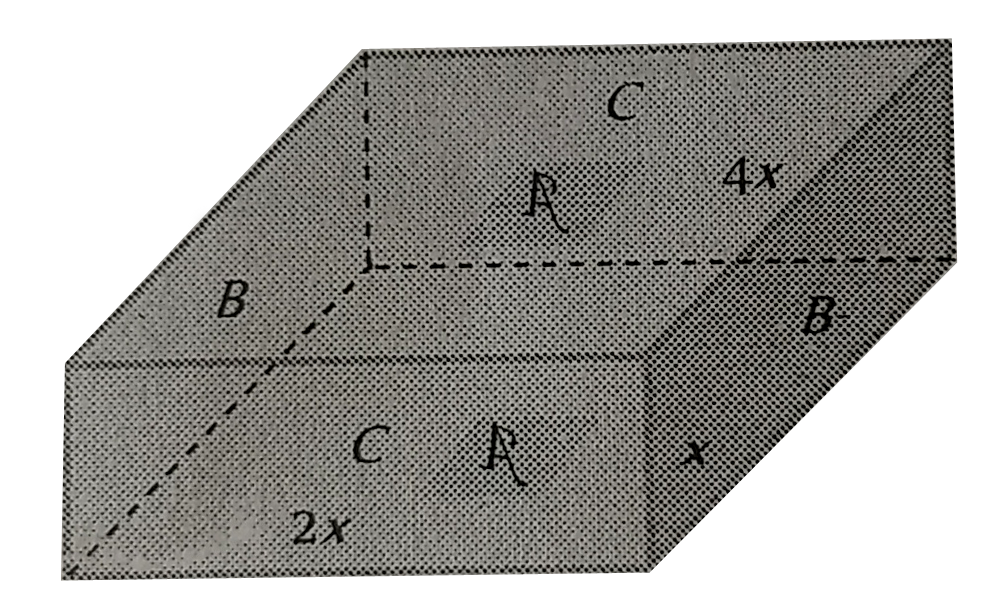
A-A
B-B
C-C
Same for all three pairs
The correct Answer is:C
R=ρlA
I→ maximum ⇒l=4x
A→ minimum ⇒A=x×2x=2x2
∴ for face C - C, R will be maximum.
Similar Questions
A block has dimensions 1 cm, 2cm, 3cm Ratio of the maximum and minimum resistance between any two points of opposite faces of this block is
View SolutionThe dimensions of a block are (1 cm × 1 cm × 100 cm) the specific resistance of its material is 3xx10^(-7) ohm × metre. Then resistance between the rectangular faces is :-
View SolutionThe dimensions of a mangnin block are 1 cm xx 1 cm xx100 cm The electrical resistivity of mangnin is 4.4 xx10^(-7) ohm–meter. The resistance between the opposite rectangular faces is –
View SolutionAll the edges of a block with parallel faces are unequal . Its longest edge is twice its shortest edge. The ratio of the maximum resistance between parallel faces is :
View SolutionThe sides of rectangular block are 2 cm, 3 cm and 4 cm. The ratio of the maximum to minimum resistance between its parallel faces is
View SolutionThe sides of rectangular block are 2cm, 3cm and 4 cm . The ratio of the maximum to minimum resistance between its parallel faces is
View SolutionA rectangular block of metal of resistivity p has dimensions d xx 2d xx 3d . A potential difference V is applied between two opposite faces of the block. (a) To which two faces of the block should the potential difference V be applied to give the maximum current density? What is the maximum current density? (b) To which two faces of the block should the potential difference V be applied to give the maximum current? What is this maximum current?
View SolutionAn electric field given →E=4.0ˆi−3.0(y2+2.0)j pierces a Gaussian cube of edge length 2.0 m and positioned as shown in the figure. (The magnitude E is in newtons per coulomb and the position z is in meters.) what is the electric flux through the (a) top face, (b) bottom face, (c) left face , (b) bottom facem (c) left face, and (d) back face ? (e) what is the net electric flux through the cube ?
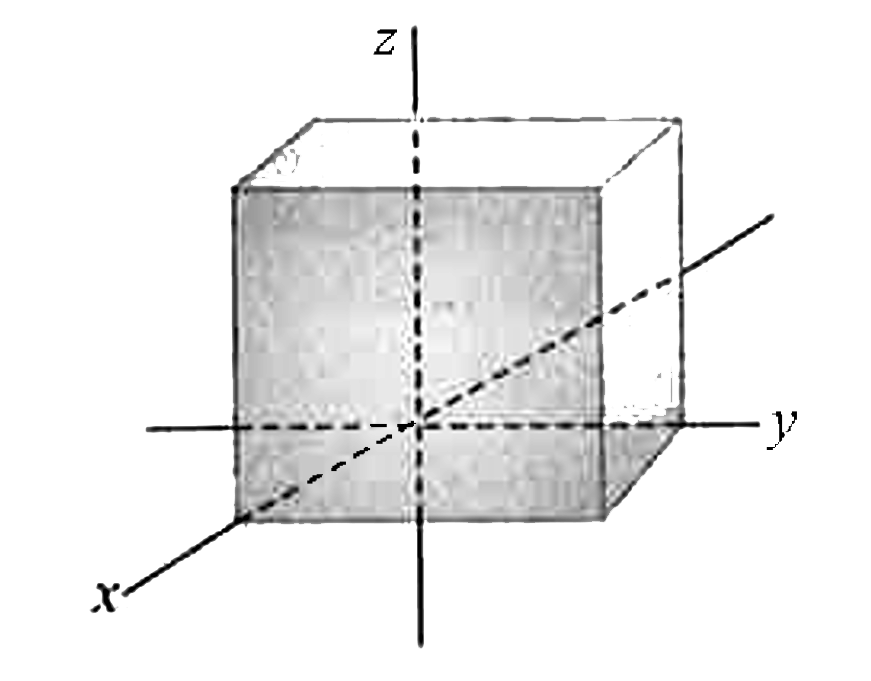
View Solution
