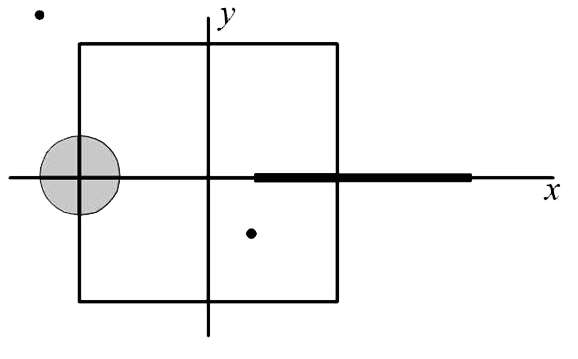
A disc of radius a/4 having a uniformly distributed charge 6C is placed in the x-y plane with its centre at (−a/2,0,0). A rod of length a carrying a uniformly distributed charge 8C is placed on the x-axis from x=a/4 to x=5a/4. Two point charges −7C and 3C are placed at (a/4,−a/4,0) and (−3a/4,3a/4,0), respectively. Conisder a cubical surface formed by isx surfaces x=±a/2, y=±a/2, z=±a/2. The electric flux through this cubical surface is
−2Cε0
2Cε0
10Cε0
12Cε0
The correct Answer is:A
Total charge inside cube
=3C+8Ca×a4−7C=3C+2C−7C=−2C
Flux through the surface ϕ=1ε0Q=−2cε0.
Similar Questions
An infinite number of charges , each equal to q , are placed along the x - axis at x = 1, x=2,x=4,x=8 and so on . The potential at x = 0 due to this set of charges is
View SolutionA ring of radius R has charge Q distributed uniformly on it. At a distance x (!=0) from centre on its axis. (consider axial points only)
View SolutionAn infinite number of charges, each equal to q, are placed to q, are placed along the x-axis at x =1, x = 2, x = 4, x = 8 and so on. What is the potential at x = 0 due to this set of charges ?
View SolutionThe number of values of 'a' for which
(a2−3a+2)x2+(a2−5a+6)x+a2−4=0 is an identity is
View SolutionA disc of radius a/4 having a uniformly distributed charge 6C is placed in the x-y plane with its centre at (−a/2,0,0). A rod of length a carrying a uniformly distributed charge 8C is placed on the x-axis from x=a/4 to x=5a/4. Two point charges −7C and 3C are placed at (a/4,−a/4,0) and (−3a/4,3a/4,0), respectively. Conisder a cubical surface formed by isx surfaces x=±a/2, y=±a/2, z=±a/2. The electric flux through this cubical surface is

View SolutionAxis of the parabola x2−4x−4y+10=0 is
View SolutionEqual charges each of 20muC are placed at 0, 2, 4, 8, 16 cm on X-axis. Find the electric field experienced by the charge at x=2cm.
View SolutionAn infinite number of charges, each equal to q are placed along x axis at x = 1, x = 2, x = 4, x = 8………… and so on. What is the electric field at the point x = 0 due to this set of charges.
View Solution
