A container of height 10 m which is open at the top. has water to its full height. Two small openings are made on the walls of the container one exactly at the middle and the other at the bottom. The ratio of the velocities with which water comes out from the middle and the bottom region respectively is
2
12
√2
1√2
The correct Answer is:D
v1v2=√2gh/2√2gh=√12=1√2.
Similar Questions
एक 10 m ऊँचाई का टैंक ऊपरी सिरे पर खुला है, इसमें पूर्ण ऊँचाई तक जल भरा है। टैंक में दो छोटे छिद्र बनाये जाते है, एक छिद्र ठीक मध्य में तथा दूसरा तली पर। अतः मध्य तथा तली के क्षेत्र से निर्गत जल के वेगों का अनुपात है
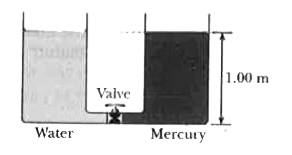
View SolutionTwo identical containers are open at the top and are connected at the bottom via a tube of negligible volume and a valve that is closed . Both containers are filled initially to the same height of 1.00 m , one with water , the other with mercury , as the figure indicates . The valve is then opened . Water and mercury are immiscible . Determine the fluid level in the left container when equilibrium is reestablished .
View SolutionA container of height 10m which is open at the top, has water to its full height. Two small openings are made on the walls of the container one exactly at the middle and the other at the bottom. The ratio of the velocities with which water comes out from the middle and the bottom region respectively is
View SolutionA cylindrical drum open at the top contains 30 liters of water. It drains out through a small opening at the bottom. 10 litres of water comes out in first 10%, the next 10 litres in a further time t2 and the last 10 litres in a further time t3. Then
View SolutionA cylindrical drum, open at the top, contains 30 litres of water. It drains out through a small opening at the bottom. 10 litres of water comes out in time t1, the next 10litres in a further time t2 and the last 10 litres in a further time t3 Then,
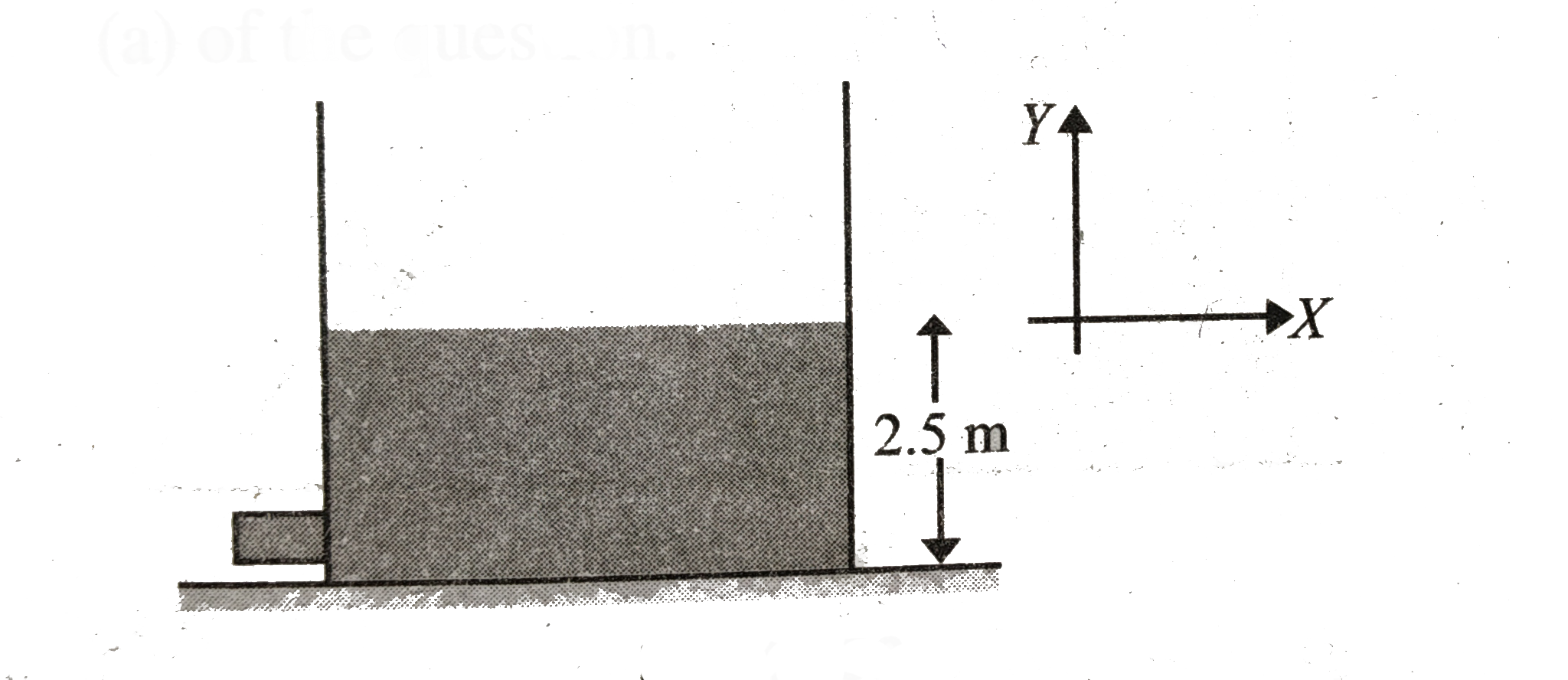
View SolutionWater is filled to a height of 2.5m in a container lying rest on a horizontal surface as shown in the figure. The bottom of the container is square shaped of side √3 and its top is open. There is a very small hole in the vertical wall of the container near the bottom. A small cap closes the hole. The hole gets opened when pressure near the bottom becomes more than 4×104 pascal
a, What minimum acceleration can be given to the container in the positive x-direction so that the cap will come out of the hole? Assume water does not spill out of container when the container accelerates.
Find the velocity of efflux as soon as the container starts moving with the acceleration calculated in part a. of the question.
View SolutionWater is filled in a container upto height 3m. A small hole of area 'a' is punched in the wall of the container at a height 52.5 cm from the bottom. The cross sectional area of the container is A. If a/A=0.1 then v2 is (where v is the velocity of water coming out of the hole)
View Solutionशीर्ष पर खुला R त्रिज्या और H ऊंचाई का एक बेलनाकार पात्र है जो पूरी तरह से जल से भरा हुआ है। बर्तन के तल के पास त्रिज्या r का एक छोटा वृत्ताकार छिद्र बना होता है। 25 % जल को बाहर प्रवाहित होने में लगा समय है:
View Solution