A rocket is launched normal to the surface of the earth, away from the sun, along the line joining the sun and the earth. The sun is 3×105 times heavier than the earth and is at a distance 2.5×104 times larger than the radius of the earth. the escape velocity from earth's gravitational field is ue=11.2kms−1. The minmum initial velocity (ue)=11.2kms−1. the minimum initial velocity (us) required for the rocket to be able to leave the sun-earth system is closest to (Ignore the rotation of the earth and the presence of any other planet
vs=72kms−1
vs=22kms−1
vs=42kms−1
vs=62kms−1
The correct Answer is:C
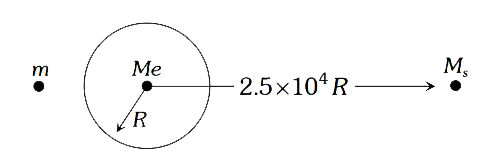
Given √2GMeR=11.2km/s
12mv2−GmMeR−GMsm2.5×104R≥0
for v=ve
v2e=2GMeR=2GMs2.5×104R=2GMeR+6×105GMe2.5×104R
=GMeR(2+24)=√26GMeR=40.4km/sec
Similar Questions
Jupiter has a mass 318 times that of the earth, and its radius is 11.2 times the earth's radius. Estimate the escape velocity of a body from Jupiter's surface, given that the escape velocity from the earth's surface is 11.2 kms^(-1) .
View Solutionरॉकेट भूतल के अभिलंबवत सूर्य एवं पृथ्वी को जोड़ने वाली रेखा में सूर्य से दूर की तरफ (radially outward from the direction of the sun) प्रक्षेपित किया गया है। सूर्य पृथ्वी से 3×105 गुना भारी है एवं पृथ्वी की त्रिज्या से 2.5×104 गुनी दूरी पर स्थि है। पृथ्वी के गुरुत्वाकर्षण क्षेत्र के लिए पलायन गति (escape velocity) =11.2kms−1 है। रॉकेट को सूर्य एवं पृथ्वी निकाय (Sun Earth system) के गुरुत्वाकर्षण से मुक्त होने के लिए कम से कम प्रारंभिक वेग (vs) का निकटतम मान है। (पृथ्वी की चक्रीय गति और परिभ्रमण तथा किसी अन्य ग्रह ही उपस्थिति की उपेक्षा करें)
View SolutionThe escape velocity from the earth’s surface is 11.2km/sThe .escape velocity from the surface of the moon is ? Given that the mass of the earth is 80 times that of the moon and radius of the earth is 4 times that of the moon,
View SolutionJupiter has a mass 318 times that of earth, and its radius is 11.2 times the earth's radius Estimate the escape velocity of a body from Jupiter's surface, given that the escape velocity from the earth's surface 11.2kms−1.
View SolutionEscape velocity on earth is 11.2kms−1what would be the escape velocity on a planet whose mass is 1000 times and radius is 10 times that of earth ?
View SolutionHow far from earth must a body be along a line towards the sun so that the sun's gravitational pull on it balances that of the earth . Distance between sun and earth's centre is 1.5 xx 10^(10) km . Mass of the sun is 3.24 xx 10^(5) times mass of the earth .
View SolutionA rocket is launched normal to the surface of the earth, away from the sun, along the line joining the sun and the earth. The sun is 3 xx 10^(5) times heavier than the earth and is at a distance 2.5 xx 10^(4) times larger than the radius of the earth. the escape velocity from earth's gravitational field is u_(e) = 11.2 kms^(-1) . The minmum initial velocity (u_(e)) = 11.2 kms^(-1) . the minimum initial velocity (u_(s)) required for the rocket to be able to leave the sun-earth system is closest to (Ignore the rotation of the earth and the presence of any other planet
View SolutionThe escape velocity for the earth is 11.2 km / sec . The mass of another planet is 100 times that of the earth and its radius is 4 times that of the earth. The escape velocity for this planet will be
View Solution
