A body at rest is moved along a horizontal straight line by a machine delivering a constant power. The distance moved by the body in time ‘t’ is proportional to:
t32
t12
t34
t14
The correct Answer is:A
P= constant
12mv2=Pt
⇒v∝√t
dxdt=C√t {∵ C= constant}
By integration, x=Ct12+112+1⇒x∝t3/2
Similar Questions
A body is moved along a straight line by a machine delivering constant power . The distance moved by the body is time t is proptional to
View SolutionA box is moved along a straight line by a machine delivering constant power. The distance moved by the body in time t is proportional to
View SolutionA body is moved along a straight line by a machine delivering a constant power. The distance moved by the body in time t is proportional to
View SolutionA body is moved along a straight line by a machine delivering constant power . The distance moved by the body is time t is proptional to
View SolutionA body is moved along a straight line by a machine delivering a power proportional to time (P∝t). Then, match the following.
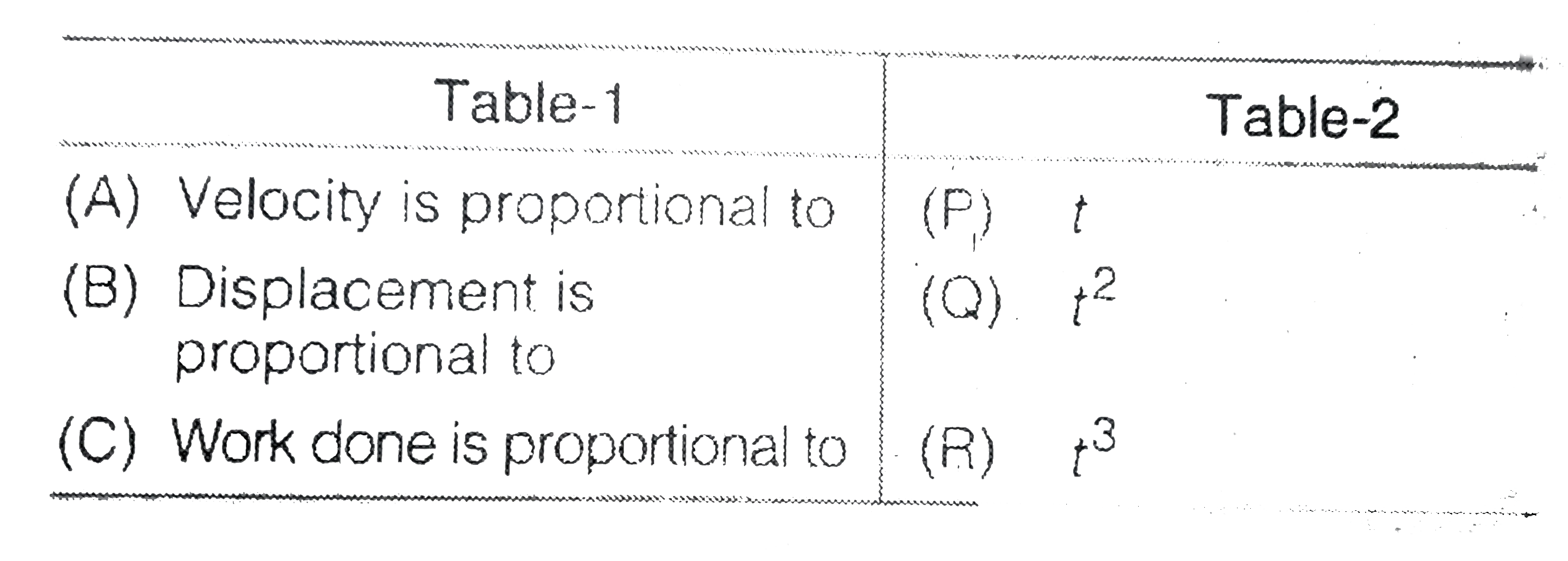
View SolutionA body is moved along a straight line by a machine delivering constant power . The distance moved by the body is time t is proptional to
View SolutionA body is moved along a straight line by a machine delivering constant power . The distance moved by the body is time t is proptional to
View SolutionA body of mass m at rest starts moving along straight line by a machine delivering a constant power distance travelled by body in time t is
View Solution
