A small body is released from a height H of an inclined plane. At the bottom of the plane is a loop of radius R as shown : ignoring friction, the minimum H required for the body to just complete the loop (that is, reach the point O) is
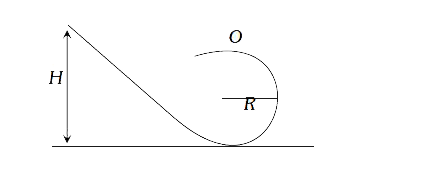
2R
5R2
3R
7R2
The correct Answer is:B
12m5gR=mgH⇒H=52R
Similar Questions
An inclined track ends in a circular loop of radius 'r'. From what height on the track a particle should be released so that it completes that loop in the vertical plane?
View SolutionA ball of 1 g released down an inclined plane describes a circle of radius 10 cm in the vertical plane on reaching the bottom. The minimum height of the inclined plane is
View SolutionA body starts rolling down an inclined plane of length L and height h. This body reaches the bottom of the plane in time t. The relation between L and t is?
View SolutionA block is released from the top of an inclined plane of inclination theta and height h. Time required to reach the foot of the inclined plane is
View SolutionThe minimum velocity required for a body at the bottom of a vertical circle to complete the circle of radius .R. is √5gR A body is released from the top edge of an inclined plane as shown in the figure. The minimum value of .h. for which the body can complete the vertical circle is:
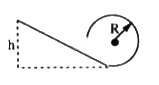
View SolutionA body slides without friction from a height H = 60 cm and then loops the loop of radius R = 20 cm at the bottom of incline. The ratio of forces exerted on the body by the track at A,B and C are (g = 10 ms^(-2))
View SolutionA frictionless track ABCDE ends in a circular loop of radius R. A body slides down the track from point A which is at height h = 5cm. Maximum value of R for a body to complete the loop successfully is
View SolutionA body starts rolling down an inclined plane of length L and height h. This body reaches the bottom of the plane in time t. The relation between L and t is?
View Solution
