The displacement of a particle is moving by x=(t−2)2 where x is in metres and t in second. The distance covered by the particle in first 4 seconds is.
4m
8m
12m
16m
The correct Answer is:B
Given x=(t−2)2
v=dxdt=ddt(t−2)2=2(t−2)m/s
a=dvdt=ddt[2(t−2)]
=2[1−0]=2m/s2
when t = 0 , v = -4m/s
t = 2s , v = 0m/s
t = 4s, v = 4m/s
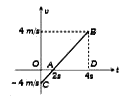
v-t graph is shown in adjacent diagram
Distance travelled = graph of the area
= Area OAC + Area ABD
=4×22+12×2×4=8m
Similar Questions
The position x of a particle varies with time t as x=6+12t−2t2 where x is in metre and t is in seconds. The distance travelled by the particle in first five seconds is
View SolutionThe displacement of a particle is given by x = (t-2)^(2) where x is in metre and t in second. The distance covered by the particle in first 4 seconds is
View SolutionThe displacement of a particle is given by x=(t−2)2 where x is in metres and t in seconds. The distance covered by the particle in first 4 seconds is
View SolutionThe displacement of a particle is given by x=(t−2)2 where x is in metres and t in seconds. The distance covered by the particle in first 4 seconds is
View SolutionThe displacement of a particle is moving by x = (t - 2)^2 where x is in metres and t in second. The distance covered by the particle in first 4 seconds is.
View SolutionA particle moves along x-axis obeying the equation x=t(t-1)(t-2), where x (in metres) is the position of the particle at any time t (in seconds). The displacement when the velocity of the particle is zero, is
View SolutionThe displacement x of a particle moving in one dimension under the action of a constant force is related to the time t by the equation t=√x+3, where x is in metre and t is in second. The work done by the force in the first 6 second is
View SolutionThe position of a particle is given by
x=2(t−t2)
where t is expressed in seconds and x is in metre.
The acceleration of the particle is
View Solution
