A particle is projected horizontally with a speed V=5m/s from the top of a plane inclined at an angle to the horizontal
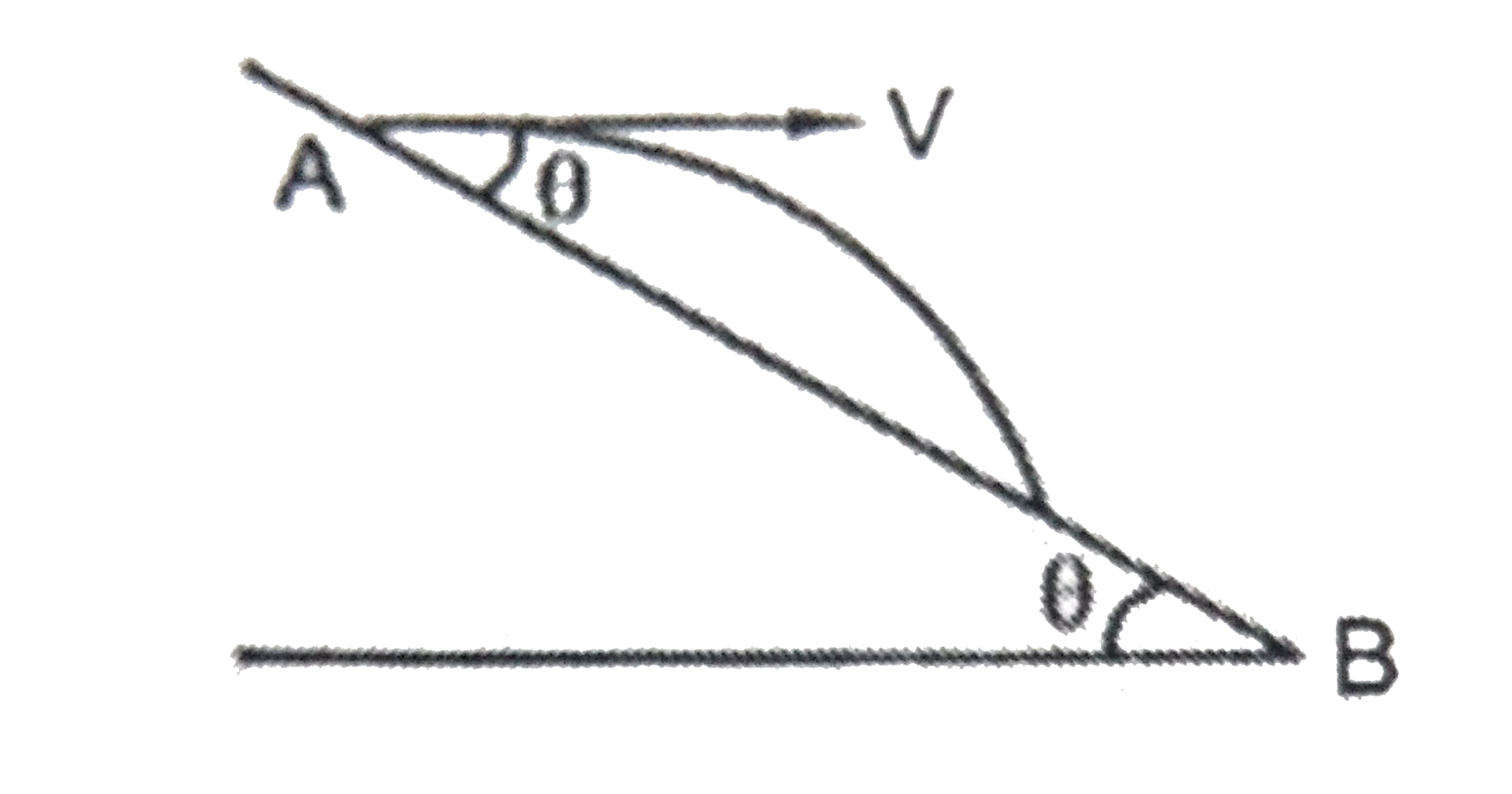
What is the velocity of the particle just before it hits the plane?
The correct Answer is:
. . . (i)
. . .(ii)
And
And
And at t = T
= 9.01 m/sec
Similar Questions
A particle is projected horizontally with a speed ''u'' rom the top of plane incline at an angle θ with the horizontal. How far from the point of projection will the particle strike the plane ?
View SolutionA particle is projected horizontally with a speed ''u'' rom the top of plane incline at an angle θ with the horizontal. How far from the point of projection will the particle strike the plane ?
View SolutionA particle is projected horizontally with a speed ''u'' rom the top of plane incline at an angle θ with the horizontal. How far from the point of projection will the particle strike the plane ?
View SolutionA particle is projected horizontally with a speed u from the top of plane inclined at an angle θ with the horizontal. How far from the point of projection will the particle strike the plane ?

View SolutionA particle is projected horizontally with a speed "u" from the top of plane incline at an angle ..θ.. with the horizontal. How far from the point of projection will the particle strike the plane ?
View SolutionA particle is projected horizontally with a speed "u" from the top of plane incline at an angle ..θ.. with the horizontal. How far from the point of projection will the particle strike the plane ?
View SolutionA particle is projected horizontally with a speed u from the top of plane inclined at an angle θ with the horizontal. How far from the point of projection will particle strike the plane?
View SolutionA particle is projected horizontally with a speed "u" from the top of plane incline at an angle "θ" with the horizontal. How far from the point of projection will the particle strike the plane?
View Solution
