vector $\to a$ lies in the xy plane, has a magnitude of 18 units, and points in a direction $250^{\circ}$ from the positive direction of the x axis. Also, vector $\to b$ has a magnitude of 12 units and points in the positive direction of the z axis. What is the vector product $\to c = \to a \times \to b$ ?

A

30

B

35

C

40

D

45

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

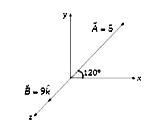
$\to A = (5 cos 120) ˆ i + (5 sin 120) ˆ j = \frac{- 5}{2} ˆ i + \frac{5 \sqrt{3}}{2} ˆ j$
$\to B = 9 ˆ k$
$\to A \times \to B = ∣ ∣ ∣ ∣ ∣ \begin{matrix} ˆ i & ˆ j & ˆ k \frac{- 5}{2} & \frac{5 \sqrt{3}}{2} & 0 0 & 0 & 9 \end{matrix} ∣ ∣ ∣ ∣ ∣ = - (\frac{- 45}{2}) ˆ j + (\frac{45 \sqrt{3}}{2}) ˆ i$
$\to A \times \to B = \frac{45 \sqrt{3}}{2} ˆ i + \frac{45}{2} ˆ j$
$∣ ∣ \to A \times \to B ∣ ∣ = \sqrt{{(\frac{45 \sqrt{3}}{2})}^{2} + {(\frac{45}{2})}^{2}} = \frac{45}{2} \sqrt{4} = 45$

Updated on:21/07/2023

|

Class 11 PHYSICS BASIC MATHEMATICS

Similar Questions

Recommended Questions

Home
Ask Doubt
Profile