Study the following information carefully and answer the given questions.
The given data is regarding the number of doctors (D) and engineers (E) in Colleges A, B and C.
College A: Respective ratio between male doctors and male engineers is 12 : 5 and the difference between them, is 280. Total number of females (D+E) is 300. Number of male engineers is 30 more than the number of female engineers.
College B: Total number of males (D+E)=total number of females in college A (D + E). Respective ratio between total number of males (D+E) and total number of females (D +E) is 6:7 in College B. Respective ratio between the number of female doctors and the number of female engineers is 2:3. The number of male, doctors is one-third of the number of male engineers in College C.
College C : Number of male engineers is 1.5 times the number of female doctors. Total number of females (D +E) is 550. The number of female engineers is 70 more than the number of female doctors. Total number of males (D + E) is 760.
What is the total number of engineers (male ± female) in colleges A, B and C together?
1390
1300
1510
1430
The correct Answer is:D
To solve the problem step-by-step, we will analyze the information provided for each college and calculate the required values.
Step 1: Analyze College A
1. Given Ratios and Differences:
- Ratio of male doctors (D) to male engineers (E) = 12:5
- Difference between male doctors and male engineers = 280
2. Let the number of male doctors be 12x and male engineers be 5x:
12x−5x=280⟹7x=280⟹x=40
3. Calculate the number of male doctors and engineers:
- Male doctors = 12x=12×40=480
- Male engineers = 5x=5×40=200
4. Total number of females (D + E) in College A:
- Total females = 300
- Male engineers = 200, so:
Female engineers=Male engineers−30=200−30=170
- Female doctors = Total females - Female engineers = 300−170=130
Step 2: Analyze College B
1. Total number of males (D + E) in College B:
- Total males = Total females in College A = 300
2. Ratios of males to females:
- Ratio of males to females = 6:7
- Let males = 6y and females = 7y:
6y+7y=300⟹13y=300⟹y=30013≈23.08
3. Calculate total males and females:
- Males = 6y=6×23.08≈138.46 (round to 138)
- Females = 7y=7×23.08≈161.54 (round to 162)
4. Female doctors and engineers ratio:
- Ratio = 2:3
- Let female doctors = 2z and female engineers = 3z:
2z+3z=162⟹5z=162⟹z=1625=32.4
- Female doctors = 2z=64.8 (round to 65)
- Female engineers = 3z=97.2 (round to 97)
Step 3: Analyze College C
1. Total number of females (D + E):
- Total females = 550
- Female engineers = Female doctors + 70
- Let female doctors = a, then female engineers = a + 70:
a+(a+70)=550⟹2a+70=550⟹2a=480⟹a=240
- Female doctors = 240, Female engineers = 310
2. Total males (D + E):
- Total males = 760
3. Male engineers:
- Male engineers = 1.5 times female doctors = 1.5×240=360
4. Calculate male doctors:
- Male doctors = Total males - Male engineers = 760−360=400
Step 4: Calculate Total Engineers
1. Total engineers in College A:
- Male engineers = 200, Female engineers = 170
- Total engineers in A = 200+170=370
2. Total engineers in College B:
- Male engineers = 138, Female engineers = 97
- Total engineers in B = 138+97=235
3. Total engineers in College C:
- Male engineers = 360, Female engineers = 310
- Total engineers in C = 360+310=670
4. Total engineers in all colleges:
Total engineers=370+235+670=1275
Final Answer
The total number of engineers (male + female) in colleges A, B, and C together is 1275.
1. Given Ratios and Differences:
- Ratio of male doctors (D) to male engineers (E) = 12:5
- Difference between male doctors and male engineers = 280
12x−5x=280⟹7x=280⟹x=40
- Male doctors = 12x=12×40=480
- Male engineers = 5x=5×40=200
- Total females = 300
- Male engineers = 200, so:
Female engineers=Male engineers−30=200−30=170
- Female doctors = Total females - Female engineers = 300−170=130
1. Total number of males (D + E) in College B:
- Total males = Total females in College A = 300
- Ratio of males to females = 6:7
- Let males = 6y and females = 7y:
6y+7y=300⟹13y=300⟹y=30013≈23.08
- Males = 6y=6×23.08≈138.46 (round to 138)
- Females = 7y=7×23.08≈161.54 (round to 162)
- Ratio = 2:3
- Let female doctors = 2z and female engineers = 3z:
2z+3z=162⟹5z=162⟹z=1625=32.4
- Female doctors = 2z=64.8 (round to 65)
- Female engineers = 3z=97.2 (round to 97)
1. Total number of females (D + E):
- Total females = 550
- Female engineers = Female doctors + 70
- Let female doctors = a, then female engineers = a + 70:
a+(a+70)=550⟹2a+70=550⟹2a=480⟹a=240
- Female doctors = 240, Female engineers = 310
- Total males = 760
- Male engineers = 1.5 times female doctors = 1.5×240=360
- Male doctors = Total males - Male engineers = 760−360=400
1. Total engineers in College A:
- Male engineers = 200, Female engineers = 170
- Total engineers in A = 200+170=370
- Male engineers = 138, Female engineers = 97
- Total engineers in B = 138+97=235
- Male engineers = 360, Female engineers = 310
- Total engineers in C = 360+310=670
Total engineers=370+235+670=1275
The total number of engineers (male + female) in colleges A, B, and C together is 1275.
Similar Questions
Read the following information carefully and answer the questions given below. Find the average of the number of female in all the colleges except college C and E?
View SolutionRead the following information carefully and answer the questions given below. Find the average difference between the number of male and female students in all the colleges?
View SolutionRead the following information carefully and answer the questions given below. The number of female students in college C is what approx. percent of the number of male students of college A?
View SolutionRead the following information carefully and answer the questions given below. Find the ratio of 2/3rd of college B male students and female students of college F.
View Solutionनिम्न बार ग्राफ में A, B, C, D और E विभिन्न संगठनों में पुरुष और महिला सदस्यों की संख्या दी गई है।
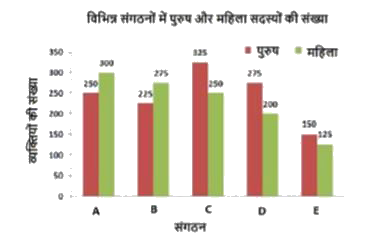
सभी पांच संगठनों में महिलाओं की औसत संख्या और सभी पांच संगठनों में पुरुषों की औसत संख्या का अनुपात ज्ञात करें।
View Solutionदिया गया दंड आरेख पांच संस्थानों A, B, C, D और E में पुरुषों और महिलाओं की संख्या को दर्शाता है।
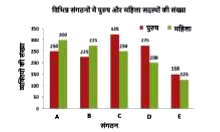
किस संस्थान में पुरुषों की संख्या, और सभी संस्थानों में महिलाओं की औसत संख्या के बीच अंतर न्यूनतम है?
View Solutionदिया गया दंड आरेख A, B, C, D और E पांच संगठनों में पुरुषो और महिलाओं की संख्या को दर्शाता है।
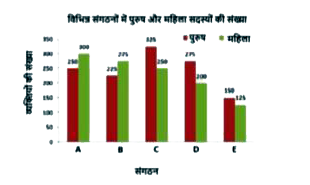
संगठनों C, D, और E में कार्यरत पुरुषो की कुल संख्या और संगठनों A, B और C में कार्यरत महिलाओं की कुल संख्या के बीच अनुपात ज्ञात करे।
View SolutionStudy the following information carefully and answer the given questions.
A, B, C, D, E, F and G are seven friends studying seven different branches of engineering, namely Mechanical, Chemical, Electrical, Electronics, Civil, Computer and Aeronautical Engineering, not necessarily in this order. Each of them studies in three different colleges, X, Y and Z. Not less than two study in any college. D studies Electrical Engineering in College X. The one who studies Chemical Engineering does not study in college Z. F studies Aeronautical Engineering in college Y with only B. A does not study in college X and does not study Civil Engineering. E studies Computer Engineering and does not study in college X. G studies Electronics Engineering but not in college X. None in college X studies Mechanical or Civil Engineering.
Which of the following groups represents the persons studying in college Z?
View Solution
