Study the following information carefully and answer the given questions.
The given data is regarding the number of doctors (D) and engineers (E) in Colleges A, B and C.
College A: Respective ratio between male doctors and male engineers is 12 : 5 and the difference between them, is 280. Total number of females (D+E) is 300. Number of male engineers is 30 more than the number of female engineers.
College B: Total number of males (D+E)=total number of females in college A (D + E). Respective ratio between total number of males (D+E) and total number of females (D +E) is 6:7 in College B. Respective ratio between the number of female doctors and the number of female engineers is 2:3. The number of male, doctors is one-third of the number of male engineers in College C.
College C: Number of male engineers is 1.5 times the number of female doctors. Total number of females (D +E) is 550. The number of female engineers is 70 more than the number of female doctors. Total number of males (D + E) is 760.
In another college D the number of females is 640 out of which 25% are doctors and the number of male doctors in college is 12.5% more than that in college C. What is the total number of doctors in college D?
630
610
570
None of those given as options
The correct Answer is:B
To solve the problem step by step, we will analyze the information provided for each college and derive the necessary values.
Step 1: Analyze College A
1. Male Doctors (MD) and Male Engineers (ME) Ratio: Given as 12:5. Let MD = 12x and ME = 5x.
2. Difference between Male Doctors and Male Engineers:
MD−ME=280⟹12x−5x=280⟹7x=280⟹x=40
- Therefore, MD=12x=480 and ME=5x=200.
3. Total number of females (D + E): Given as 300.
4. Number of Female Engineers (FE) and Female Doctors (FD):
- Let FD+FE=300.
- Male Engineers are 30 more than Female Engineers: ME=FE+30⟹200=FE+30⟹FE=170.
- Therefore, FD=300−170=130.
Step 2: Analyze College B
1. Total number of males (D + E): Equals total number of females in College A, which is 300.
2. Ratio of males to females in College B: Given as 6:7.
- Let total males = 6y and total females = 7y.
- From the above, 6y=300⟹y=50.
- Therefore, total males = 300 and total females = 350.
3. Female Doctors (FD) and Female Engineers (FE) Ratio: Given as 2:3.
- Let FD=2z and FE=3z.
- Therefore, 2z+3z=350⟹5z=350⟹z=70.
- Hence, FD=140 and FE=210.
Step 3: Analyze College C
1. Total number of females (D + E): Given as 550.
2. Number of Female Engineers (FE) and Female Doctors (FD):
- Given that FE=FD+70.
- Let FD=a, then FE=a+70.
- Therefore, a+(a+70)=550⟹2a+70=550⟹2a=480⟹a=240.
- Hence, FD=240 and FE=310.
3. Total number of males (D + E): Given as 760.
4. Male Engineers (ME): Given as 1.5 times the number of Female Doctors:
ME=1.5×240=360.
5. Male Doctors (MD):
MD+ME=760⟹MD+360=760⟹MD=400.
Step 4: Analyze College D
1. Number of females: Given as 640, and 25% are doctors.
Number of Female Doctors=25% of 640=0.25×640=160.
2. Number of Male Doctors in College D: Given as 12.5% more than in College C.
MDD=MDC+12.5% of MDC=400+0.125×400=400+50=450.
Step 5: Total Number of Doctors in College D
1. Total Doctors in College D:
Total Doctors=MDD+FDD=450+160=610.
Final Answer
The total number of doctors in College D is 610.
1. Male Doctors (MD) and Male Engineers (ME) Ratio: Given as 12:5. Let MD = 12x and ME = 5x.
2. Difference between Male Doctors and Male Engineers:
MD−ME=280⟹12x−5x=280⟹7x=280⟹x=40
- Therefore, MD=12x=480 and ME=5x=200.
3. Total number of females (D + E): Given as 300.
4. Number of Female Engineers (FE) and Female Doctors (FD):
- Let FD+FE=300.
- Male Engineers are 30 more than Female Engineers: ME=FE+30⟹200=FE+30⟹FE=170.
- Therefore, FD=300−170=130.
1. Total number of males (D + E): Equals total number of females in College A, which is 300.
2. Ratio of males to females in College B: Given as 6:7.
- Let total males = 6y and total females = 7y.
- From the above, 6y=300⟹y=50.
- Therefore, total males = 300 and total females = 350.
3. Female Doctors (FD) and Female Engineers (FE) Ratio: Given as 2:3.
- Let FD=2z and FE=3z.
- Therefore, 2z+3z=350⟹5z=350⟹z=70.
- Hence, FD=140 and FE=210.
1. Total number of females (D + E): Given as 550.
2. Number of Female Engineers (FE) and Female Doctors (FD):
- Given that FE=FD+70.
- Let FD=a, then FE=a+70.
- Therefore, a+(a+70)=550⟹2a+70=550⟹2a=480⟹a=240.
- Hence, FD=240 and FE=310.
3. Total number of males (D + E): Given as 760.
4. Male Engineers (ME): Given as 1.5 times the number of Female Doctors:
ME=1.5×240=360.
5. Male Doctors (MD):
MD+ME=760⟹MD+360=760⟹MD=400.
1. Number of females: Given as 640, and 25% are doctors.
Number of Female Doctors=25% of 640=0.25×640=160.
2. Number of Male Doctors in College D: Given as 12.5% more than in College C.
MDD=MDC+12.5% of MDC=400+0.125×400=400+50=450.
1. Total Doctors in College D:
Total Doctors=MDD+FDD=450+160=610.
The total number of doctors in College D is 610.
Similar Questions
Read the following information carefully and answer the questions given below. Find the average of the number of female in all the colleges except college C and E?
View SolutionRead the following information carefully and answer the questions given below. Find the average difference between the number of male and female students in all the colleges?
View SolutionRead the following information carefully and answer the questions given below. The number of female students in college C is what approx. percent of the number of male students of college A?
View SolutionRead the following information carefully and answer the questions given below. Find the ratio of 2/3rd of college B male students and female students of college F.
View Solutionनिम्न बार ग्राफ में A, B, C, D और E विभिन्न संगठनों में पुरुष और महिला सदस्यों की संख्या दी गई है।
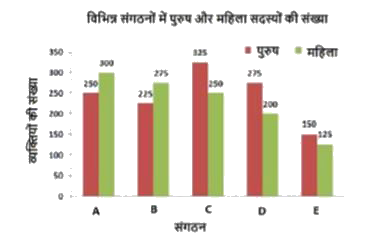
सभी पांच संगठनों में महिलाओं की औसत संख्या और सभी पांच संगठनों में पुरुषों की औसत संख्या का अनुपात ज्ञात करें।
View Solutionदिया गया दंड आरेख पांच संस्थानों A, B, C, D और E में पुरुषों और महिलाओं की संख्या को दर्शाता है।
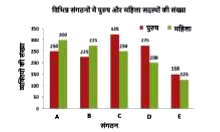
किस संस्थान में पुरुषों की संख्या, और सभी संस्थानों में महिलाओं की औसत संख्या के बीच अंतर न्यूनतम है?
View Solutionदिया गया दंड आरेख A, B, C, D और E पांच संगठनों में पुरुषो और महिलाओं की संख्या को दर्शाता है।
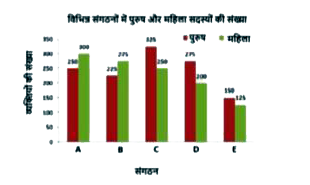
संगठनों C, D, और E में कार्यरत पुरुषो की कुल संख्या और संगठनों A, B और C में कार्यरत महिलाओं की कुल संख्या के बीच अनुपात ज्ञात करे।
View SolutionStudy the following information carefully and answer the given questions.
A, B, C, D, E, F and G are seven friends studying seven different branches of engineering, namely Mechanical, Chemical, Electrical, Electronics, Civil, Computer and Aeronautical Engineering, not necessarily in this order. Each of them studies in three different colleges, X, Y and Z. Not less than two study in any college. D studies Electrical Engineering in College X. The one who studies Chemical Engineering does not study in college Z. F studies Aeronautical Engineering in college Y with only B. A does not study in college X and does not study Civil Engineering. E studies Computer Engineering and does not study in college X. G studies Electronics Engineering but not in college X. None in college X studies Mechanical or Civil Engineering.
Which of the following groups represents the persons studying in college Z?
View Solution
