From the options given below, find out the correct floral formula for a flower having the following characters namely actinomorphic, bisxual, five united sepals, five united petals, stamens five and epipetalous, bicarpellary syncarpous with superior ovary
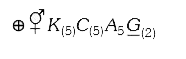
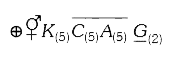
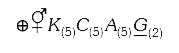
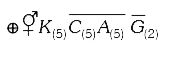
The correct Answer is:B
Flower is bisexual, actinomorphic, sepals are five, and petals are five, bicarpellary.
Similar Questions
Write floral formula for a flower which is bisexual, actinomorphic sepals five, twisted aestivation, petals five valvate aestivation, stamens six, ovary tricarpellary, syncarpous , superior, trilocular with axile placentation.
View SolutionWrite the floral formula of an actinomorphic bisexual, hypogynous flower with five united sepals, five free petals. Five free stamens and two united carpals with superior ovary and axile placentation.
View Solution
Knowledge Check
Assertion:The floral formula of Family Solanaceae is
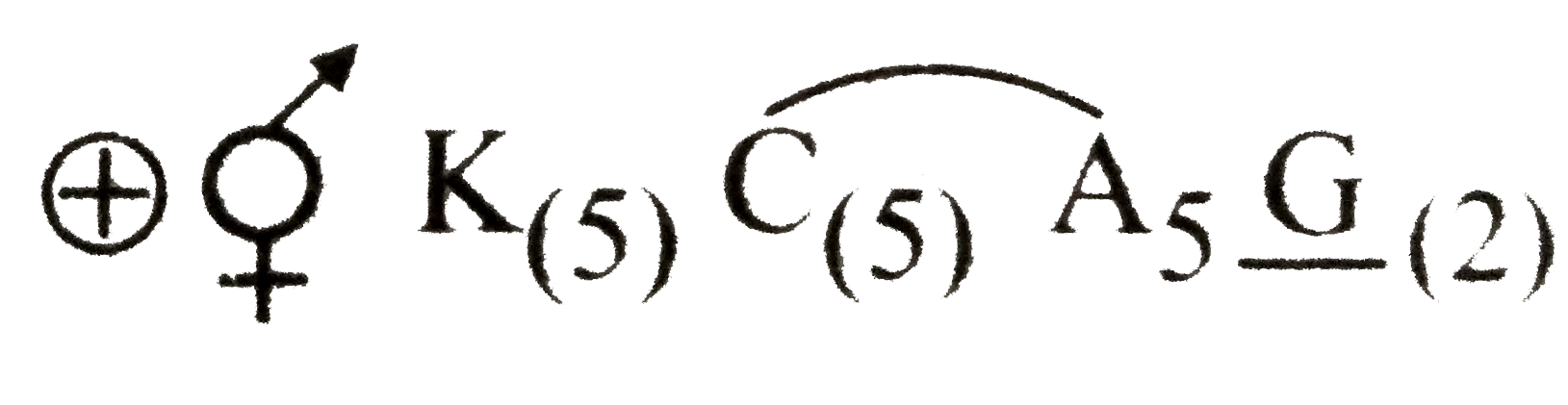
Reason:This floral formula of Solanaceae tells that flower is bisexual, sepals five, petals five, stamens five and gynoecium tricarpellary, trilocular with many ovules.AIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true but reason is not the correct explanation of assertion.CIf assertion is true but reason is false.DIf assertion and reason are false.Read the following statements and find out the incorrect statement.
a. Mustard has hypogynous, actinomorphic flower, parietal placentation, syncarpous gynoecium and belongs to family Brassicaceae
b. China rose has superior ovary, twisted aestivation, monodephous stamens and axile placentation
c. Pea has bilateral symmetry, vexillary aestivation, diadelphous stamens, marginal placentation and belongs to family fabaceae
d. Chilli has radial symmetry, epipetalous stamen, swollen placenta, monocarpellary gynoecium and belongs to family solanaceae
e. Lily has actinomorphic flower, axile placentation, imbricate aestivation, tricarpellary and trilocular gynoeciom belonging to family liliaceae.A3B1C4D2A man is facing towards the east. He turns towards north and walks for 5 km, and then turns 270 degrees anticlockwise and walks for 12 km more. What is the minimum distance between his initial and final position?
A(a) 17 kmB(b) 13 kmC(c) 11 kmD(d) 9 km
Select the most appropriate option, out of the five options given for each of the following sentences, which in your view, should be grammatically and structurally correct. Please note that the meaning and the context of the sentence must not change.
View SolutionSelect the most appropriate option, out of the five options given for each of the following sentences, which in vour view, should be grammatically and structurally correct. Please note that the meaning and the context of the sentence must not change.
View SolutionIn given question below consists of five or six statement followed by options consisting of three statements put together in a specific order. Choose the option which indicates a valid argument containing logically related statements that is, where the third statement is a conclusion drawn from the preceding two statement
A : Six is five.
B : Five is not four.
C : Some five is ten.
D : Some six is twelve.
E : Some twelve is five.
F : Some ten is four.
View SolutionRead the following statements and find the suitable option
a. Generally petals are green, leaf like and protect the flower in the bud stage
b. Sepals are brightly coloured to attract insects for pollination
c. If stamens in a flower remain free this is called polyandrous
d. Each ovary bears one or more ovules attached to a flattened, cushion like placenta
e. There may be a variation in the length of filaments of stamen with on flower, as in Salvinia and mustard.
View SolutionIn the following question, the sentence given with a blank to be filled in with an appropriate word. Select the correct alternative out of the four and indicate it by selecting the appropriate option.
………….of the five persons will appear in the court.
View Solution