Inside a cylinder having insulating walls and dosed at ends is a movable piston, which divides the cylinder into two compartments. On one side of the piston is a mass m of a gas and on the other side a mass 2 m of the same gas. What fraction of volume of the cylinder will be occupied by the Larger mass of the gas when the piston is in equilibrium Consider that the movable piston is conducting so that the temperature is the same throughout
14
13
12
23
The correct Answer is:D
To solve the problem, we need to analyze the situation using the principles of the kinetic theory of gases and the ideal gas law.
1. Understanding the Setup: We have a cylinder divided into two compartments by a movable piston. On one side, there is a mass m of gas, and on the other side, there is a mass 2m of the same gas. The walls of the cylinder are insulating, and the piston is conducting, meaning the temperature is uniform throughout.
2. Using the Ideal Gas Law: The ideal gas law states that PV=nRT, where:
- P is the pressure,
- V is the volume,
- n is the number of moles of gas,
- R is the universal gas constant,
- T is the temperature.
3. Defining Variables: Let:
- V1 be the volume occupied by mass m,
- V2 be the volume occupied by mass 2m.
4. Pressure Equilibrium: Since the piston is in equilibrium, the pressure on both sides must be equal:
P1=P2
5. Applying the Ideal Gas Law: For the gas with mass m:
PV1=n1RT
For the gas with mass 2m:
PV2=n2RT
Here, n1 and n2 are the number of moles of gas on each side. Since the gas is the same, we can relate the number of moles to the mass:
n1=mMandn2=2mM
where M is the molar mass of the gas.
6. Setting Up the Equations: Substituting n1 and n2 into the ideal gas equations:
PV1=mMRT(1)
PV2=2mMRT(2)
7. Dividing the Equations: Since P and RT are the same for both sides, we can divide equation (1) by equation (2):
V1V2=m2m=12
8. Expressing Volumes: This means:
V1=12V2
9. Total Volume: The total volume V of the cylinder is:
V=V1+V2=12V2+V2=32V2
10. Finding the Fraction: We want to find the fraction of the total volume occupied by the larger mass of gas (which occupies volume V2):
Fraction occupied by 2m=V2V=V232V2=23
Thus, the fraction of the volume of the cylinder occupied by the larger mass of gas is 23.
Final Answer:
The fraction of volume occupied by the larger mass of gas is 23.
- P is the pressure,
- V is the volume,
- n is the number of moles of gas,
- R is the universal gas constant,
- T is the temperature.
- V1 be the volume occupied by mass m,
- V2 be the volume occupied by mass 2m.
P1=P2
PV1=n1RT
For the gas with mass 2m:
PV2=n2RT
Here, n1 and n2 are the number of moles of gas on each side. Since the gas is the same, we can relate the number of moles to the mass:
n1=mMandn2=2mM
where M is the molar mass of the gas.
PV1=mMRT(1)
PV2=2mMRT(2)
V1V2=m2m=12
V1=12V2
V=V1+V2=12V2+V2=32V2
Fraction occupied by 2m=V2V=V232V2=23
The fraction of volume occupied by the larger mass of gas is 23.
Topper's Solved these Questions
- KINETIC THEORY OF GASES BOOK - ERRORLESSCHAPTER - KINETIC THEORY OF GASES EXERCISE - NCERT BASED QUESTIONS (Speed of Gas)
24 Videos
- KINETIC THEORY OF GASES BOOK - ERRORLESSCHAPTER - KINETIC THEORY OF GASES EXERCISE - NCERT BASED QUESTIONS (Degree of Freedom and Specific Heat)
30 Videos
- GRAVITATIONBOOK - ERRORLESSCHAPTER - GRAVITATIONEXERCISE - Assertion and Reason
25 Videos
- MOTION IN ONE DIMENSIONBOOK - ERRORLESSCHAPTER - MOTION IN ONE DIMENSIONEXERCISE - ASSERTION AND REASON
24 Videos
Similar Questions
Knowledge Check
A gas is filled in the cylinder shown in fig. The two pistons are joined by a string. If the gas is heated, the right piston will
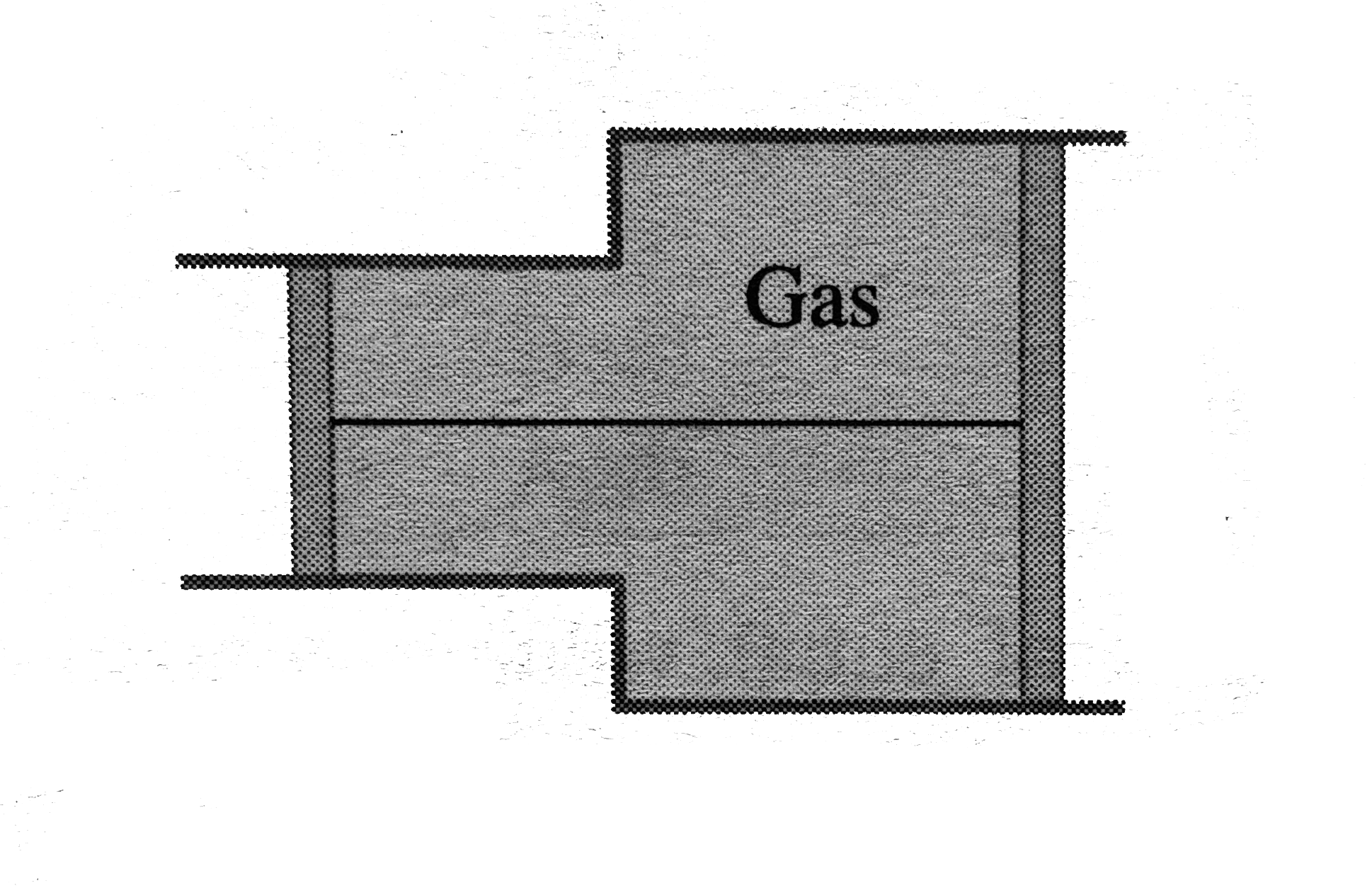 Amove toward leftBmove toward rightCremain stationaryDnone of these
Amove toward leftBmove toward rightCremain stationaryDnone of these10dm3 of hydrogen under 1 bar pressure are contained in a cylinder which has a movable piston. The piston is moved in until the same mass of gas occupies 2dm3 at the same temperature. What will be the pressure in the cylinder ?
A5 barB6 barC7 barDNone of theseA closed and isolated cylinder contains ideal gas. An adiabatic separator of mass m, cross sectional area A divides the cylinder into two equal parts each with volume V0 and pressure P0 in equilibrium Assume the sepatator to move without friction.
If the piston is slightly displaced by x, the net force acting on the piston isAP0γA2xV0B2P0γA2xV0C3P0γA2xV0DP0γA2x(γ−1)V0
Ten dm3 of hydrogen under 1 bar pressure are contained in a cylinder which has a movable piston. The piston is moved in until the same mass of gas occupies 2dm3 at the same temperature. Find the pressure in the cylinder.
View SolutionA gas is filled in a cylinder fitted with a piston at a definite temperature and pressure. Explain on the basis of kinetic theory why on pulling the piston out, the pressure of decreases.
View SolutionA vertical cylinder of cross-sectional area 0.1m2 closed at both ends is fitted with a frictionless piston of mass M dividing the cylinder into two parts. Each part contains one mole of an ideal gas in equilibrium at 300K. The volume of the upper part is 0.1m3 and that of lower part is 0.05m3 .What force must be applied to the piston so that the volumes of the two parts remain unchanged when the temperature is increased to 500K?
View SolutionThe pressure of a gas contained in a cylinder with a movable piston is 300 Pa. The area of the piston is 0.5m2. Calculate the force that is exerted on the piston.
View SolutionA cylinder containing an ideal gas is in vertical position and has a piston of mass M that is able to move up or down without friction (figure). If the temperature is increased
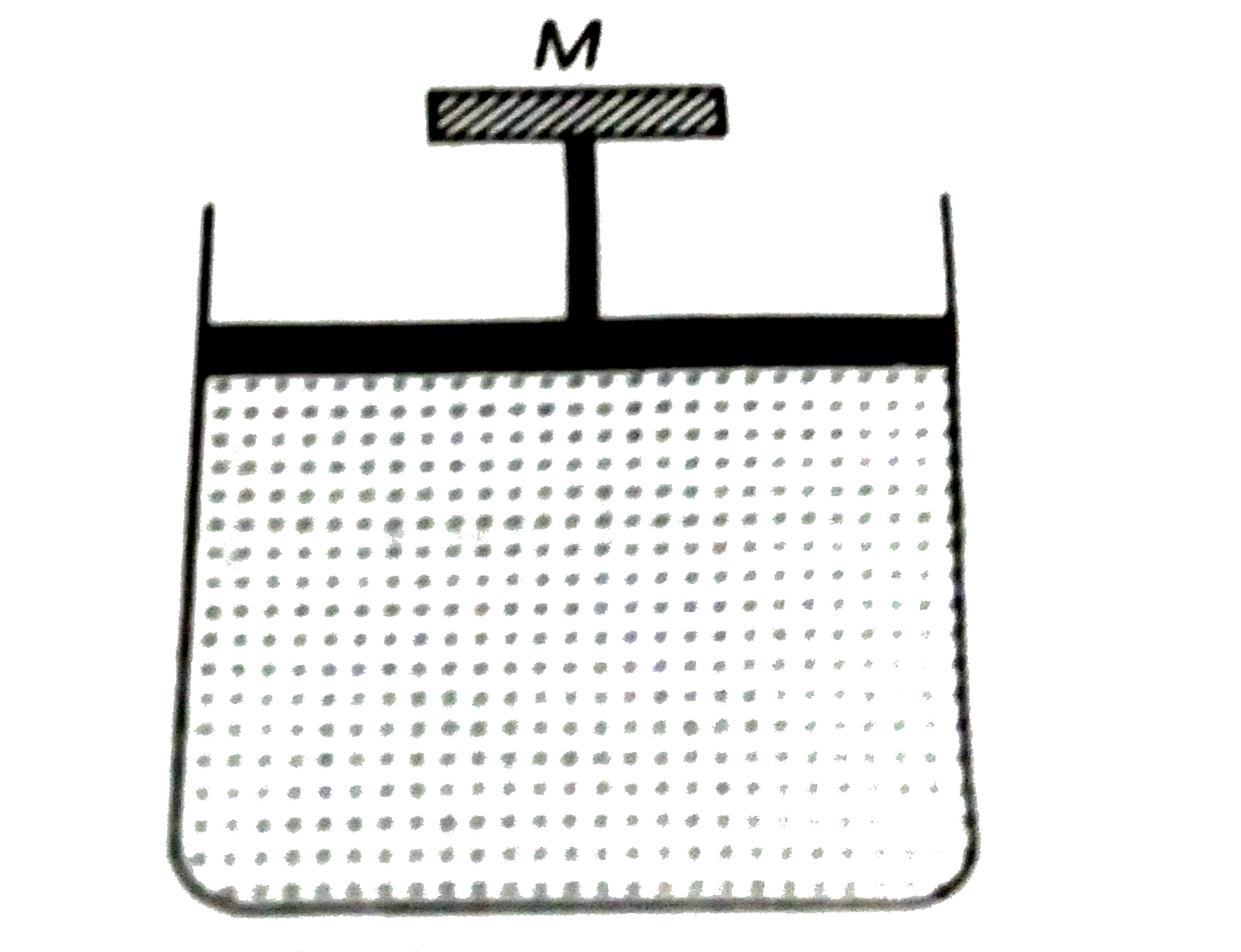
View Solution
