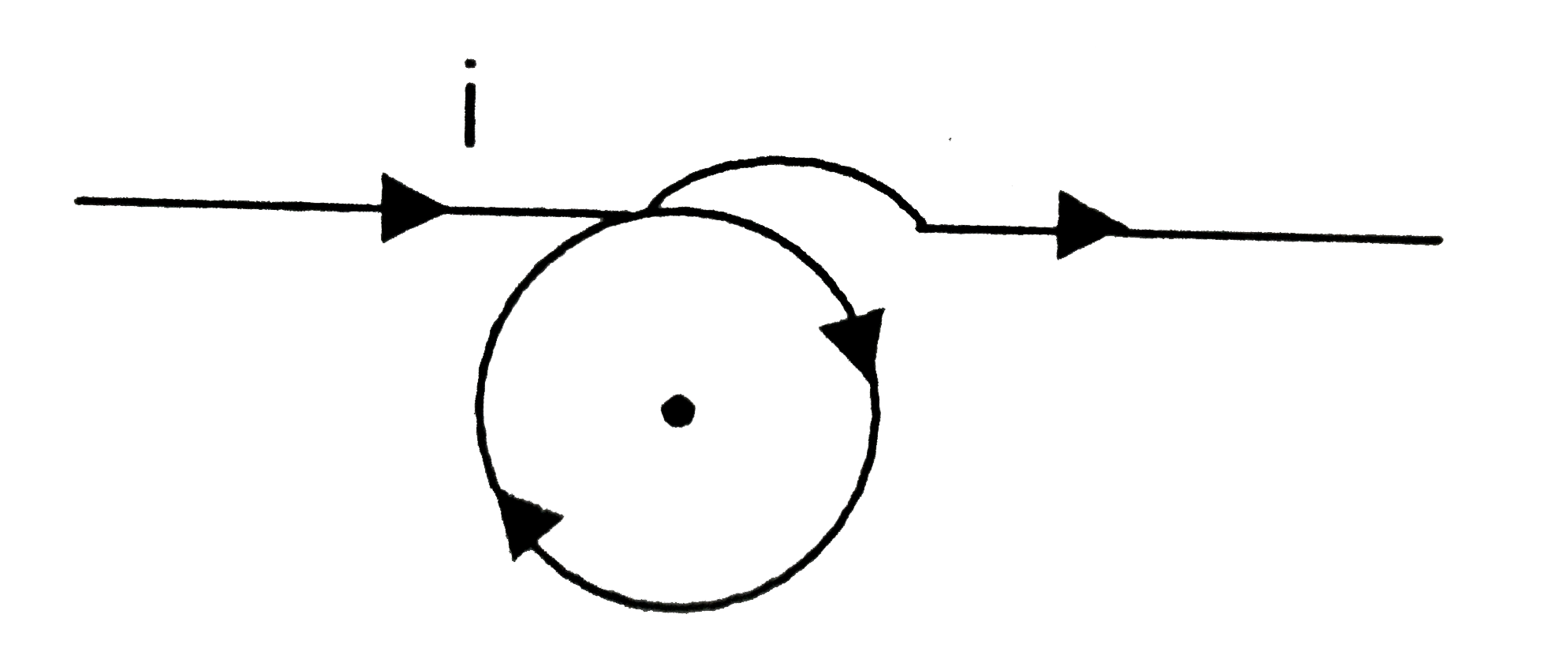
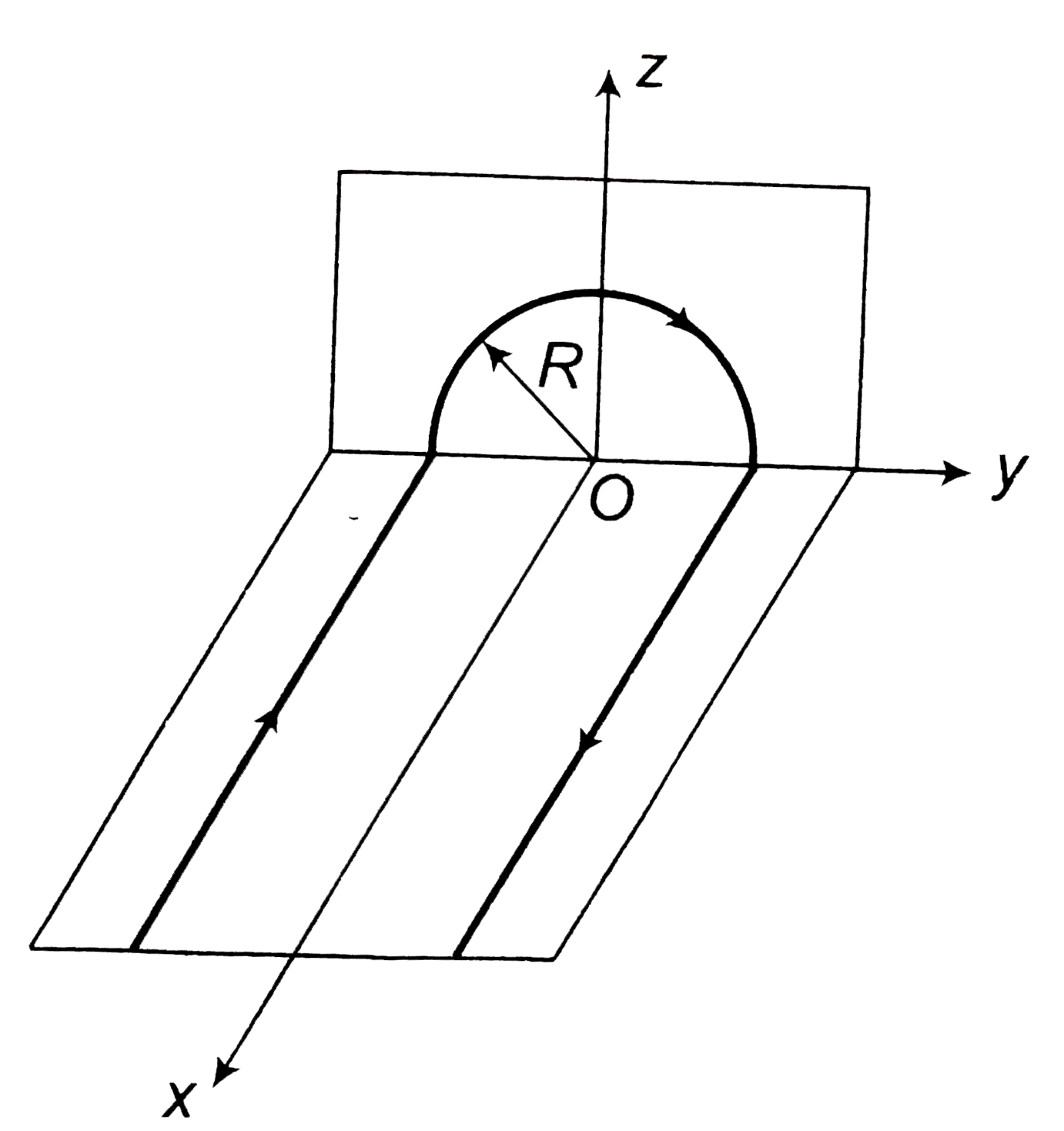
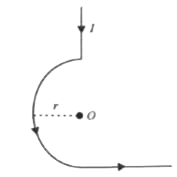
In the given figure net magnetic field at O will be
A
2μ0i3πa√4−π2
B
μ0i3πa√4+π2
C
2μ0i3πa2√4+π2
D
μ0i3πa√4−π2
Video Solution
Text Solution
The correct Answer is:B
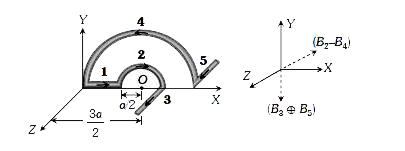
Inner loop ,
B1=μ04π(πi)(a/2)⊗ (-ve z-axis)
Outer loop
B2=μ04π(πi3a/2)⊙ (+ ve z-axis)
Along z-axis
B1−B2=μ04π⋅πia[2−23]⊗
Wire-inner ,
B3=μ04π⋅i(a/2) (-ve y-axis)
Outer ,
B4=μ04π(i3a/2) (-ve y-axis)
B3+B4=μ04π.Ia(2+23) (-ve y-axis)
Bnet=√(B1+B2)2+(B3+B4)2=μ0i3πa√π2+4
|
Topper's Solved these Questions
- MAGNETIC EFFECTS OF CURRENT BOOK - ERRORLESSCHAPTER - MAGNETIC EFFECTS OF CURRENT EXERCISE - NCERT BASED QUESTIONS (Motion of Charged Particle in Magnetic Field)
69 Videos
- MAGNETIC EFFECTS OF CURRENT BOOK - ERRORLESSCHAPTER - MAGNETIC EFFECTS OF CURRENT EXERCISE - NCERT BASED QUESTIONS (Force and Torque on a Current Carrying Conductor )
51 Videos
- ELECTROSTATICSBOOK - ERRORLESSCHAPTER - ELECTROSTATICSEXERCISE - ASSERTION & REASON
25 Videos
- MAGNETISMBOOK - ERRORLESSCHAPTER - MAGNETISMEXERCISE - Assertion and Reason
22 Videos
Similar Questions
Knowledge Check
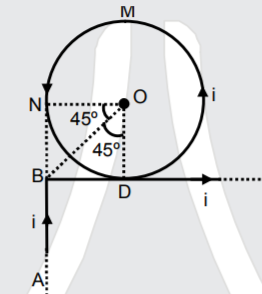
The magnetic field at O is
 Aμ0I2R√(14+1π2)Bμ0I2R√(12+1π2)Cμ0IR√(14+1π2)Dμ0IR√(14+1π)
Aμ0I2R√(14+1π2)Bμ0I2R√(12+1π2)Cμ0IR√(14+1π2)Dμ0IR√(14+1π)The magnetic field at O is
 AzeroB1C2D3
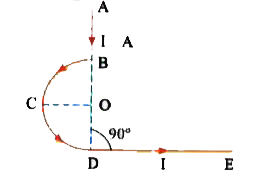
AzeroB1C2D3Find magnetic field at O.
 Aμ0i2πR[−1√2+π]Bμ0i2πR[π−1]Cμ0i2RDμ0i2πR[1√2+π]
Aμ0i2πR[−1√2+π]Bμ0i2πR[π−1]Cμ0i2RDμ0i2πR[1√2+π]
In the given figure the magnitude of magnetic field at O is ( all three wires are quarter circular ares )

View SolutionIn the given figure the magnetic induction at the point O is
View SolutionA current I flows through a long straight conductor which is bent into a circular loop of radius R in the middle as shown in the figure .
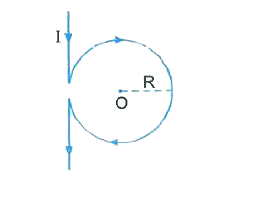
The magnitude of the net magnetic field at point O will be
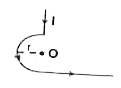
View SolutionIn the given figure what is the magnetic field induction at point O?
View SolutionIn the given figure what is the magnetic field induction at point O?
View Solution