Three identical points charges, as shown are placed at the vertices of an isosceles right angled triangle. Which of the nembered vectors coincides in direction with the electric field at the mid-point the hypotenuse
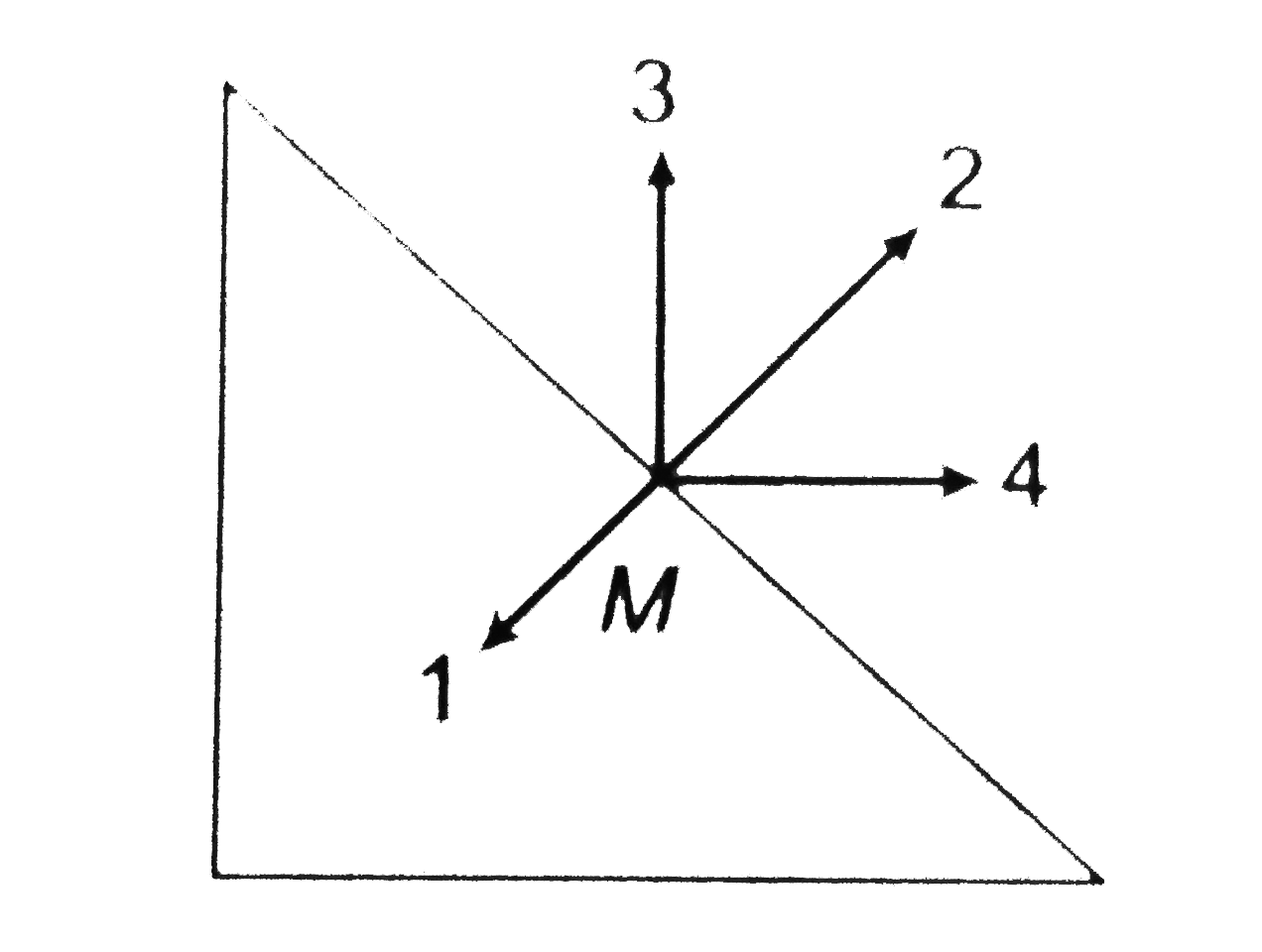
1
2
3
4
The correct Answer is:A, B
If all charges are +ve. If all charges are –ve
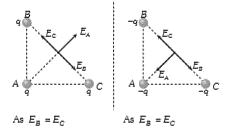
As As
Topper's Solved these Questions
- ELECTROSTATICSBOOK - ERRORLESSCHAPTER - ELECTROSTATICSEXERCISE - NCERT BASED QUESTIONS (Electric Dipole)
21 Videos
- ELECTROSTATICSBOOK - ERRORLESSCHAPTER - ELECTROSTATICSEXERCISE - NCERT BASED QUESTIONS (Electric Flux and Gauss.s Law)
27 Videos
- ELECTROSTATICSBOOK - ERRORLESSCHAPTER - ELECTROSTATICSEXERCISE - ASSERTION & REASON
25 Videos
- ELECTRONICSBOOK - ERRORLESSCHAPTER - ELECTRONICSEXERCISE - Assertion & Reason
26 Videos
- MAGNETIC EFFECTS OF CURRENT BOOK - ERRORLESSCHAPTER - MAGNETIC EFFECTS OF CURRENT EXERCISE - ASSERTION & REASON
18 Videos
Similar Questions
Knowledge Check
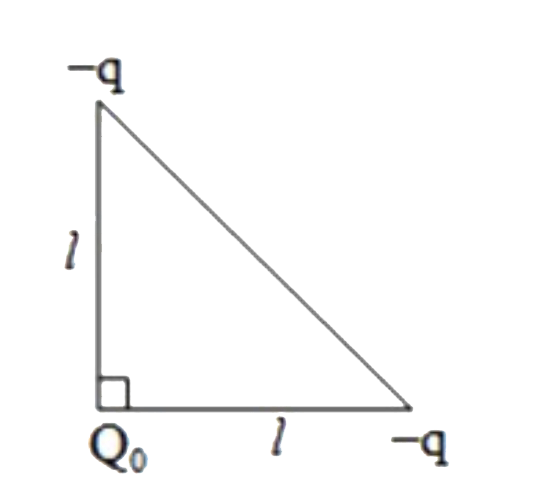
Three charges Q0,−q and −q are placed at the vertices of an isosceles right angle triangle as in the figure. The net electrostatic potential energy is zero if Q 0 is equal to :
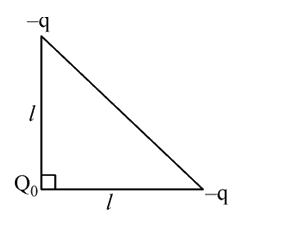 Aq4Bq2√2C√2qD+q
Aq4Bq2√2C√2qD+q
Show that the points (2,-1,3), (0,1,2) and (1,-3,1) are the vertices of an isosceles right angled triangle.
View SolutionShow that the points (0,7,10), (-1,6,6) and(-4,9,6) are the vertices of an isosceles right angled triangle.
View SolutionShow that the points A(7, 10), B(-2, 5) and C(3, -4) are the vertices of an isosceles right-angled triangle.
View SolutionAs shown in the figure, charges and are placed at the vertices and of an isosceles triangle. The potential at the vertex A is
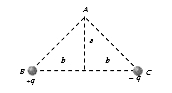
View SolutionShow that the points A(2,-1,3),B(1,-3,1) and C(0,1,2) are the vertices of an isosceles right angled triangle.
View SolutionThree charges Q0 - q and q are placed at the vertices of an isosceles right angle triangle as in the future. The net electrostatic potential energy is zero if Q0 is equal to
View SolutionShow that the points (0, 7, 10), (-1, 6, 6) and (-4, 9, 6) are the vertices of an isosceles right
angled triangle.
View Solution
